ES
3
K
Khách
Hãy nhập câu hỏi của bạn vào đây, nếu là tài khoản VIP, bạn sẽ được ưu tiên trả lời.
Các câu hỏi dưới đây có thể giống với câu hỏi trên
KV
26 tháng 12 2022
a) A = 2⁰ + 2¹ + 2² + 2³ + ... + 2²⁰²²
2A = 2 + 2² + 2³ + 2⁴ + ... + 2²⁰²³
A = 2A - A
= (2 + 2² + 2³ + 2⁴ + ... + 2²⁰²³) - (2⁰ + 2¹ + 2² + 2³ + ... + 2²⁰²²)
= 2²⁰²³ - 2⁰
= 2²⁰²³ - 1
Vậy A = B
b) A = 2021 . 2023
= (2022 - 1).(2022 + 1)
= 2022.(2022 + 1) - 2022 - 1
= 2022² + 2022 - 2022 - 1
= 2022² - 1 < 2022²
Vậy A < B
NC
2
29 tháng 7 2023
a) \(S=1+2+2^2+2^3+...+2^{2022}=\dfrac{2^{2022+1}-1}{2-1}=2^{2023}-1\)
b) \(S=1+4+4^2+4^3+...+4^{2022}=\dfrac{4^{2022+1}-1}{4-1}=\dfrac{4^{2023}-1}{3}\)
29 tháng 7 2023
\(S=1+2+2^2+2^3+...+2^{2022}\\ 2S=2+2^2+2^3+2^4+...+2^{2023}\\ 2S-S=2+2^2+2^3+2^4+...+2^{2023}-1-2-2^2-2^3-...-2^{2022}\\ S=2^{2023}-1\\ S=4+4^2+4^3+...+4^{2022}\\ 4S=4^2+4^3+4^4+...+4^{2023}\\ 4S-S=4^2+4^3+4^4+...+4^{2023}-4-4^2-4^3-...-4^{2023}\\ 3S=4^{2023}-4\\ S=\dfrac{4^{2023}-4}{3}\)
17 tháng 11 2021
\(a,\Rightarrow x+2=-40\\ \Rightarrow x=-42\\ b,\Rightarrow6x-7-2x=5\\ \Rightarrow4x=12\Rightarrow x=3\\ c,\Rightarrow68-56-x=-2\\ \Rightarrow12-x=-2\\ \Rightarrow x=14\)
AH
Akai Haruma
Giáo viên
31 tháng 12 2023
Câu 1:
$A=(2+2^2)+(2^3+2^4)+(2^5+2^6)+....+(2^{2019}+2^{2020})$
$=2(1+2)+2^3(1+2)+2^5(1+2)+....+2^{2019}(1+2)$
$=(1+2)(2+2^3+2^5+...+2^{2019})=3(2+2^3+2^5+...+2^{2019})\vdots 3$
-----------------
$A=2+(2^2+2^3+2^4)+(2^5+2^6+2^7)+....+(2^{2018}+2^{2019}+2^{2020})$
$=2+2^2(1+2+2^2)+2^5(1+2+2^2)+....+2^{2018}(1+2+2^2)$
$=2+(1+2+2^2)(2^2+2^5+....+2^{2018})$
$=2+7(2^2+2^5+...+2^{2018})$
$\Rightarrow A$ chia $7$ dư $2$.
AH
Akai Haruma
Giáo viên
31 tháng 12 2023
Câu 2:
$B=(3+3^2)+(3^3+3^4)+....+(3^{2021}+3^{2022})$
$=3(1+3)+3^3(1+3)+...+3^{2021}(1+3)$
$=(1+3)(3+3^3+...+3^{2021})=4(3+3^3+....+3^{2021})\vdots 4$
-------------------
$B=(3+3^2+3^3)+(3^4+3^5+3^6)+...+(3^{2020}+3^{2021}+3^{2022})$
$=3(1+3+3^2)+3^4(1+3+3^2)+....+3^{2020}(1+3+3^2)$
$=(1+3+3^2)(3+3^4+...+3^{2020})=13(3+3^4+...+3^{2020})\vdots 13$ (đpcm)
HT
1
30 tháng 10 2023
\(A=2+2^2+2^3+...+2^{2021}\)
=>\(2A=2^2+2^3+2^4+...+2^{2022}\)
=>\(2A-A=2^2+2^3+...+2^{2021}+2^{2022}-2-2^2-2^3-...-2^{2021}\)
=>\(A=2^{2022}-2\)
=>A<B
NH
1
NV
Nguyễn Việt Lâm
Giáo viên
28 tháng 12 2021
\(A=1+2+2^2+...+2^{2022}\)
\(\Rightarrow2A=2+2^2+...+2^{2023}\)
\(\Rightarrow2A-A=2^{2023}-1\)
\(\Rightarrow A=2^{2023}-1\)
\(\Rightarrow A< 2^{2023}=2^2.2^{2021}=4.2^{2021}< 5^{2021}\)
\(\Rightarrow A< B\)
CM
17 tháng 5 2017
a) 22 + (2x -13) = 83 => 2x -13 = 61 => x = 37.
b) 51 - (-12 + 3x) = 27 => 63 - 3x = 27 => x = 12.
c) - (2x + 2) + 21 = - 23 => 2x + 2 = 44 => x = 21.
d) 25 - (25 - x) = 0 => 25 - 25 + x = 0 => x = 0.
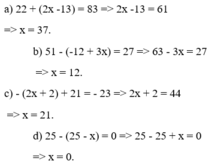
a) \(A=2+2^2+2^3+...+2^{2022}\)
\(2A=2.\left(2+2^2+2^3+...+2^{2022}\right)\)
\(2.A=2^2+2^3+2^4+...+2^{2023}\)
\(2A-A=\left(2^2+2^3+2^4+...+2^{2023}\right)-\left(2+2^2+2^3+...+2^{2022}\right)\)
\(A=2^{2023}-2\)
b) A + 2 = 2x
Hay \(\left(2^{2023}-2\right)+2=2^x\)
\(2^{2023}-2+2=2^x\)
\(2^{2023}=2^x\)
\(\Rightarrow x=2023\)
a, A = 21 + 22 + 23 + ...+ 22022
2A = 22 + 23 +...+ 22022 + 22023
2A - A = 22023 - 21
A = 22023 - 2
b, A + 2 = 2\(^x\) ⇒ 22023 - 2 + 2 = 2\(x\)
22023 = 2\(^x\)
2023 = \(x\)