17+(-20)+23+(-26)+...+53+(-56)
Hãy nhập câu hỏi của bạn vào đây, nếu là tài khoản VIP, bạn sẽ được ưu tiên trả lời.


Bài 2:
a. $x^2=12y^2+1$ lẻ nên $x$ lẻ
Ta biết một scp khi chia 8 dư $0,1,4$. Mà $x$ lẻ nên $x^2$ chia $8$ dư $1$
$\Rightarrow 12y^2+1\equiv 1\pmod 8$
$\Rightarrow 12y^2\equiv 0\pmod 8$
$\Rightarrow y^2\equiv 0\pmod 2$
$\Rightarrow y$ chẵn. Mà $y$ nguyên tố nên $y=2$.
Khi đó: $x^2=12y^2+1=12.2^2+1=49\Rightarrow x=7$ (tm)
Bài 2:
b.
$x^2=8y+1$ nên $x$ lẻ. Đặt $x=2k+1$ với $k$ tự nhiên.
Khi đó: $8y+1=x^2=(2k+1)^2=4k^2+4k+1$
$\Rightarrow 2y=k(k+1)$
Vì $(k,k+1)=1, k< k+1$ và $y$ nguyên tố nên xảy ra các TH sau:
TH1: $k=2, k+1=y\Rightarrow y=3\Rightarrow x=5$ (tm)
TH2: $k=1, k+1=2y\Rightarrow y=1$ (vô lý)
TH3: $k=y, k+1=2\Rightarrow y=1$ (vô lý)
Vậy $(x,y)=(5,3)$ là đáp án duy nhất thỏa mãn.

tìm x biết:
2x:(1+\(\dfrac{1}{1+2}\)\(+\dfrac{1}{1+2+3}\)\(+.....\)\(+\dfrac{1}{1+2+3+...+x}\))=2023

\(2x:\left(1+\dfrac{1}{1+2}+\dfrac{1}{1+2+3}+...+\dfrac{1}{1+2+3+...x}\right)=2023\left(1\right)\)
Đặt \(A=\left(1+\dfrac{1}{1+2}+\dfrac{1}{1+2+3}+...+\dfrac{1}{1+2+3+...x}\right)\)
\(\Rightarrow A=\left(1+\dfrac{1}{3}+\dfrac{1}{6}+...+\dfrac{1}{\dfrac{x\left(x+1\right)}{2}}\right)\)
\(\Rightarrow\dfrac{1}{2}A=\left(\dfrac{1}{2}+\dfrac{1}{2.3}+\dfrac{1}{3.4}+...+\dfrac{1}{x\left(x+1\right)}\right)\)
\(\Rightarrow\dfrac{1}{2}A=\left(1-\dfrac{1}{2}+\dfrac{1}{2}-\dfrac{1}{3}+\dfrac{1}{3}-\dfrac{1}{4}+...+\dfrac{1}{x}-\dfrac{1}{x+1}\right)\)
\(\Rightarrow\dfrac{1}{2}A=1-\dfrac{1}{x+1}\)
\(\Rightarrow A=2\left(1-\dfrac{1}{x+1}\right)\Rightarrow A=\dfrac{2x}{x+1}\)
\(\left(1\right)\Rightarrow2x:\dfrac{2x}{x+1}=2023\)
\(\Rightarrow2x.\dfrac{x+1}{2x}=2023\left(x\ne0\right)\)
\(\Rightarrow x+1=2023\)
\(\Rightarrow x=2022\)

Để olm.vn giúp em, em nhé.
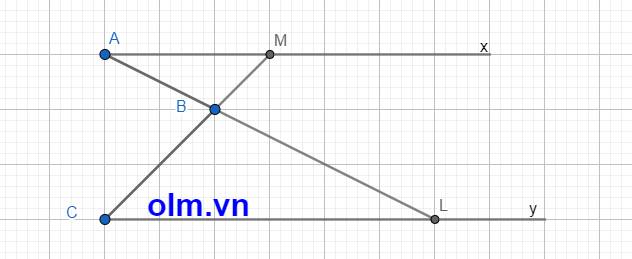
Kẻ AB kéo dài về phía B cắt đường thẳng y tại L
Kéo dài CB vế phía B cắt đường thẳng x tại M
Vì x//y ta có: \(\widehat{MAB}\) = \(\widehat{BLC}\) ( so le trong)
\(\widehat{ABC}\) = \(\widehat{BCL}\) + \(\widehat{BLC}\) (góc ngoài tam giác bằng tổng hai góc trong không kề với nó)
⇒ \(\widehat{ABC}\) = \(\widehat{BCL}\) + \(\widehat{MAB}\) (đpcm)

d, -(24 - 87) + (- 87 + 48 - 24)
= - 24 + 87 - 87 + 48 - 24
= - (24 + 24) + (87 - 87 ) + 48
= -48 + 0 + 48
= 0
e, (2027. 20262024 - 20262024) : 20262025
= 20262024.(2027 - 1):20262025
= 20262024.2026: 20262025
= 20262025:20262025
= 1

\(A=\dfrac{10}{7.12}+\dfrac{10}{12.17}+\dfrac{10}{17.22}+...+\dfrac{10}{502.507}\) (sửa 502+507 thành 503.507)
\(\Rightarrow A=10\left(\dfrac{1}{7.12}+\dfrac{1}{12.17}+\dfrac{1}{17.22}+...+\dfrac{1}{502.507}\right)\)
\(\Rightarrow A=10.\dfrac{1}{5}\left(\dfrac{1}{7}-\dfrac{1}{12}+\dfrac{1}{12}-\dfrac{1}{17}+\dfrac{1}{17}-\dfrac{1}{22}+...+\dfrac{1}{502}-\dfrac{1}{507}\right)\)
\(\Rightarrow A=2.\left(\dfrac{1}{7}-\dfrac{1}{507}\right)=2.\left(\dfrac{500}{3549}\right)=\dfrac{1000}{3549}\)
\(B=\dfrac{4}{8.13}+\dfrac{4}{13.18}+\dfrac{4}{18.23}+...+\dfrac{4}{253.258}\)
\(\Rightarrow B=4\left(\dfrac{1}{8.13}+\dfrac{1}{13.18}+\dfrac{1}{18.23}+...+\dfrac{1}{253.258}\right)\)
\(\Rightarrow B=4.\dfrac{1}{5}\left(\dfrac{1}{8}-\dfrac{1}{13}+\dfrac{1}{13}-\dfrac{1}{18}+\dfrac{1}{18}-\dfrac{1}{23}+...+\dfrac{1}{253}-\dfrac{1}{258}\right)\)
\(\Rightarrow B=\dfrac{4}{5}\left(\dfrac{1}{8}-\dfrac{1}{258}\right)=\dfrac{4}{5}\left(\dfrac{129}{1032}-\dfrac{8}{1032}\right)=\dfrac{4}{5}.\dfrac{121}{1032}=\dfrac{121}{1290}\)
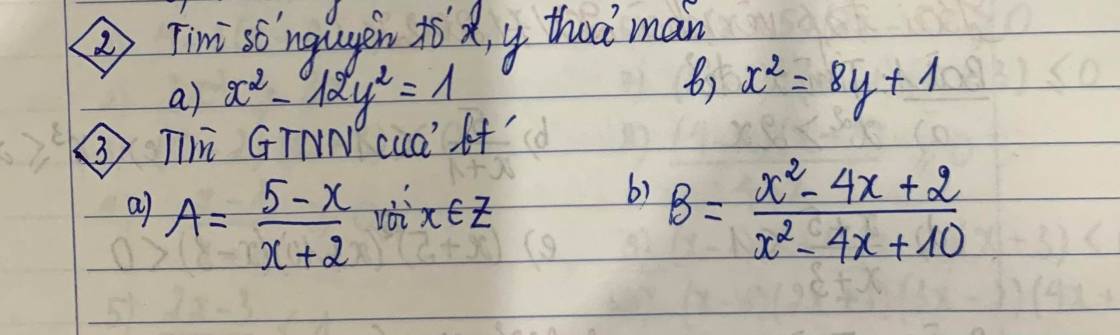
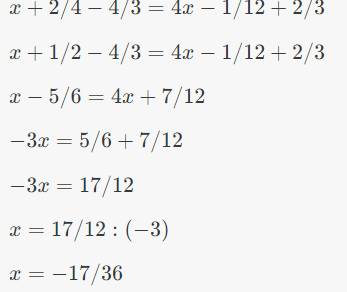

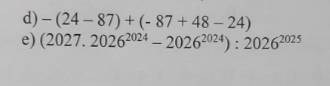
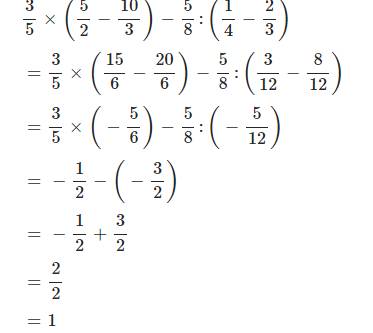
17+(-20)+23+(-26)+...+53+(-56)
=-3-3-3-...-3=-3x14=-52.