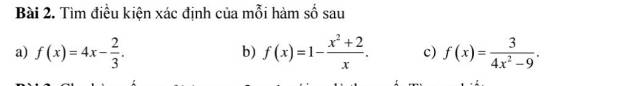
Hãy nhập câu hỏi của bạn vào đây, nếu là tài khoản VIP, bạn sẽ được ưu tiên trả lời.


a) f(2) = 3.2² - 2.2 + 1
= 3.4 - 4 + 1
= 12 - 4 + 1
= 9
Vậy tại x₀ = 2 thì f(x) = 9
b) f(1) = (1 - 3)/(1² + 1)
= -2/2
= -1
Vậy tại x₀ = 1 thì f(x) = -1
a) f(2) = 3.2² - 2.2 + 1
= 3.4 - 4 + 1
= 12 - 4 + 1
= 9
Vậy tại x₀ = 2 thì f(x) = 9
b) f(1) = (1 - 3)/(1² + 1)
= -2/2
= -1
Vậy tại x₀ = 1 thì f(x) = -1
- Cho mình 1 like nha

Lời giải:
a. $3x^2-9x=3x(x-3)$
b. $4x^2+7y-4xy-7x=(4x^2-4xy)-(7x-7y)=4x(x-y)-7(x-y)=(x-y)(4x-7)$

Ta có: \(2x^4-21^3+34x^2+105x+50=0\)
\(\Leftrightarrow2x^4-12x^3-10x^2-9x^3+54x^2+45x-10x^2+60x+50=0\)
\(\Leftrightarrow2x^2\left(x^2-6x-5\right)-9x\left(x^2-6x-5\right)-10\left(x^2-6x-5\right)=0\)
\(\Leftrightarrow\left(x^2-6x-5\right)\left(2x^2-9x-10\right)=0\)
\(\Leftrightarrow\left[{}\begin{matrix}x^2-6x-5=0\\2x^2-9x-10=0\end{matrix}\right.\)\(\Leftrightarrow\left[{}\begin{matrix}x=3+\sqrt{14}\\x=3-\sqrt{14}\\x=\dfrac{9+\sqrt{161}}{4}\\x=\dfrac{9-\sqrt{161}}{4}\end{matrix}\right.\)

Lời giải:
$x^2+x-12=0$
$\Leftrightarrow (x^2-3x)+(4x-12)=0$
$\Leftrightarrow x(x-3)+4(x-3)=0$
$\Leftrightarrow (x-3)(x+4)=0$
$\Leftrightarrow x-3=0$ hoặc $x+4=0$
$\Leftrightarrow x=3$ hoặc $x=-4$

Khi đó, là đường trung tuyến của tam giác .
Vì là trọng tâm của tam giác nên điểm nằm trên cạnh .
Ta có hay .
Vì // , theo định lí Thalès, ta suy ra: .
Ta có (vì là trung điểm của cạnh ) nên .
Do đó (đpcm).

Ta có DE//AC \(\Rightarrow\dfrac{AE}{AB}=\dfrac{CD}{BC}\) (Talet)
Ta có DF//AB \(\Rightarrow\dfrac{AF}{AC}=\dfrac{BD}{BC}\) (Talet)
\(\Rightarrow\dfrac{AE}{AB}+\dfrac{AF}{AC}=\dfrac{CD}{BC}+\dfrac{BD}{BC}=\dfrac{BC}{BC}=1\left(dpcm\right)\)

a)ĐKXĐ: \(x\inℝ\)
b)ĐKXĐ: \(x\ne0\)
c)ĐKXĐ: \(4x^2-9\ne0< =>4x^2\ne9\\ < =>x^2\ne\dfrac{9}{4}\\ < =>x\ne\pm\dfrac{3}{2}\)