Cho hàm số y=\(f\left(x\right)\)=3x2+1.Tính:\(f\left(\frac{1}{2}\right)\);\(f\left(1\right)\);\(f\left(3\right)\)
Hãy nhập câu hỏi của bạn vào đây, nếu là tài khoản VIP, bạn sẽ được ưu tiên trả lời.


\(\text{1)}\)
\(\text{Thay }x=-2,\text{ ta có: }f\left(-2\right)-5f\left(-2\right)=\left(-2\right)^2\Rightarrow f\left(-2\right)=-1\)
\(\Rightarrow f\left(x\right)=x^2+5f\left(-2\right)=x^2-5\)
\(f\left(3\right)=3^2-5\)
\(\text{2)}\)
\(\text{Thay }x=1,\text{ ta có: }f\left(1\right)+f\left(1\right)+f\left(1\right)=6\Rightarrow f\left(1\right)=2\)
\(\text{Thay }x=-1,\text{ ta có: }f\left(-1\right)+f\left(-1\right)+2=6\Rightarrow f\left(-1\right)=2\)
\(\text{3)}\)
\(\text{Thay }x=2,\text{ ta có: }f\left(2\right)+3f\left(\frac{1}{2}\right)=2^2\text{ (1)}\)
\(\text{Thay }x=\frac{1}{2},\text{ ta có: }f\left(\frac{1}{2}\right)+3f\left(2\right)=\left(\frac{1}{2}\right)^2\text{ (2)}\)
\(\text{(1) - 3}\times\text{(2) }\Rightarrow f\left(2\right)+3f\left(\frac{1}{2}\right)-3f\left(\frac{1}{2}\right)-9f\left(2\right)=4-\frac{1}{4}\)
\(\Rightarrow-8f\left(2\right)=\frac{15}{4}\Rightarrow f\left(2\right)=-\frac{15}{32}\)

• Xét hàm số \(f\left( x \right) = 2x - \sin x\) có tập xác định \(D = \mathbb{R}\).
Vậy hàm số \(f\left( x \right)\) liên tục trên \(\mathbb{R}\).
• Xét hàm số \(g\left( x \right) = \sqrt {x - 1} \)
ĐKXĐ: \(x - 1 \ge 0 \Leftrightarrow x \ge 1\)
Hàm số \(g\left( x \right) = \sqrt {x - 1} \) có tập xác định \(D = \left[ {1; + \infty } \right)\).
Hàm số \(g\left( x \right) = \sqrt {x - 1} \) là hàm căn thức nên liên tục trên khoảng \(\left( {1; + \infty } \right)\).
Ta có: \(\mathop {\lim }\limits_{x \to {1^ + }} g\left( x \right) = \mathop {\lim }\limits_{x \to {1^ + }} \sqrt {x - 1} = \sqrt {1 - 1} = 0 = g\left( 1 \right)\)
Do đó hàm số \(g\left( x \right) = \sqrt {x - 1} \) liên tục tại điểm \({x_0} = 1\).
Vậy hàm số \(g\left( x \right) = \sqrt {x - 1} \) liên tục trên nửa khoảng \(\left[ {1; + \infty } \right)\).
• Xét hàm số \(y = f\left( x \right).g\left( x \right) = \left( {2x - \sin x} \right)\sqrt {x - 1} \)
Do hàm số \(y = f\left( x \right)\) và \(y = g\left( x \right)\) đều liên tục tại mọi điểm \({x_0} \in \left[ {1; + \infty } \right)\) nên hàm số \(y = f\left( x \right).g\left( x \right)\) liên tục trên nửa khoảng \(\left[ {1; + \infty } \right)\).
• Xét hàm số \(y = \frac{{f\left( x \right)}}{{g\left( x \right)}} = \frac{{2x - \sin x}}{{\sqrt {x - 1} }}\)
Do hàm số \(y = f\left( x \right)\) và \(y = g\left( x \right)\) đều liên tục tại mọi điểm \({x_0} \in \left[ {1; + \infty } \right)\) nên hàm số \(y = \frac{{f\left( x \right)}}{{g\left( x \right)}}\) liên tục trên khoảng \(\left( {1; + \infty } \right)\).

\(f\left( { - 3} \right) = - {\left( { - 3} \right)^2} + 1 = - 9 + 1 = - 8\);
\(f\left( { - 2} \right) = - {\left( { - 2} \right)^2} + 1 = - 4 + 1 = - 3\);
\(f\left( { - 1} \right) = - {\left( { - 1} \right)^2} + 1 = - 1 + 1 = 0\);
\(f\left( 0 \right) = - {0^2} + 1 = 0 + 1 = 1\);
\(f\left( 1 \right) = - {1^2} + 1 = - 1 + 1 = 0\);

\(f\left( { - 3} \right) = {\left( { - 3} \right)^2} + 4 = 9 + 4 = 13\);
\(f\left( { - 2} \right) = {\left( { - 2} \right)^2} + 4 = 4 + 4 = 8\);
\(f\left( { - 1} \right) = {\left( { - 1} \right)^2} + 4 = 1 + 4 = 5\);
\(f\left( 0 \right) = {0^2} + 4 = 0 + 4 = 4\);
\(f\left( 1 \right) = {1^2} + 4 = 1 + 4 = 5\).

a)
\(\begin{array}{l}f'\left( 1 \right) = \mathop {\lim }\limits_{x \to 1} \frac{{f\left( x \right) - f\left( 1 \right)}}{{x - 1}} = \mathop {\lim }\limits_{x \to 1} \frac{{\frac{1}{2}{x^2} - \frac{1}{2}}}{{x - 1}} = \mathop {\lim }\limits_{x \to 1} \frac{{\frac{1}{2}\left( {{x^2} - 1} \right)}}{{x - 1}} = \mathop {\lim }\limits_{x \to 1} \frac{{\frac{1}{2}\left( {x - 1} \right)\left( {x + 1} \right)}}{{x - 1}}\\ = \mathop {\lim }\limits_{x \to 1} \frac{1}{2}\left( {x + 1} \right) = \frac{1}{2}\left( {1 + 1} \right) = 1\end{array}\)
b) Phương trình đường thẳng \(d\) đi qua điểm \(M\left( {1;\frac{1}{2}} \right)\) và có hệ số góc bằng \(k = f'\left( 1 \right) = 1\) là: \(y - \frac{1}{2} = 1\left( {x - 1} \right) \Leftrightarrow y = x - 1 + \frac{1}{2} \Leftrightarrow y = x - \frac{1}{2}\).
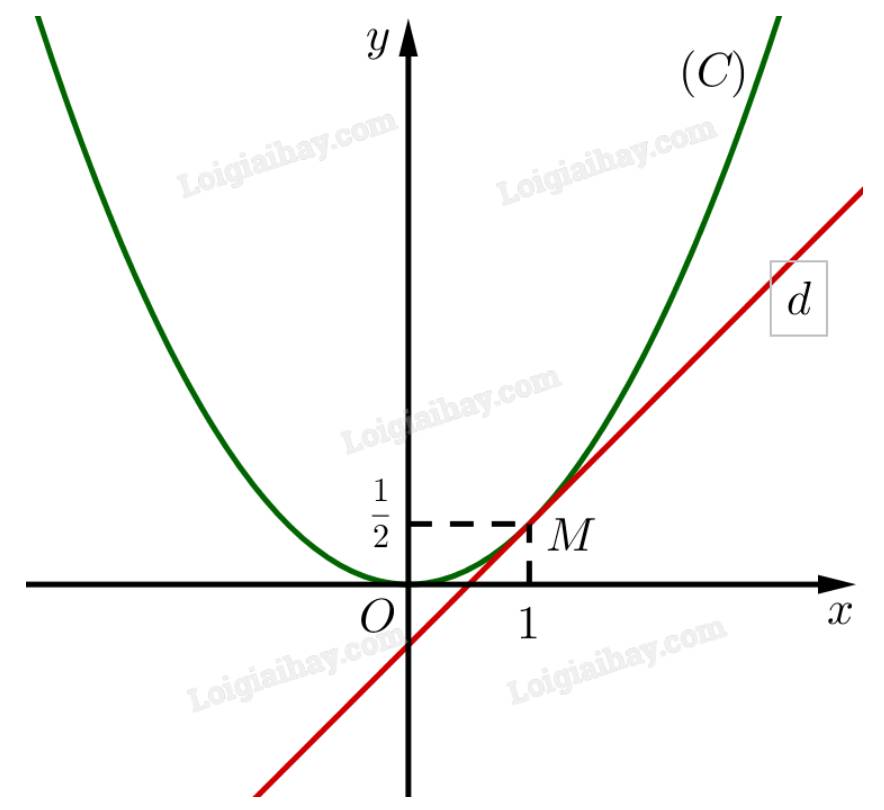
Đường thẳng \(d\) cắt đồ thị hàm số \(\left( C \right)\) tại duy nhất điểm \(M\left( {1;\frac{1}{2}} \right)\).

\(f\left(x\right)=\frac{x^2+2x+1-x^2}{x^2\left(x+1\right)^2}=\frac{\left(x+1\right)^2-x^2}{x^2\left(x+1\right)^2}=\frac{1}{x^2}-\frac{1}{\left(x+1\right)^2}\)
\(\Rightarrow f\left(1\right)+f\left(2\right)+....+f\left(x\right)=1-\frac{1}{2^2}+\frac{1}{2^2}-....-\frac{1}{\left(x+1\right)^2}\)
\(\Rightarrow\frac{2y\left(x+1\right)^3-1}{\left(x+1\right)^2}-19+x=\frac{x\left(x+2\right)}{\left(x+1\right)^2}\)
\(\Leftrightarrow\frac{2y\left(x+1\right)^3-1}{\left(x+1\right)^2}-19+x=\frac{2y\left(x+1\right)^3-1}{\left(x+1\right)^2}-20+\left(x+1\right)=\frac{x\left(x+2\right)}{\left(x+1\right)^2}\)
Dat:\(x+1=a\Rightarrow\frac{\left(2y+1\right)a^3-20a^2-1}{a^2}=\frac{a^2-1}{a^2}\Leftrightarrow\left(2y+1\right)a^3-20a^2-1=a^2-1\)
\(\Leftrightarrow\left(2y+1\right)a^3-20a^2=a^2\Leftrightarrow\left(2ay+a\right)-20=1\left(coi:x=-1cophailanghiemko\right)\)
\(\Leftrightarrow2ay+a=21\Leftrightarrow a\left(2y+1\right)=21\Leftrightarrow\left(x+1\right)\left(2y+1\right)=21\)

Đặt \(h\left( x \right) = f\left( x \right) + g\left( x \right) = \frac{1}{{x - 1}} + \sqrt {4 - x} \). Ta có:
\(\begin{array}{l}h\left( 2 \right) = \frac{1}{{2 - 1}} + \sqrt {4 - 2} = 1 + \sqrt 2 \\\mathop {\lim }\limits_{x \to 2} h\left( x \right) = \mathop {\lim }\limits_{x \to x} \left( {\frac{1}{{x - 1}} + \sqrt {4 - x} } \right) = \frac{1}{{2 - 1}} + \sqrt {4 - 2} = 1 + \sqrt 2 \end{array}\)
Vì \(\mathop {\lim }\limits_{x \to 2} h\left( x \right) = h\left( 2 \right)\) nên hàm số \(y = f\left( x \right) + g\left( x \right)\) liên tục tại \(x = 2\).