Khi nào thì tích vô hướng của hai vectơ \(\overrightarrow u ,\;\overrightarrow v \) là một số dương? Là một số âm?
Hãy nhập câu hỏi của bạn vào đây, nếu là tài khoản VIP, bạn sẽ được ưu tiên trả lời.


Có \(\overrightarrow{a}.\overrightarrow{b}=\left|\overrightarrow{a}\right|.\left|\overrightarrow{b}\right|.cos\left(\overrightarrow{a},\overrightarrow{b}\right)\).
Vì vậy:
\(\overrightarrow{a}.\overrightarrow{b}< 0\) khi \(cos\left(\overrightarrow{a},\overrightarrow{b}\right)< 0\) hay \(90^o< \left(\overrightarrow{a},\overrightarrow{b}\right)\le180^o\).
\(\overrightarrow{a}.\overrightarrow{b}>0\) khi \(cos\left(\overrightarrow{a},\overrightarrow{b}\right)>0\) hay \(0^o\le\left(\overrightarrow{a},\overrightarrow{b}\right)< 90^o\).
\(\overrightarrow{a}.\overrightarrow{b}=0\) khi \(cos\left(\overrightarrow{a},\overrightarrow{b}\right)=0\) hay \(\left(\overrightarrow{a},\overrightarrow{b}\right)=90^o\).

Ta có: \(\overrightarrow u = \left( {0; - 5} \right),\;\overrightarrow v = \left( {\sqrt 3 ;1} \right)\)
\( \Rightarrow \overrightarrow u .\;\,\overrightarrow v = 0.\sqrt 3 + \left( { - 5} \right).1 = - 5.\)

Ta có: \(\overrightarrow a .\overrightarrow b = \left| {\overrightarrow a } \right|.\left| {\overrightarrow b } \right|.\cos \left( {\overrightarrow a ,\overrightarrow b } \right)\)
\( \Leftrightarrow 12\sqrt 2 = 3.8.\cos \left( {\overrightarrow a ,\overrightarrow b } \right) \Leftrightarrow \cos \left( {\overrightarrow a ,\overrightarrow b } \right) = \frac{{\sqrt 2 }}{2}\)
\( \Rightarrow \left( {\overrightarrow a ,\overrightarrow b } \right) = 45^\circ \)
Vậy góc giữa hai vectơ \(\overrightarrow a \) và \(\overrightarrow b \) là \(45^\circ \)

\(cos\left(\alpha-\beta\right)=x_M\cdot x_N=cos\alpha\cdot cos\beta+sin\alpha\cdot sin\beta\\ cos\left(\alpha+\beta\right)=cos\left[\alpha-\left(-\beta\right)\right]=cos\alpha\cdot cos\left(-\beta\right)+sin\alpha\cdot sin\left(-\beta\right)=cos\alpha\cdot cos\beta-sin\alpha\cdot sin\beta\)

Tích vô hướng của hai vec tơ a→ và b→:
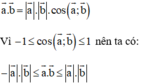
+ a→.b→ đạt giá trị lớn nhất bằng 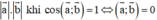 ⇔ a→ và b→ cùng hướng.
⇔ a→ và b→ cùng hướng.
+ a→.b→ đạt giá trị nhỏ nhất bằng 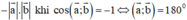 ⇔ a→ và b→ ngược hướng.
⇔ a→ và b→ ngược hướng.

a) Ta có: \(\overrightarrow {OM} = \left( {2;1} \right),\overrightarrow {MN} = \left( { - 3;2} \right),\overrightarrow {MP} = \left( {2;1} \right)\)
b) Ta có: \(\overrightarrow {MN} .\overrightarrow {MP} = - 3.2 + 2.1 = - 4\)
c) Ta có: \(MN = \left| {\overrightarrow {MN} } \right| = \sqrt {{{\left( { - 3} \right)}^2} + {2^2}} = \sqrt {13} ,MP = \left| {\overrightarrow {MP} } \right| = \sqrt {{2^2} + {1^2}} = \sqrt 5 \)
d) Ta có: \(\cos \widehat {MNP} = \frac{{\overrightarrow {MN} .\overrightarrow {MP} }}{{\left| {\overrightarrow {MN} } \right|.\left| {\overrightarrow {MP} } \right|}} = \frac{- 4}{{\sqrt {13} .\sqrt 5 }} = \frac{- 4}{{\sqrt {65} }}\)
e) Tọa độ trung điểm I của đoạn NP là: \(\left\{ \begin{array}{l}{x_I} = \frac{{{x_N} + {x_P}}}{2} = \frac{3}{2}\\{y_I} = \frac{{{y_N} + {y_P}}}{2} = \frac{5}{2}\end{array} \right. \Leftrightarrow I\left( {\frac{3}{2};\frac{5}{2}} \right)\)
Tọa độ trọng tâm G của tam giác MNP là: \(\left\{ \begin{array}{l}{x_G} = \frac{{{x_M} + {x_N} + {x_P}}}{3}\\{y_G} = \frac{{{y_M} + {y_N} + {y_P}}}{3}\end{array} \right. \Leftrightarrow \left\{ \begin{array}{l}{x_G} = \frac{5}{3}\\{y_C} = 2\end{array} \right. \Leftrightarrow G\left( {\frac{5}{3};2} \right)\)

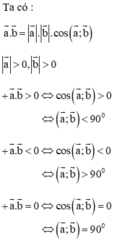

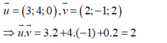
Tham khảo:
Dễ thấy: \(\overrightarrow u .\;\overrightarrow v \) cùng dấu với \(\cos \;\left( {\overrightarrow u ,\;\overrightarrow v } \right)\) (do \(\left| {\overrightarrow u } \right|.\;\left| {\overrightarrow v } \right| > 0\)). Do đó:
+) \(\overrightarrow u .\;\overrightarrow v \;\; > 0\) \( \Leftrightarrow \cos \;\left( {\overrightarrow u ,\;\overrightarrow v } \right) > 0\) hay \({0^o} \le \left( {\overrightarrow u ,\;\overrightarrow v } \right) < {90^o}\)
+) \(\overrightarrow u .\;\overrightarrow v \;\; < 0\) \( \Leftrightarrow \cos \;\left( {\overrightarrow u ,\;\overrightarrow v } \right)\;\; < 0\) hay \({90^o} < \left( {\overrightarrow u ,\;\overrightarrow v } \right) \le {180^o}\)
Vậy \(\overrightarrow u .\;\overrightarrow v \;\; > 0\) nếu \({0^o} \le \left( {\overrightarrow u ,\;\overrightarrow v } \right) < {90^o}\) và \(\overrightarrow u .\;\overrightarrow v \;\; < 0\) nếu \({90^o} < \left( {\overrightarrow u ,\;\overrightarrow v } \right) \le {180^o}.\)