Giải giùm ạ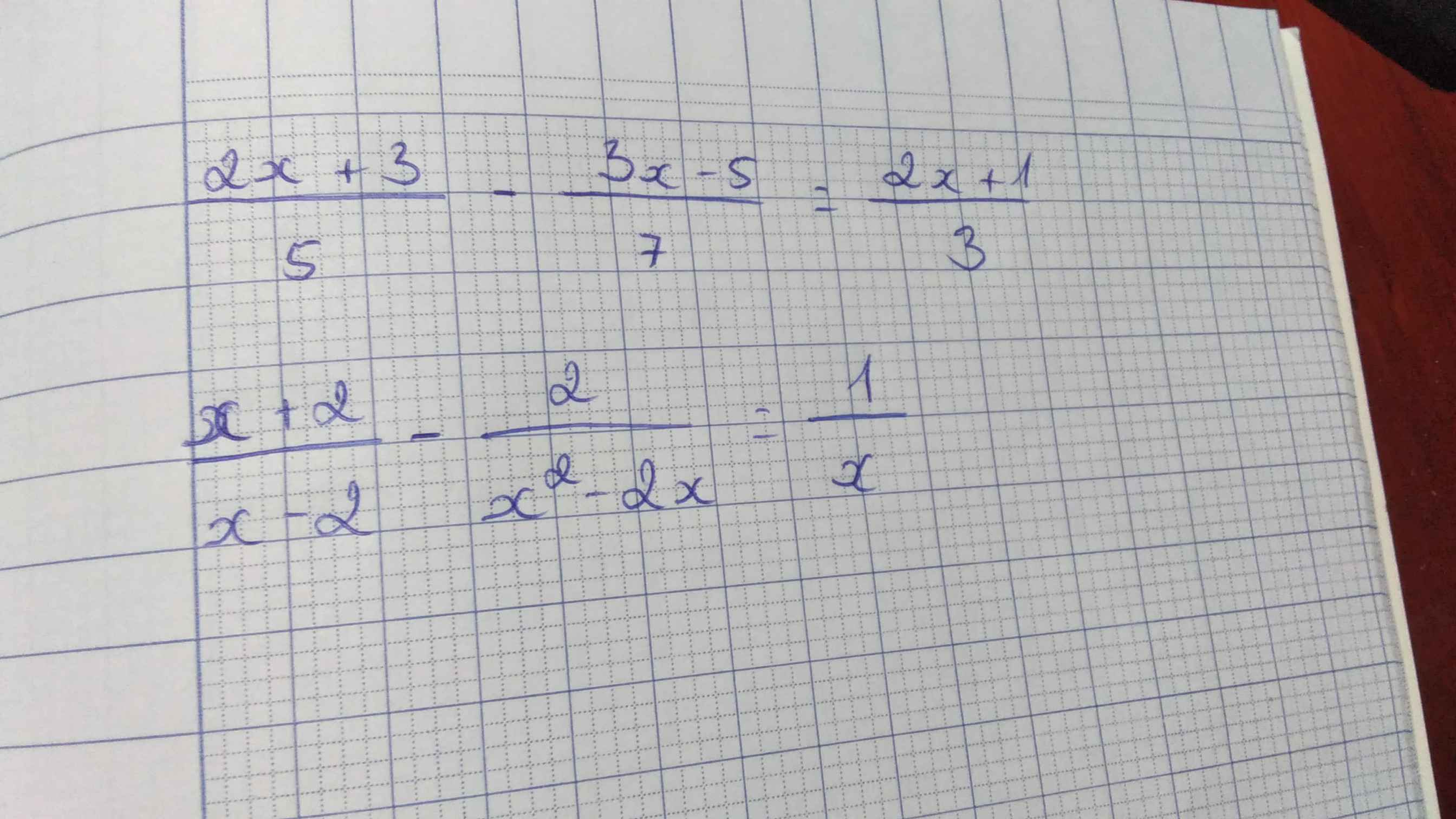
K
Khách
Hãy nhập câu hỏi của bạn vào đây, nếu là tài khoản VIP, bạn sẽ được ưu tiên trả lời.
Những câu hỏi liên quan
3 tháng 12 2016
a) x + 7 = -12
x = (-12) - 7
x = 19
b) x - 15 = -21
x = (-21) + 15
x = -6
c) 13 - x = 20
x = 13 - 20
x = -7
3 tháng 12 2016
a, x+7=-12
\(\Leftrightarrow\) x= -19
b, x-15=-21
\(\Leftrightarrow\) x= -6
c, 13-x=20
\(\Leftrightarrow\) x=-7
27 tháng 7 2021
Ta có: \(2x^2y\cdot\left(x^3y+4x^3y-4x-x^2y+5x^3y-y\right)\)
\(=2x^2y\cdot\left(10x^3y-4x-x^2y-y\right)\)
\(=20x^5y^2-8x^3y-2x^4y^2-2x^2y^2\)
NV
Nguyễn Việt Lâm
Giáo viên
9 tháng 7 2021
Gọi \(\overrightarrow{v}=\left(a;b\right)\)
d nhận \(\left(4;3\right)\) là 1 vtcp, mà \(\overrightarrow{v}\) vuông góc d \(\overrightarrow{v}=\left(3k;-4k\right)\)
Chọn \(M\left(-1;0\right)\in d\) , do \(T_{\overrightarrow{v}}\left(d\right)=d_1\Rightarrow\) ảnh \(M_1\left(x_1;y_1\right)\) của \(M\) qua phép tịnh tiến \(\overrightarrow{v}\) nằm trên \(d_1\)
\(\left\{{}\begin{matrix}x_1=-1+3k\\y_1=0-4k=-4k\end{matrix}\right.\) \(\Rightarrow M_1\left(-1+3k;-4k\right)\)
Thế vào pt \(d_1\)
\(3\left(-1+3k\right)-4.\left(-4k\right)-2=0\) \(\Rightarrow k=\dfrac{1}{5}\)
\(\Rightarrow\overrightarrow{v}=\left(\dfrac{3}{5};-\dfrac{4}{5}\right)\)
6 tháng 11 2021
b: Xét ΔABC vuông tại A có AH là đường cao
nên \(BH\cdot CH=AH^2=AM^2\)
1 tháng 5 2021
a) Ta có: \(\dfrac{7x+4}{5}-x=\dfrac{3x-5}{2}\)
\(\Leftrightarrow\dfrac{2\left(7x+4\right)}{10}-\dfrac{10x}{10}=\dfrac{5\left(3x-5\right)}{10}\)
Suy ra: \(14x+8-10x=15x-25\)
\(\Leftrightarrow4x+8-15x+25=0\)
\(\Leftrightarrow-11x+33=0\)
\(\Leftrightarrow-11x=-33\)
hay x=3
Vậy: S={3}
1 tháng 5 2021
b) ĐKXĐ: \(x\notin\left\{-2;-3\right\}\)
Ta có: \(\dfrac{x-2}{x+2}-\dfrac{x-3}{x+3}+\dfrac{x^2}{\left(x+2\right)\left(x+3\right)}=0\)
\(\Leftrightarrow\dfrac{\left(x-2\right)\left(x+3\right)}{\left(x+2\right)\left(x+3\right)}-\dfrac{\left(x-3\right)\left(x+2\right)}{\left(x+3\right)\left(x+2\right)}+\dfrac{x^2}{\left(x+2\right)\left(x+3\right)}=0\)
Suy ra: \(x^2+3x-2x-6-\left(x^2+2x-3x-6\right)+x^2=0\)
\(\Leftrightarrow2x^2+x-6-x^2+x+6=0\)
\(\Leftrightarrow x^2+2x=0\)
\(\Leftrightarrow x\left(x+2\right)=0\)
\(\Leftrightarrow\left[{}\begin{matrix}x=0\\x+2=0\end{matrix}\right.\Leftrightarrow\left[{}\begin{matrix}x=0\left(nhận\right)\\x=-2\left(loại\right)\end{matrix}\right.\)
Vậy: S={0}
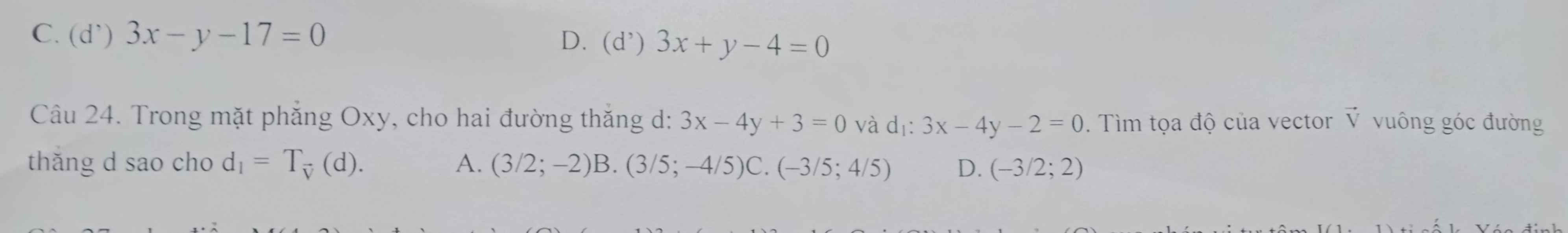
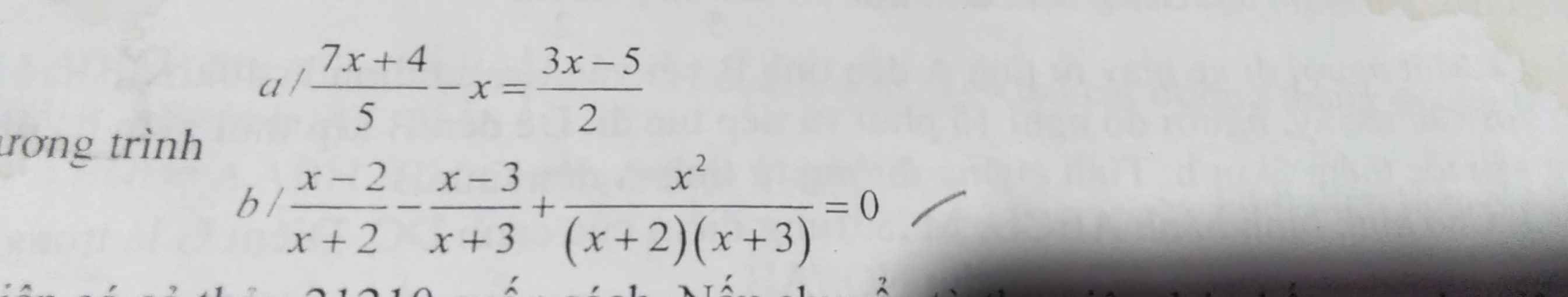
P1. \(\dfrac{2x+3}{5}-\dfrac{3x-5}{7}=\dfrac{2x+1}{3}\)
\(\Leftrightarrow \dfrac{21\left(2x+3\right)}{105}-\dfrac{15\left(3x-5\right)}{105}=\dfrac{35\left(2x+1\right)}{105}\)
\(\Rightarrow 42x+63-45x+75=70x+35\)
\(\Leftrightarrow -3x+138=70x+35\)
\(\Leftrightarrow -3x-70x=-138+35\)
\(\Leftrightarrow -73x=-103\)
\(\Leftrightarrow x=\dfrac{103}{73}\)
Vậy tập No của PT là \(S=\left\{\dfrac{103}{73}\right\}\)
phần còn lại mik chx lm ra :(