Tìm x lớn nhất thỏa mãn: 2.|x+9|=10
Hãy nhập câu hỏi của bạn vào đây, nếu là tài khoản VIP, bạn sẽ được ưu tiên trả lời.



2 . |x + 9| = 10
=> 2 . (x + 9) = 10 hoặc 2 . (x + 9) = -10
=> x + 9 = 10 : 2 hoặc x + 9 = -10 : 2
=> x + 9 = 5 hoặc x + 9 = -5
=> x = 5 - 9 hoặc x = -5 - 9
=> x = -4 hoặc x = -14
Mà x lớn nhất.
=> x = -4

\(3=x+y+xy\le\sqrt{2\left(x^2+y^2\right)}+\dfrac{x^2+y^2}{2}\)
\(\Rightarrow\left(\sqrt{x^2+y^2}-\sqrt{2}\right)\left(\sqrt{x^2+y^2}+3\sqrt{2}\right)\ge0\)
\(\Rightarrow x^2+y^2\ge2\)
\(\Rightarrow-\left(x^2+y^2\right)\le-2\)
\(P=\sqrt{9-x^2}+\sqrt{9-y^2}+\dfrac{x+y}{4}\le\sqrt{2\left(9-x^2+9-y^2\right)}+\dfrac{\sqrt{2\left(x^2+y^2\right)}}{4}\)
\(P\le\sqrt{2\left(18-x^2-y^2\right)}+\dfrac{1}{4}.\sqrt{2\left(x^2+y^2\right)}\)
\(P\le\left(\sqrt{2}-1\right)\sqrt{18-x^2-y^2}+\sqrt[]{2}\sqrt{\dfrac{\left(18-x^2-y^2\right)}{2}}+\dfrac{1}{2}\sqrt{\dfrac{x^2+y^2}{2}}\)
\(P\le\left(\sqrt{2}-1\right).\sqrt{18-2}+\sqrt{\left(2+\dfrac{1}{4}\right)\left(\dfrac{18-x^2-y^2+x^2+y^2}{2}\right)}=\dfrac{1+8\sqrt{2}}{2}\)
Dấu "=" xảy ra khi \(x=y=1\)
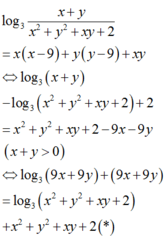

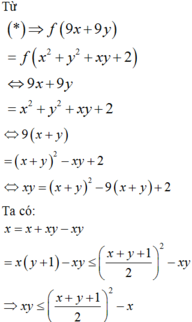
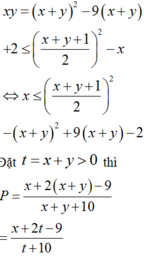
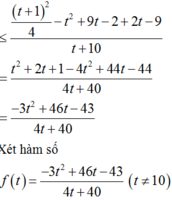
2.|x+9| = 10
=> |x+9| = 5
=> x+ 9 = 5
hoặc x+ 9 = - 5
=> x= - 4
hoặc x= - 14
vậy x= - 4
|x+9|=10:2
|x+9|=5
x+9=5 hoặc x+9=-5
x=5-9 hoặc x=-5-9
x=-4 hoặc x=-14
vậy giá trị x lớn nhất là-4