2021/2018 x 2022 ... ... ... 2019/2020 x 2020
Hãy nhập câu hỏi của bạn vào đây, nếu là tài khoản VIP, bạn sẽ được ưu tiên trả lời.


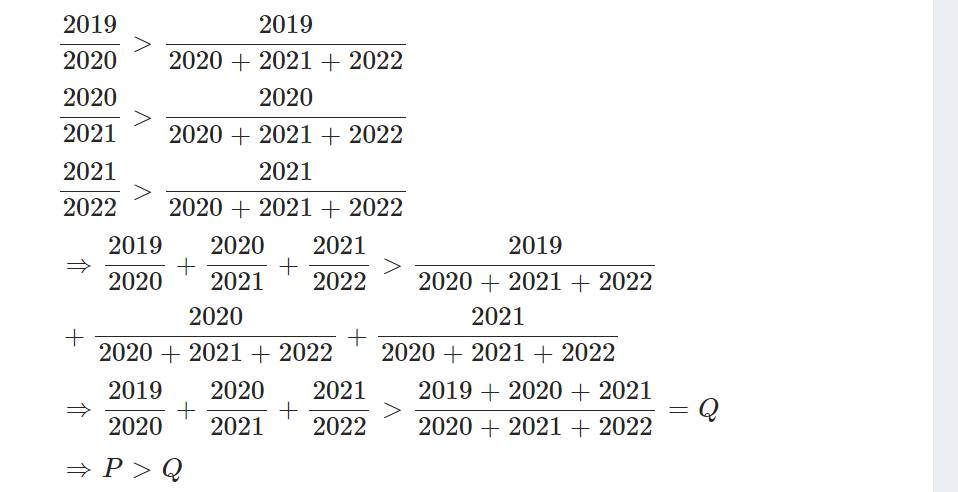
A = 2021/2022+2020/2021+2019/2020+2018/2019+2017/2018
A<2022/2022+2021/2021+2020/2020+2019/2019+2018/2018
A<1+1+1+1+1
A<5

\(\left(1-\frac{1}{2018}\right)\times\left(1-\frac{1}{2019}\right)\times\left(1-\frac{1}{2020}\right)\times\left(1-\frac{1}{2021}\right)\times\left(1-\frac{1}{2022}\right)\)
\(=\frac{2017}{2018}\times\frac{2018}{2019}\times\frac{2019}{2020}\times\frac{2020}{2021}\times\frac{2021}{2022}\)
\(=\frac{2017}{2022}\)

Sửa đề: 1-2-3+4+5-6-7+8+...-2018-2019+2020+2021-2022-2023
=(1-2-3+4)+(5-6-7+8)+...+(2017-2018-2019+2020)+(2021-2022-2023)
=0+0+...+0+(-1-2023)
=-2024

2011+2012+2013+2014+2015+2016+2017+2018+2019+2020+2021+ 2022+2023 =(2011+2023)+(2013+2022)+...+(2016+2018)+2017 =4034+4034+4034+4034+4034+4034+2017 =4034x6+2017=26221
2011+2012+2013+2014+2015+2016+2017+2018+2019+2020+2021+2022+2023
=(2011+2023)+(2013+2022)+...+(2016+2018)+2017 =4034+4034+4034+4034+4034+4034+2017 =4034x6+2017=26221

x=2020 nên x+1=2021
\(P\left(x\right)=x^{2021}-x^{2020}\left(x+1\right)+x^{2019}\left(x+1\right)-....+x\left(x+1\right)-2020\)
\(=x^{2021}-x^{2021}-x^{2020}+x^{2020}-...+x^2+x-2020\)
=x-2020=0

Cho đa thức \(f\left(x\right)\)bậc 3 với hệ số \(x^3\)là số nguyên dương thỏa mãn:
\(f\left(2019\right)=2020;f\left(2020\right)=2021\)
CMR \(f\left(2021\right)-f\left(2018\right)\)là hợp số