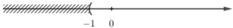
giải bất phương trình và biểu diễn tập nghiệm trên trục số:x(x-1)-3(x-2)<x^2-4(x+2)
K
Khách
Hãy nhập câu hỏi của bạn vào đây, nếu là tài khoản VIP, bạn sẽ được ưu tiên trả lời.
Những câu hỏi liên quan
TT
1
5 tháng 8 2021
Ta có: \(x^2< 9\)
\(\Leftrightarrow\left\{{}\begin{matrix}-3< x\\x< 3\end{matrix}\right.\Leftrightarrow-3< x< 3\)
CV
2
5 tháng 4 2023
\(x-8\ge2\left(\dfrac{x+1}{2}\right)+7\)
⇔\(x-8\ge x+1+7\)
⇔\(x-x\ge1+7+8\)
⇔\(0x\ge16\)(vô lí)
Tập nghiệm của bất phương trình là :
\(S=\left\{\varnothing\right\}\)
H
18 tháng 4 2023
\(\dfrac{x-2}{2}+1\le\dfrac{x-1}{3}\)
\(\Leftrightarrow\dfrac{3\left(x-2\right)}{6}+\dfrac{1.6}{6}\le\dfrac{2\left(x-1\right)}{6}\)
`<=> 3x - 6 + 6 <= 2x-2`
`<=> 3x <= 2x-2`
`<=> 3x -2x <= -2`
`<=> x <= -2`
18 tháng 4 2023
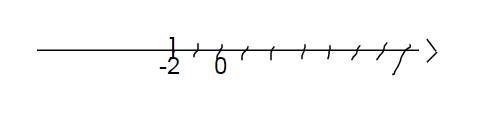
\(\dfrac{x-2}{2}\)+1≤\(\dfrac{x-1}{3}\)
<=>\(\dfrac{3x-6}{6}\)+\(\dfrac{6}{6}\)≤\(\dfrac{2x-1}{6}\)
<=>3x-6+6≤2x-1
<=>x<-1
PT
1
CM
12 tháng 4 2019
x – 1 < 3
⇔ x < 3 + 1 (Chuyển vế và đổi dấu hạng tử -1)
⇔ x < 4
Vậy bất phương trình có nghiệm x < 4.
16 tháng 6 2023
=>2x^2-3x-4x+6+3x+8<2x^2+4x+2-4x
=>2x^2-4x+14<2x^2+2
=>-4x<-12
=>x>3
12 tháng 5 2022
a: 2x-1>=5
nên 2x>=6
hay x>=3
b: \(\dfrac{x-2}{3}>=x-\dfrac{x-1}{2}\)
=>2x-4>=6x-3(x-1)
=>2x-4>=6x-3x+3
=>2x-4>=3x+3
=>-x>=7
hay x<=-7
12 tháng 5 2022
a.\(2x-1\ge5\)
\(\Leftrightarrow2x\ge6\)
\(\Leftrightarrow x\ge3\)
Vậy \(S=\left\{x|x\ge3\right\}\)
b.\(\dfrac{x-2}{3}\ge x-\dfrac{x-1}{2}\)
\(\Leftrightarrow\dfrac{2\left(x-2\right)}{6}\ge\dfrac{6x-3\left(x-1\right)}{6}\)
\(\Leftrightarrow2\left(x-2\right)\ge6x-3\left(x-1\right)\)
\(\Leftrightarrow2x-4\ge6x-3x+3\)
\(\Leftrightarrow-x\ge7\)
\(\Leftrightarrow x\le7\)
Vậy \(S=\left\{x|x\le7\right\}\)
PT
1
CM
14 tháng 8 2019
x + 2 > 1
⇔ x > 1 – 2
⇔ x > -1.
Vậy bất phương trình có nghiệm x > -1.
K
0