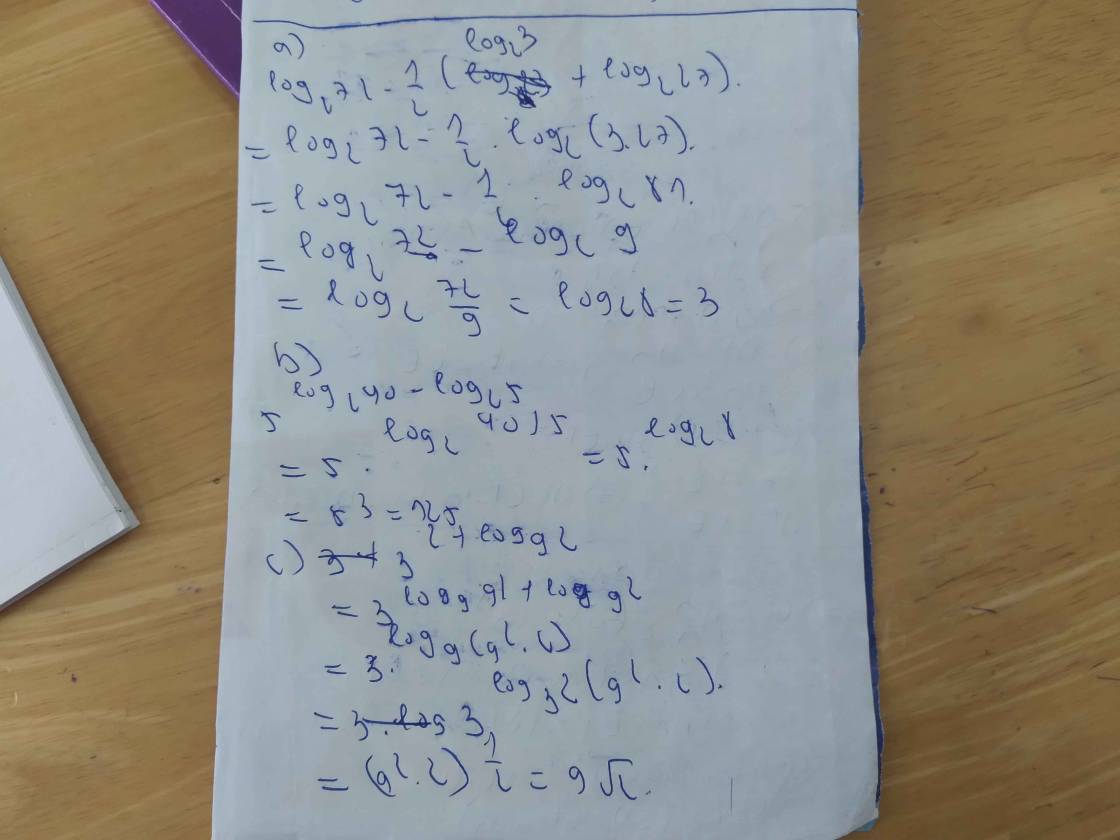\(x+x^{log_23}=x^{log_25}\)
K
Khách
Hãy nhập câu hỏi của bạn vào đây, nếu là tài khoản VIP, bạn sẽ được ưu tiên trả lời.
Những câu hỏi liên quan
HQ
Hà Quang Minh
Giáo viên
26 tháng 8 2023
\(a,log_272-\dfrac{1}{2}\left(log_23+log_227\right)\\ =log_272-\dfrac{1}{2}log_2\left(3\cdot27\right)\\ =log_272-log_2\left(81\right)^{\dfrac{1}{2}}\\ =log_272-log_29\\ =log_2\dfrac{72}{9}\\ =log_28\\ =3\)
\(b,5^{log_240-log_25}\\ =5^{log_2\dfrac{40}{5}}\\ =5^{log_28}\\ =5^3\\ =125\)
\(c,3^{2+log_92}\\ =3^{log_9\left(81\cdot2\right)}\\ =3^{\dfrac{1}{2}log_3162}\\ =\left(162\right)^{\dfrac{1}{2}}\\ =\sqrt{162}\\ =9\sqrt{2}\)
NV
Nguyễn Việt Lâm
Giáo viên
12 tháng 1
\(log_216=log_22^4=4\)
\(log_32187=log_33^7=7\)
\(log_{10}\dfrac{1}{100}=log_{10}10^{-2}=-2\)
\(log10000=log10^4=4\)
\(9^{log_312}=3^{2log_312}=3^{log_3144}=144\)
\(8^{log_25}=2^{3log_25}=2^{log_2125}=125\)
\(\left(\dfrac{1}{25}\right)^{log_5\dfrac{1}{3}}=5^{-2log_5\dfrac{1}{3}}=5^{log_59}=9\)
\(\left(\dfrac{1}{4}\right)^{log_23}=2^{-2log_23}=2^{log_2\dfrac{1}{9}}=\dfrac{1}{9}\)
11 tháng 5 2016
Ta có : \(D=\log_6\left(21,6\right)=\frac{\log_2\left(21,6\right)}{\log_26}=\frac{\log_2\frac{2^2.3^3}{5}}{\log_2\left(2.3\right)}=\frac{2+3\log_23-\log_25}{1+\log_23}=\frac{2+3a-b}{1+a}\)
AH
Akai Haruma
Giáo viên
27 tháng 1 2019
Lời giải:
ĐK: \(x\in (0;+\infty)\)
\(x^{\log_29}=x^2.3^{\log_2x}-x^{\log_23}\)
\(\Leftrightarrow x^{2\log_23}=x^2.x^{\log_23}-x^{\log_23}=x^{\log_23+2}-x^{\log_23}\)
\(\Leftrightarrow x^{\log_23}(x^{\log_23}-x^2+1)=0\). Do $x\neq 0$ nên:
\(x^2-x^{\log_23}=1(*)\)
Nếu \(0< x\leq 1\Rightarrow x^2\leq 1; x^{\log_23}>0\Rightarrow x^2-x^{\log_23}< 1\) (vô lý). Do đó \(x\in (1;+\infty)\)
Đặt \(f(x)=x^2-x^{\log_23}\Rightarrow f'(x)=2x-\log_23x^{\log_23-1}\)
\(=x^{\log_23-1}(2x^{2-\log_23}-\log_23)>x^{\log_23-1}(2.1-\log_23)>0\)với mọi $x\in (1;+\infty)$ nên $f(x)$ đồng biến với mọi $x\in (1;+infty)$. Mà ở vế phải thì $1$ là hàm hằng. Do đó $(*)$ chỉ có nghiệm duy nhất.
Dễ thấy $x=2$ là nghiệm duy nhất của pt
NV
Nguyễn Việt Lâm
Giáo viên
25 tháng 6 2021
ĐKXĐ: \(-x^2+4x+m>0\)
\(log_2\left(-x^2+4x+m\right)-log_2\left(x^2+2\right)< log_23\)
\(\Leftrightarrow log_2\left(\dfrac{-x^2+4x+m}{x^2+2}\right)< log_23\)
\(\Leftrightarrow\dfrac{-x^2+4x+m}{x^2+2}< 3\)
\(\Leftrightarrow\left\{{}\begin{matrix}-x^2+4x+m>0\\-x^2+4x+m< 3x^2+6\end{matrix}\right.\)
\(\Leftrightarrow\left\{{}\begin{matrix}m>x^2-4x\\m< 4x^2-4x+6\end{matrix}\right.\) ; \(\forall x\in\left[1;5\right]\)
Xét hai hàm \(\left\{{}\begin{matrix}f\left(x\right)=x^2-4x\\g\left(x\right)=4x^2-4x+6\end{matrix}\right.\) trên \(\left[1;5\right]\) ta được: \(\left\{{}\begin{matrix}f\left(x\right)_{max}=f\left(5\right)=5\\g\left(x\right)_{min}=g\left(1\right)=6\end{matrix}\right.\)
\(\Rightarrow5\le m\le6\)
Có 2 giá trị nguyên của m
AH
Akai Haruma
Giáo viên
25 tháng 1 2017
Lời giải:
a) Vì \(6^x-2^x>0\Rightarrow x>0\)
Xét \(y=6^x-2^x-32\) có \(y'=\ln 6.6^x-\ln 2.2^x>0\forall x>0\) nên hàm $y$ đồng biến trên \(x\in(0,+\infty)\).
Khi đó phương trình \(6^x-2^x=32\) có nghiệm duy nhất $x=2$
b) Có \(5^{7^x}=7^{5^x}\Leftrightarrow \log(5^{7^x})=\log (7^{5^x})\)
\(\Leftrightarrow 7^x\log 5=5^x\log 7=7^{x\frac{\log 5}{\log 7}}\log 7\)
\(\Leftrightarrow 7^{x(1-\frac{\log 5}{\log 7})}=\frac{\log 7}{\log 5}=10^{x\log 7(1-\frac{\log 5}{\log 7})}=10^{x\log(\frac{7}{5})}\)
\(\Leftrightarrow x\log\frac{7}{5}=\log\left ( \frac{\log 7}{\log 5} \right )\)\(\Rightarrow x=\frac{\log\left ( \frac{\log 7}{\log 5} \right )}{\log\frac{7}{5}}\)
AH
Akai Haruma
Giáo viên
27 tháng 1 2017
d) ĐKXĐ:...........
\(3^x+\frac{1}{3^x}=\sqrt{8-x^2}\Leftrightarrow 9^x+\frac{1}{9^x}+2=8-x^2\)
\(\Leftrightarrow 9^x+\frac{1}{9^x}+x^2=6\)
Giả sử \(x\geq 0\) . Xét hàm \(y=9^x+\frac{1}{9^x}+x^2\) có \(y'=9^x\ln 9-\frac{\ln 9}{9^x}+2x\geq 0\) nên hàm đồng biến trên \(x\in [0,+\infty)\)
Do đó PT \(9^x+\frac{1}{9^x}+x^2=6\) với $x\geq 0$ có nghiệm duy nhất \(x\approx 0,753897\)
---------------------------------------------------------------------------------
Vì hàm \(y\) là hàm chẵn nên $-x$ cũng là nghiệm, do đó tổng kết lại PT có nghiệm là \(x\approx \pm 0,753897\)
NV
Nguyễn Việt Lâm
Giáo viên
30 tháng 6 2020
18.
\(F\left(x\right)=\int\limits xe^{x^2}dx\)
Đặt \(t=x^2\Rightarrow xdx=\frac{1}{2}dt\)
\(\Rightarrow F\left(x\right)=\frac{1}{2}\int e^tdt=\frac{1}{2}e^t+C=\frac{1}{2}e^{x^2}+C\)
Ủa bạn có ghi nhầm đáp án A ko? Thế nào thì cả A và D đều ko phải nguyên hàm
19.
\(F\left(x\right)=\int sin^4xcosxdx=\int sin^4x.d\left(sinx\right)=\frac{1}{5}sin^5x+C\)
20.
Đặt \(4x=t\Rightarrow dx=\frac{1}{4}dt\) ; \(\left\{{}\begin{matrix}x=0\Rightarrow t=0\\x=2\Rightarrow t=8\end{matrix}\right.\)
\(\int\limits^2_0f\left(4x\right)dx=\int\limits^8_0\frac{1}{4}f\left(t\right)dt=\frac{1}{4}\int\limits^8_0f\left(x\right)dx=\frac{1}{4}.24=6\)
NV
Nguyễn Việt Lâm
Giáo viên
30 tháng 6 2020
15.
\(t=cosx\Rightarrow sinx.dx=-dt\) ; \(\left\{{}\begin{matrix}x=0\Rightarrow t=1\\x=\frac{\pi}{2}\Rightarrow t=0\end{matrix}\right.\)
\(\Rightarrow I=\int\limits^0_1e^t\left(-dt\right)=\int\limits^1_0e^tdt\)
Nếu cần kết quả tích phân thì \(I=e-1\)
16.
\(t=x^2\Rightarrow x.dx=\frac{1}{2}dt\) ; \(\left\{{}\begin{matrix}x=0\Rightarrow t=0\\x=2\Rightarrow t=4\end{matrix}\right.\)
\(\Rightarrow I=\int\limits^4_04^t\left(\frac{1}{2}dt\right)=\frac{1}{2}\int\limits^4_04^tdt\)
17.
\(t=x^2+2x\Rightarrow\left(x+1\right)dx=\frac{1}{2}dt\) ; \(\left\{{}\begin{matrix}x=0\Rightarrow t=0\\x=1\Rightarrow t=3\end{matrix}\right.\)
\(\Rightarrow I=\int\limits^3_0e^t\left(\frac{1}{2}dt\right)=\frac{1}{2}\int\limits^3_0e^tdt\)
NV
Nguyễn Việt Lâm
Giáo viên
14 tháng 1
1.
\(A=3log_{2^2}\sqrt{a}-log_{2^{-1}}a^2+2log_{a^{\dfrac{1}{2}}}a\)
\(=3.\dfrac{1}{2}.\dfrac{1}{2}log_2a-\left(-1\right).2.log_2a+2.2.log_2a\)
\(=\dfrac{27}{4}log_2a\)
2.
\(log_{12}36=\dfrac{log_236}{log_212}=\dfrac{log_2\left(3^2.2^2\right)}{log_2\left(3.2^2\right)}=\dfrac{log_23^2+log_22^2}{log_23+log_22^2}\)
\(=\dfrac{2.log_23+2}{log_23+2}=\dfrac{2a+2}{a+2}\)
15 tháng 1
cho e hỏi tại sao \(3\log_{2^2}\sqrt{a}\) lại bằng \(3.\dfrac{1}{2}.\dfrac{1}{2}\log_2a\) và \(2\log_{a^{\dfrac{1}{2}}}a=2.2.\log_2a\)