Tìm các số nguyên n để đa thức 3n3+10n2-8 chia hết cho đa thức 3n+1
Hãy nhập câu hỏi của bạn vào đây, nếu là tài khoản VIP, bạn sẽ được ưu tiên trả lời.


b: \(\Leftrightarrow3n^3+n^2+9n^2+3n-3n-1-4⋮3n+1\)
\(\Leftrightarrow3n+1\in\left\{1;-1;2;-2;4;-4\right\}\)
\(\Leftrightarrow n\in\left\{0;-1;1\right\}\)

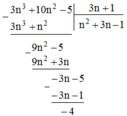
Ta có: 3 n 3 + 10 n 2 - 5 = 3 n + 1 n 2 + 3 n - 1 - 4
Để phép chia đó là chia hết thì 4 ⋮ 3n + 1⇒ 3n + 1 ∈ Ư(4)
3n + 1 ∈ {-4; -2; -1; 1; 2; 4}
3n + 1 = -4⇒ 3n = -5⇒ n = 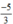 ∉ Z : loại
∉ Z : loại
3n + 1 = -2⇒ 3n = -3⇒ n = -1 ∈ Z
3n + 1 = -1⇒ 3n = -2⇒ n = 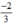 ∉ Z : loại
∉ Z : loại
3n + 1 = 1⇒ 3n = 0⇒ n = 0 ∈ Z
3n + 1 = 2⇒ 3n = 2⇒ n = 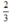 ∉ Z : loại
∉ Z : loại
3n + 1 = 4⇒ 3n = 3⇒ n = 1 ∈ Z
Vậy n ∈ {-1; 0; 1} thì 3 n 3 + 10 n 2 - 5 chia hết cho 3n + 1.



x^4 -x ^3 + 6x^2 - x + n x^2-x+5 x^2+1 - x^4-x^3+5x^2 x^2-x+n - x^2-x+n 0
ĐỂ x4 - x3 + 6x2 -x \(⋮x^2-x+5\)
\(\Rightarrow x-5=0\Rightarrow x=5\)
b , ta có : \(3x^3+10x^2-5⋮3x+1\)
\(\Rightarrow3x^3+x^2+9x^2+3x-3x-1-4⋮3x+1\)
\(\Rightarrow x\left(3x+1\right)+3x\left(3x+1\right)-\left(3x+1\right)-4⋮3x+1\)
mà : \(\left(3x+1\right)\left(4x-1\right)⋮3x+1\)
\(\Rightarrow4⋮3x+1\Rightarrow3x+1\inƯ\left(4\right)=\left\{\pm1;\pm2;\pm4\right\}\)
Nếu : 3x + 1 = 1 => x = 0 ( TM )
3x + 1 = -1 => x = -2/3 ( loại )
3x + 1 = 2 => x = 1/3 ( loại )
3x + 1 = -2 => x = -1 ( TM )
3x + 1 = 4 => x = 1 ( TM )
3x + 1 = -1 => x = -5/3 ( loại )
\(\Rightarrow x\in\left\{0;\pm1\right\}\)

n^4 - 3x^3 + n^2 - 3n + 1 n^2 + 1 n^2 - 3n n^4 + n^2 - 3n^3 - 3n + 1 - 3n^3 -3n 1
Để chia \(n^4-3n^3+n^2-3n+1\) cho \(n^2+1\) có giá trị nguyên
⇔ \(n^4-3n^3+n^2-3n+1\) \(⋮n^2+1\)
⇔ \(1⋮n^2+1\)
\(\Leftrightarrow n^2+1\inƯ\left(1\right)=\left\{1;-1\right\}\)
| n2 + 1 | 1 | -1 |
|
n |
0 | ( loại ) |
Ta co : 3.n^3+10.n^2-5
=3.n^3+9.n^2+3n-3n-1-4
=n^2.(3n+1)+3n(3n+1)-(3n+1)-4
=(3n+1)(n^2+3n-1)-4
De 3.=10.-5 chia het cho 3n+1
=> (3n+1)(3n-1)-4 chia het cho 3n+1
=> -4 chia het cho 3n+1
Ma U(-4)={-4;-2;-1;1;2;4}
=>3n+1={-4;-2;-1;1;2;4}
=>3n={-5;-3;-2;0;1;4}
=>n={5/3;-1;-2/3;0;1/3;1}
Ma n thuoc N
Vay n={-1;0;1}
lik e nhe