cho α ϵ(π/2,3π/4),tanα/cotα=√5 -1/√5 +1.tính p=sin2αtanα+cos2αcotα+sin2α.
K
Khách
Hãy nhập câu hỏi của bạn vào đây, nếu là tài khoản VIP, bạn sẽ được ưu tiên trả lời.
Những câu hỏi liên quan
CM
8 tháng 3 2019
Chọn C.
Ta có tan α – cotα = 1
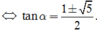
Do 
suy ra tanα < 0 nên
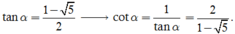
Thay
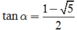và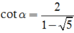 
vào P ta được
DN
1
18 tháng 1 2022
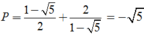
Vì \(\dfrac{\pi}{2}< \alpha< \pi\) \(\Rightarrow\) cos \(\alpha\) < 0
\(\Rightarrow\) cos \(\alpha\) = \(-\sqrt{1-sin^2\alpha}\) = \(-\dfrac{2\sqrt{2}}{3}\)
\(\Rightarrow\) tan \(\alpha\) = \(\dfrac{sin\alpha}{cos\alpha}=\dfrac{-\sqrt{2}}{4}\)
\(\Rightarrow\) cot \(\alpha\) = \(\dfrac{1}{tan\alpha}\) = \(-2\sqrt{2}\)
Chúc bn học tốt!
CM
28 tháng 7 2017
Ta có
1 cos 2 α = 1 + tan 2 α = 1 + 4 = 5
Vì π < α < 3 π 2 nên cos α < 0
Suy ra cos α = 1 5
Khi đó
M = sin 2 α + sin α + π 2 + sin 5 π 2 - 2 α
= sin 2 α + cos α + cos 2 α = sin 2 α + cos α + 2 cos 2 α - 1 = cos 2 α + cos α = 1 5 - 1 5 = 1 - 5 5
Đáp án C
PT
1
CM
29 tháng 6 2018
Chọn B.
Ta có P = tan3α + cot3α = (tanα + cotα) 3 - 3tanα.cotα( tanα + cotα)
= 53 - 3.5 = 110
TS
1
6 tháng 7 2023
3/4pi<a<pi
=>sin a>0; cosa<0
sin2a=-4/5
=>2*sina*cosa=-4/5
=>sina*cosa=-2/5
(sina-cosa)^2=sin^2a+cos^2a-2*sina*cosa=1+4/5=9/5
=>sin a-cosa=3/căn 5
21 tháng 10 2021
a: \(\cos\alpha=\dfrac{1}{2}\)
\(\tan\alpha=\sqrt{3}\)
\(\cot\alpha=\dfrac{\sqrt{3}}{3}\)
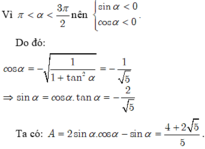
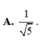
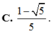
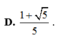
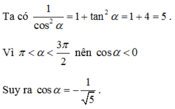
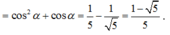
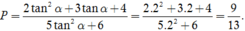
Do \(\alpha\in\left(\frac{\pi}{2};\frac{3\pi}{4}\right)\Rightarrow sin\alpha>0;cos\alpha< 0;tan\alpha< 0\)
\(\frac{tana}{cota}=\frac{\sqrt{5}-1}{\sqrt{5}+1}\Leftrightarrow tan^2a=\frac{\sqrt{5}-1}{\sqrt{5}+1}=\frac{\left(\sqrt{5}-1\right)^2}{4}\Rightarrow tana=\frac{1-\sqrt{5}}{2}\Rightarrow cota=\frac{-1-\sqrt{5}}{2}\)
\(1+tan^2a=\frac{1}{cos^2a}\Rightarrow cos^2a=\frac{1}{1+tan^2a}=\frac{5+\sqrt{5}}{10}\)
\(\Rightarrow sin^2a=1-cos^2a=\frac{5-\sqrt{5}}{10}\)
\(sin2a=2sina.cosa=2tana.cos^2a=-\frac{2\sqrt{5}}{5}\)
Thay vào ta được:
\(P=...\)
Bạn tự thay số và bấm máy