\(\dfrac{1}{\sqrt{x-1}-\sqrt{x}}+\dfrac{1}{\sqrt{x-1}+\sqrt{x}}+\dfrac{\sqrt{x^3}-x}{\sqrt{x-1}}\)giúp mik bài này với mn ơi,mik cảm ơn nhiều lắm!!!!
K
Khách
Hãy nhập câu hỏi của bạn vào đây, nếu là tài khoản VIP, bạn sẽ được ưu tiên trả lời.
Những câu hỏi liên quan
9 tháng 3 2022
\(=\dfrac{\sqrt{x}+1}{\sqrt{x}\left(\sqrt{x}-1\right)}\cdot\dfrac{\left(\sqrt{x}-1\right)^2}{\sqrt{x}+1}=\dfrac{\sqrt{x}-1}{\sqrt{x}}\)
KV
3 tháng 6 2023
a) \(P=\left(3-\dfrac{3}{\sqrt{x}-1}\right):\left(\dfrac{x+2}{x+\sqrt{x}-2}-\dfrac{\sqrt{x}}{\sqrt{x}+2}\right)\)
\(=\left(\dfrac{3\left(\sqrt{x}-1\right)-3}{\sqrt{x}-1}\right):\left[\dfrac{x+2}{\left(\sqrt{x}-1\right)\left(\sqrt{x+2}\right)}-\dfrac{\sqrt{x}}{\sqrt{x}+2}\right]\)
\(=\dfrac{3\sqrt{x}-3-3}{\sqrt{x}-1}:\dfrac{x+2-\sqrt{x}\left(\sqrt{x}-1\right)}{\left(\sqrt{x}-1\right)\left(\sqrt{x}+2\right)}\)
\(=\dfrac{3\sqrt{x}-6}{\sqrt{x}-1}:\dfrac{x+2-x+\sqrt{x}}{\left(\sqrt{x}-1\right)\left(\sqrt{x}+2\right)}\)
\(=\dfrac{3\sqrt{x}-6}{\sqrt{x}-1}:\dfrac{\sqrt{x}+2}{\left(\sqrt{x}-1\right)\left(\sqrt{x}+2\right)}\)
\(=\dfrac{3\sqrt{x}-6}{\sqrt{x}-1}:\dfrac{1}{\sqrt{x}-1}\)
\(=\dfrac{3\sqrt{x}-6}{\sqrt{x}-1}.\left(\sqrt{x}-1\right)\)
\(=3\sqrt{x}-6\)
b) \(P=\dfrac{4\sqrt{x}-1}{\sqrt{x}}\)
\(\Leftrightarrow3\sqrt{x}-6=\dfrac{4\sqrt{x}-1}{\sqrt{x}}\) (1)
ĐKXĐ: \(x>0\)
\(\left(1\right)\Leftrightarrow3x-6\sqrt{x}=4\sqrt{x}-1\)
\(\Leftrightarrow3x-6\sqrt{x}-4\sqrt{x}+1=0\)
\(\Leftrightarrow3x-10\sqrt{x}+1=0\) (2)
Đặt \(t=\sqrt{x}\ge0\)
\(\left(2\right)\Leftrightarrow3t^2-10t+1=0\)
\(\Delta'=25-4=22\)
Phương trình có hai nghiệm phân biệt:
\(t_1=\dfrac{5+\sqrt{22}}{3}\) (nhận)
\(t_2=\dfrac{5-\sqrt{22}}{3}\) (nhận)
Với \(t=\dfrac{5+\sqrt{22}}{3}\) \(\Leftrightarrow\sqrt{x}=\dfrac{5+\sqrt{22}}{3}\Leftrightarrow x=\dfrac{47+10\sqrt{22}}{9}\) (nhận)
Với \(t=\dfrac{5-\sqrt{22}}{3}\Leftrightarrow\sqrt{x}=\dfrac{5-\sqrt{22}}{3}\Leftrightarrow x=\dfrac{47-10\sqrt{22}}{9}\) (nhận)
Vậy \(x=\dfrac{47+10\sqrt{22}}{9};x=\dfrac{47-10\sqrt{22}}{9}\) thì \(P=\dfrac{4\sqrt{x}-1}{\sqrt{x}}\)
2 tháng 6 2023
a: \(P=\dfrac{3\sqrt{x}-3-3}{\sqrt{x}-1}:\dfrac{x+2-x+\sqrt{x}}{\left(\sqrt{x}+2\right)\left(\sqrt{x}-1\right)}\)
\(=\dfrac{3\left(\sqrt{x}-2\right)\left(\sqrt{x}+2\right)}{\sqrt{x}+2}=3\sqrt{x}-6\)
b: P=(4căn x-1)/căn x
=>3x-6căn x-4căn x+1=0
=>3x-10căn x+1=0
=>x=(47+10căn 22)/9 hoặc x=(47-10căn 22)/9
AT
1 tháng 8 2018
rút gọn hở bạn?
đkxđ: x>0 ; x≠1
\(S=\left(\dfrac{x\sqrt{x}-1}{x-\sqrt{x}}-\dfrac{x\sqrt{x}+1}{x+\sqrt{x}}\right)+\left(x-\dfrac{1}{\sqrt{x}}\right)\left(\dfrac{\sqrt{x}+1}{\sqrt{x}-1}+\dfrac{\sqrt{x}-1}{\sqrt{x}+1}\right)\)
\(=\left(\dfrac{\left(\sqrt{x}-1\right)\left(x+\sqrt{x}+1\right)}{\sqrt{x}\left(\sqrt{x}-1\right)}-\dfrac{\left(\sqrt{x}+1\right)\left(x-\sqrt{x}+1\right)}{\sqrt{x}\left(\sqrt{x}+1\right)}\right)+\dfrac{x-1}{\sqrt{x}}\left(\dfrac{\left(\sqrt{x}+1\right)^2-\left(\sqrt{x}-1\right)^2}{x-1}\right)\)
\(=\dfrac{x+\sqrt{x}+1-x+\sqrt{x}-1}{\sqrt{x}}+\dfrac{x-1}{\sqrt{x}}\cdot\dfrac{\left(\sqrt{x}+1-\sqrt{x}+1\right)\left(\sqrt{x}+1+\sqrt{x}-1\right)}{x-1}\)
\(\dfrac{2\sqrt{x}}{\sqrt{x}}+\dfrac{2\cdot2\sqrt{x}}{\sqrt{x}}=\dfrac{6\sqrt{x}}{\sqrt{x}}=6\)
HD
26 tháng 7 2021
Bài 2
b, `\sqrt{3x^2}=x+2` ĐKXĐ : `x>=0`
`=>(\sqrt{3x^2})^2=(x+2)^2`
`=>3x^2=x^2+4x+4`
`=>3x^2-x^2-4x-4=0`
`=>2x^2-4x-4=0`
`=>x^2-2x-2=0`
`=>(x^2-2x+1)-3=0`
`=>(x-1)^2=3`
`=>(x-1)^2=(\pm \sqrt{3})^2`
`=>` $\left[\begin{matrix} x-1=\sqrt{3}\\ x-1=-\sqrt{3}\end{matrix}\right.$
`=>` $\left[\begin{matrix} x=1+\sqrt{3}\\ x=1-\sqrt{3}\end{matrix}\right.$
Vậy `S={1+\sqrt{3};1-\sqrt{3}}`
5 tháng 8 2021
a) ĐKXĐ: \(\left\{{}\begin{matrix}x\ge0\\x\ne1\end{matrix}\right.\)
b) Ta có: \(B=\dfrac{1}{2\sqrt{x}-2}-\dfrac{1}{2\sqrt{x}+2}+\dfrac{\sqrt{x}}{1-x}\)
\(=\dfrac{\sqrt{x}+1-\sqrt{x}+1-2\sqrt{x}}{2\left(\sqrt{x}-1\right)\left(\sqrt{x}+1\right)}\)
\(=\dfrac{-1}{\sqrt{x}+1}\)
Thay x=3 vào B, ta được:
\(B=\dfrac{-1}{\sqrt{3}+1}=\dfrac{-\sqrt{3}+1}{2}\)
NV
Nguyễn Việt Lâm
Giáo viên
18 tháng 8 2021
1.
Điều kiện xác định của căn thức: \(\left[{}\begin{matrix}x\ge3\\x\le-3\end{matrix}\right.\)
\(\lim\limits_{x\rightarrow+\infty}\dfrac{\sqrt{x^2+1}-x}{\sqrt{x^2-9}-4}=\dfrac{1-1}{1}=0\Rightarrow y=0\) là 1 TCN
\(\lim\limits_{x\rightarrow-\infty}\dfrac{\sqrt{x^2+1}-x}{\sqrt{x^2-9}-4}=\dfrac{-1-1}{-1}=2\Rightarrow y=2\) là 1 TCN
\(\lim\limits_{x\rightarrow-5}\dfrac{\sqrt{x^2+1}-x}{\sqrt{x^2-9}-4}=\dfrac{\sqrt{26}+5}{0}=+\infty\Rightarrow x=-5\) là 1 TCĐ
\(\lim\limits_{x\rightarrow5}\dfrac{\sqrt{x^2+1}-x}{\sqrt{x^2-9}-4}=\dfrac{\sqrt{26}-5}{0}=+\infty\Rightarrow x=5\) là 1 TCĐ
Hàm có 4 tiệm cận
NV
Nguyễn Việt Lâm
Giáo viên
18 tháng 8 2021
2.
Căn thức của hàm luôn xác định
Ta có:
\(\lim\limits_{x\rightarrow2}\dfrac{2x-1-\sqrt{x^2+x+3}}{x^2-5x+6}=\lim\limits_{x\rightarrow2}\dfrac{\left(2x-1\right)^2-\left(x^2+x+3\right)}{\left(x-2\right)\left(x-3\right)\left(2x-1+\sqrt{x^2+x+3}\right)}\)
\(=\lim\limits_{x\rightarrow2}\dfrac{\left(x-2\right)\left(3x+1\right)}{\left(x-2\right)\left(x-3\right)\left(2x-1+\sqrt{x^2+x+3}\right)}\)
\(=\lim\limits_{x\rightarrow2}\dfrac{3x+1}{\left(x-3\right)\left(2x-1+\sqrt{x^2+x+3}\right)}=\dfrac{-7}{6}\) hữu hạn
\(\Rightarrow x=2\) ko phải TCĐ
\(\lim\limits_{x\rightarrow3}\dfrac{2x-1-\sqrt{x^2+x+3}}{x^2-5x+6}=\dfrac{5-\sqrt{15}}{0}=+\infty\)
\(\Rightarrow x=3\) là tiệm cận đứng duy nhất
30 tháng 7 2018
\(A=\left(\dfrac{2\sqrt{x}+x+1}{\sqrt{x}+1}\right)\left(1-\dfrac{x-\sqrt{x}}{\sqrt{x}-1}\right):\left(1-\sqrt{x}\right)\)
(ĐKXĐ: x\(\ge\) 0 ; x \(\ne\) 1 )
\(=\dfrac{\left(\sqrt{x}+1\right)^2}{\sqrt{x}+1}\left(1-\dfrac{\sqrt{x}\left(\sqrt{x}-1\right)}{\sqrt{x}-1}\right):\left(1-\sqrt{x}\right)\)
\(=\left(\sqrt{x}+1\right)\left(1-\sqrt{x}\right):\left(1-\sqrt{x}\right)\)
\(=\sqrt{x}+1\)
AT
30 tháng 7 2018
\(A=\left(\dfrac{2\sqrt{x}+x+1}{\sqrt{x}+1}\right)\left(1-\dfrac{x-\sqrt{x}}{\sqrt{x}-1}\right):\left(1-\sqrt{x}\right)=\left(\dfrac{\left(\sqrt{x}+1\right)^2}{\sqrt{x}+1}\right)\left(1-\dfrac{\sqrt{x}\left(\sqrt{x}-1\right)}{\sqrt{x}-1}\right):\left(1-\sqrt{x}\right)=\left(\sqrt{x}+1\right)\left(1-\sqrt{x}\right)\left(1-\sqrt{x}\right)=\left(1-x\right)\left(1-\sqrt{x}\right)=1-\sqrt{x}-x+x\sqrt{x}=x\sqrt{x}-x-\sqrt{x}+1\)
23 tháng 6 2021
`1/((sqrtx-1)(sqrtx+2))-1/((sqrtx-1)(3-sqrtx))`
`=1/((sqrtx-1)(sqrtx+2))+1/((sqrtx-1)(sqrtx-3))`
`=(sqrtx-3+sqrtx+2)/((sqrtx-1)(sqrtx+2)(sqrtx-3))`
`=(2sqrtx-1)/((sqrtx-1)(sqrtx+2)(sqrtx-3))`
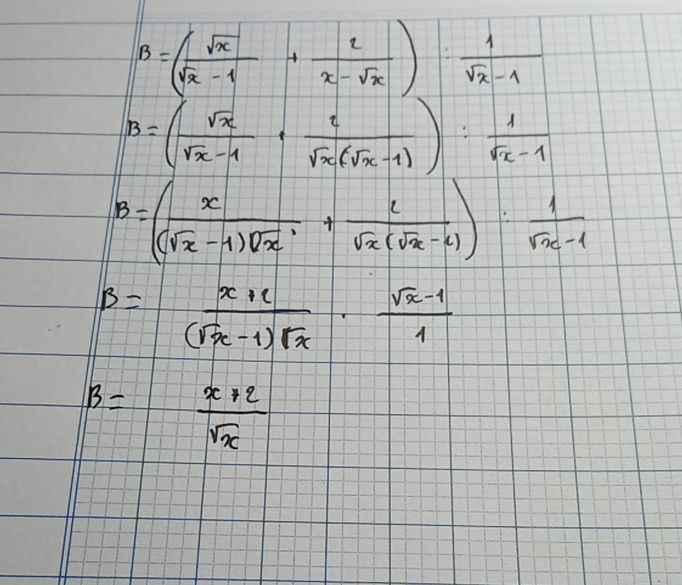
\(\dfrac{1}{\sqrt{x-1}-\sqrt{x}}+\dfrac{1}{\sqrt{x-1}+\sqrt{x}}+\dfrac{\sqrt{x^3}-x}{\sqrt{x-1}}\)
\(\Leftrightarrow-\left(\sqrt{x-1}+\sqrt{x}\right)-\left(\sqrt{x-1}-\sqrt{x}\right)+\dfrac{x\sqrt{x}-x}{\sqrt{x-1}}\)
\(\Leftrightarrow-\sqrt{x-1}-\sqrt{x}-\sqrt{x-1}+\sqrt{x}+\dfrac{x\sqrt{x}-x}{\sqrt{x-1}}\)
\(\Leftrightarrow-2\sqrt{x-1}+\dfrac{x\sqrt{x}-x}{\sqrt{x-1}}\)
\(\Leftrightarrow\dfrac{-2\left(x-1\right)+x\sqrt{x}-x}{\sqrt{x-1}}\)
\(\Leftrightarrow\dfrac{-2x+2+x\sqrt{x}-x}{\sqrt{x-1}}\)
\(\Leftrightarrow\dfrac{-3x+2+x\sqrt{x}}{\sqrt{x-1}}\)
À mình quên ĐKXĐ rồi bạn bổ sung vô nha :v