

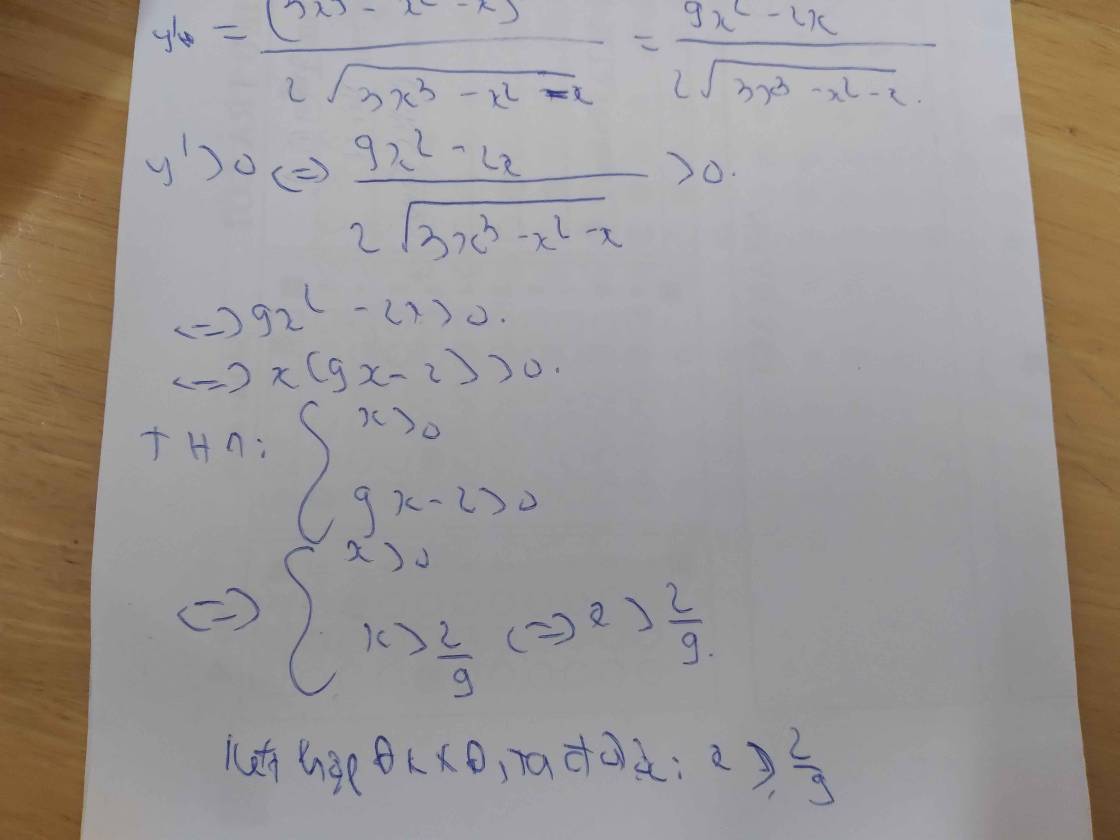

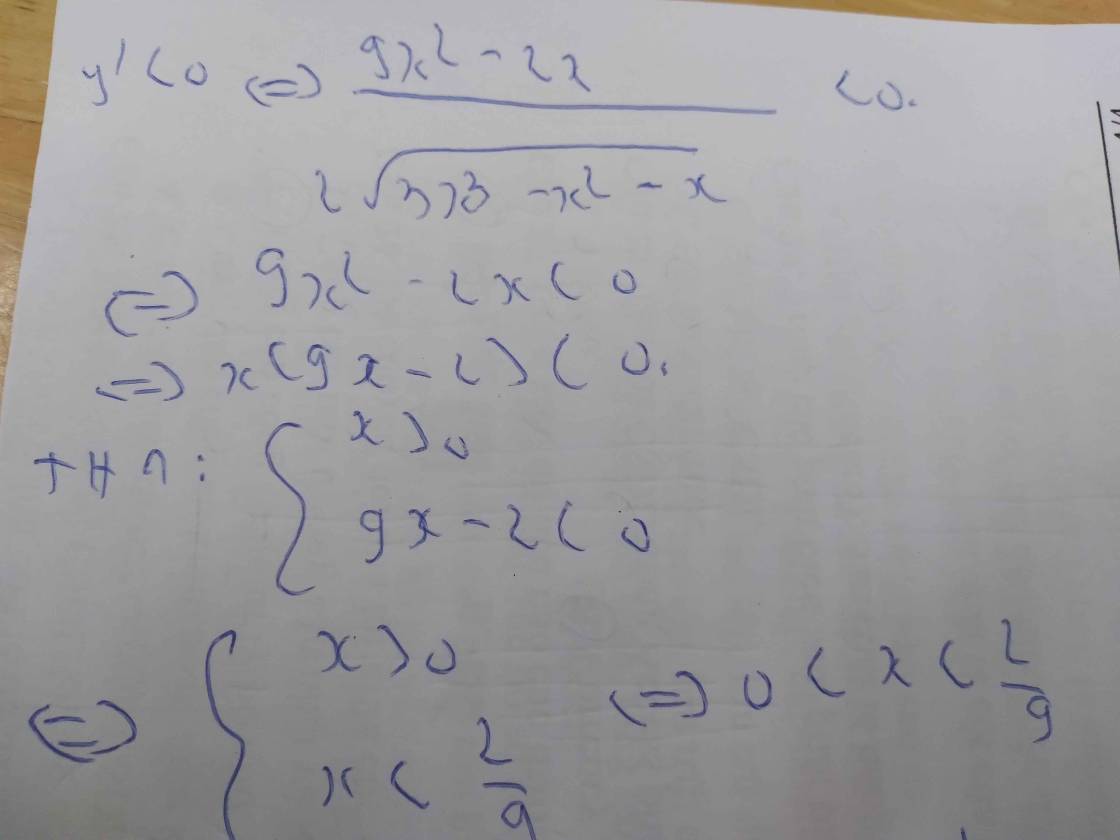
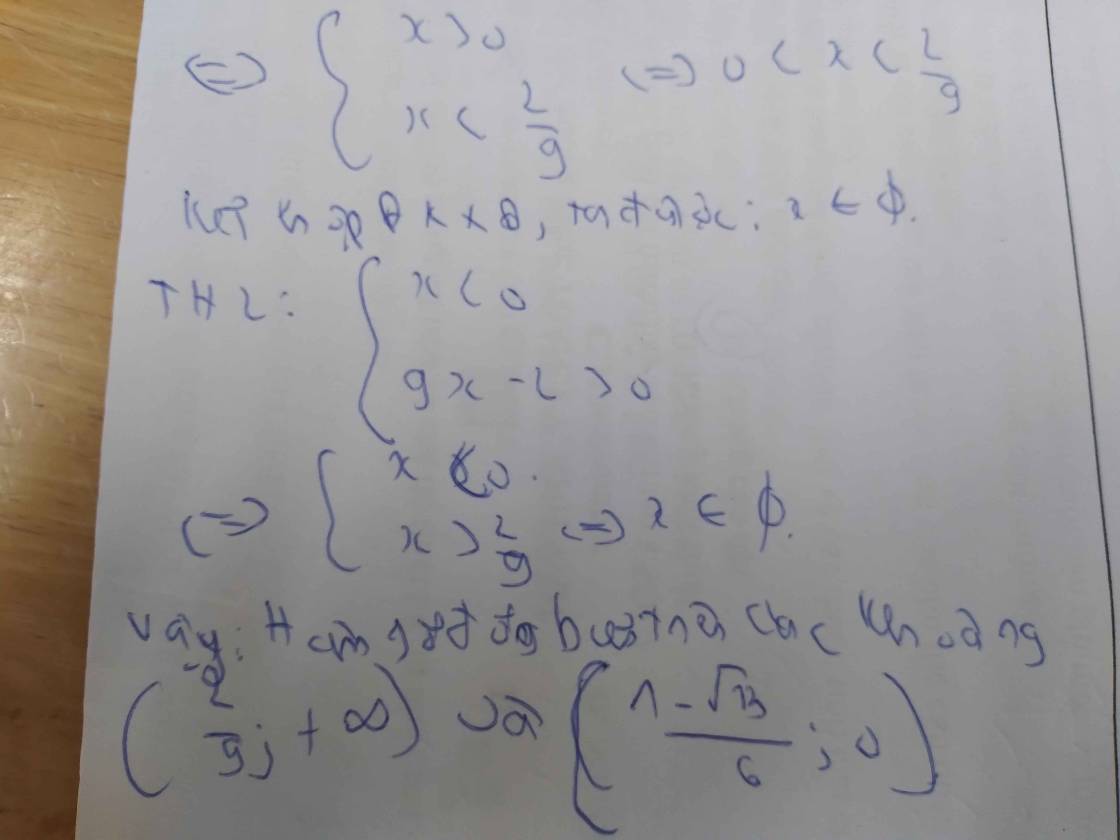





Hãy nhập câu hỏi của bạn vào đây, nếu là tài khoản VIP, bạn sẽ được ưu tiên trả lời.


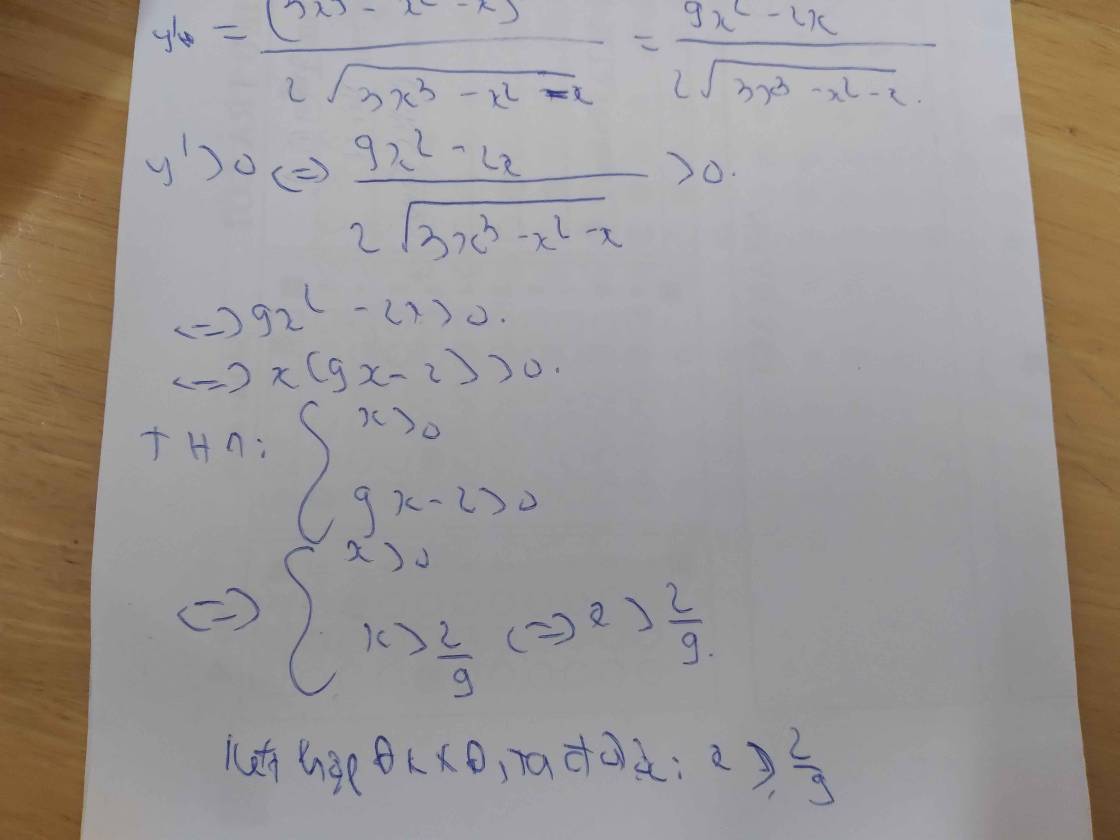

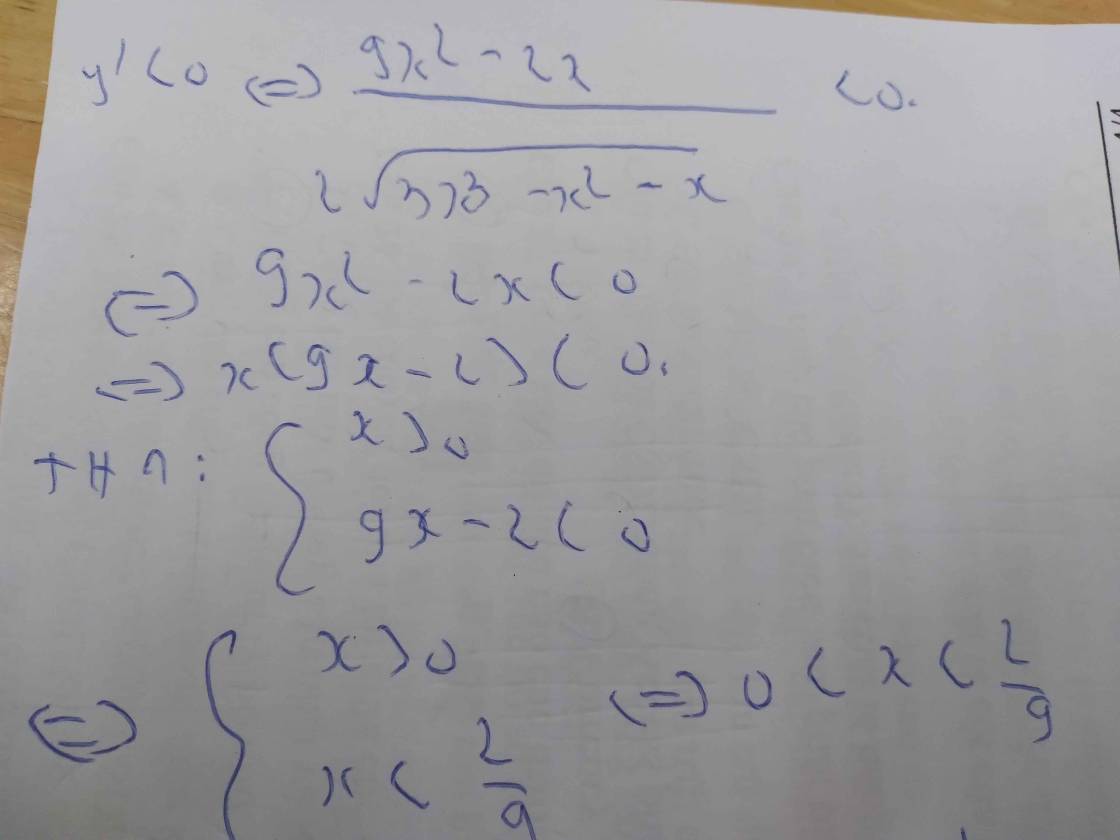
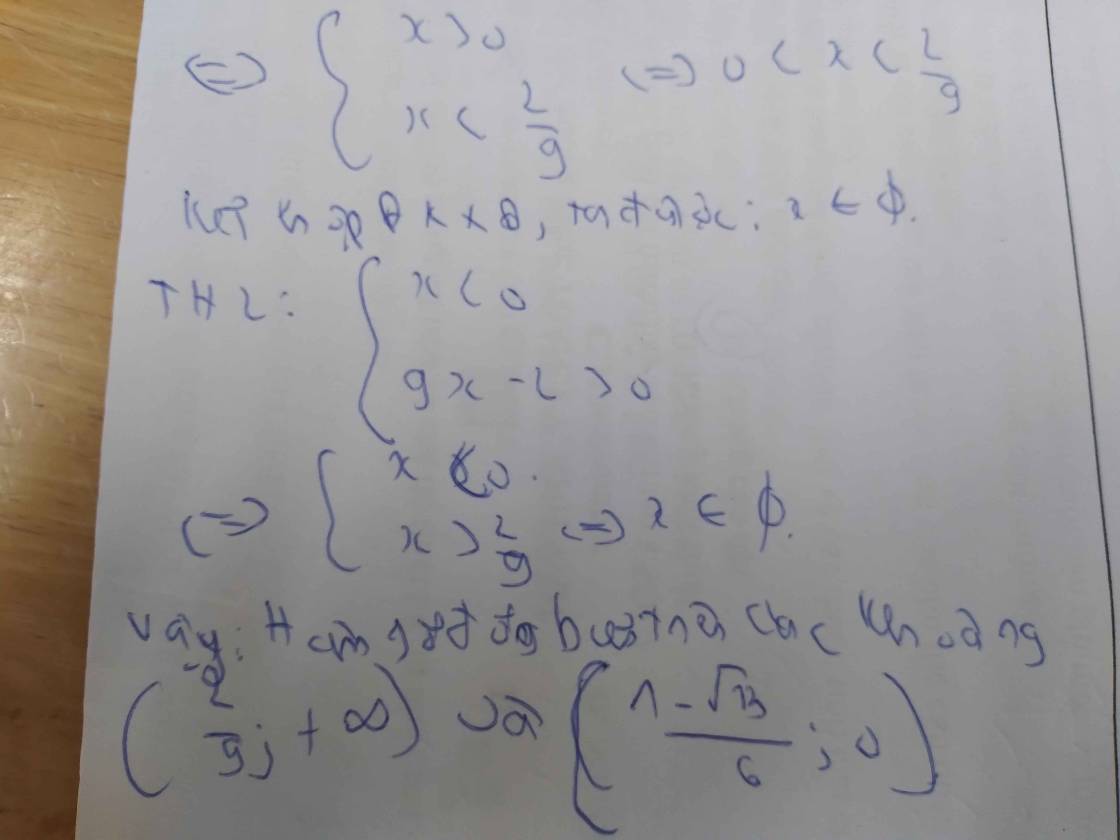






a: \(y=\left(x^2-1\right)^2\)
=>\(y'=2\left(x^2-1\right)'\left(x^2-1\right)\)
\(=4x\left(x^2-1\right)\)
Đặt y'>0
=>\(x\left(x^2-1\right)>0\)
TH1: \(\left\{{}\begin{matrix}x>0\\x^2-1>0\end{matrix}\right.\)
=>\(\left\{{}\begin{matrix}x>0\\x^2>1\end{matrix}\right.\Leftrightarrow\left\{{}\begin{matrix}x>0\\\left[{}\begin{matrix}x>1\\x< -1\end{matrix}\right.\end{matrix}\right.\)
=>\(x>1\)
TH2: \(\left\{{}\begin{matrix}x< 0\\x^2-1< 0\end{matrix}\right.\)
=>\(\left\{{}\begin{matrix}x< 0\\-1< x< 1\end{matrix}\right.\Leftrightarrow-1< x< 0\)
Đặt y'<0
=>\(x\left(x^2-1\right)< 0\)
TH1: \(\left\{{}\begin{matrix}x>0\\x^2-1< 0\end{matrix}\right.\)
=>\(\left\{{}\begin{matrix}x>0\\x^2< 1\end{matrix}\right.\Leftrightarrow\left\{{}\begin{matrix}x>0\\-1< x< 1\end{matrix}\right.\)
=>0<x<1
TH2: \(\left\{{}\begin{matrix}x< 0\\x^2-1>0\end{matrix}\right.\)
=>\(\left\{{}\begin{matrix}x< 0\\x^2>1\end{matrix}\right.\Leftrightarrow\left\{{}\begin{matrix}x< 0\\\left[{}\begin{matrix}x>1\\x< -1\end{matrix}\right.\end{matrix}\right.\)
=>x<-1
Vậy: Hàm số đồng biến trên các khoảng \(\left(1;+\infty\right);\left(-1;0\right)\)
Hàm số nghịch biến trên các khoảng (0;1) và \(\left(-\infty;-1\right)\)
b: \(y=\left(3x+4\right)^3\)
=>\(y'=3\left(3x+4\right)'\left(3x+4\right)^2\)
\(\Leftrightarrow y'=9\left(3x+4\right)^2>=0\forall x\)
=>Hàm số luôn đồng biến trên R
c: \(y=\left(x+3\right)^2\left(x-1\right)\)
=>\(y=\left(x^2+6x+9\right)\left(x-1\right)\)
=>\(y'=\left(x^2+6x+9\right)'\left(x-1\right)+\left(x^2+6x+9\right)\left(x-1\right)'\)
=>\(y'=\left(2x+6\right)\left(x-1\right)+x^2+6x+9\)
=>\(y'=2x^2-2x+6x-6+x^2+6x+9\)
=>\(y'=3x^2-2x+3\)
\(\Leftrightarrow y'=3\left(x^2-\dfrac{2}{3}x+1\right)\)
=>\(y'=3\left(x^2-2\cdot x\cdot\dfrac{1}{3}+\dfrac{1}{9}+\dfrac{8}{9}\right)\)
=>\(y'=3\left(x-\dfrac{1}{3}\right)^2+\dfrac{8}{3}>=\dfrac{8}{3}>0\forall x\)
=>Hàm số luôn đồng biến trên R
d: \(y=\left(2x+2\right)\left(x^3-1\right)\)
=>\(y'=\left(2x+2\right)'\left(x^3-1\right)+\left(2x+2\right)\left(x^3-1\right)'\)
\(=2\left(x^3-1\right)+3x^2\left(2x+2\right)\)
\(=2x^3-2+6x^3+6x^2\)
\(=8x^3+6x^2-2\)
Đặt y'>0
=>\(8x^3+6x^2-2>0\)
=>\(x>0,46\)
Đặt y'<0
=>\(8x^3+6x^2-2< 0\)
=>\(x< 0,46\)
Vậy: Hàm số đồng biến trên khoảng tầm \(\left(0,46;+\infty\right)\)
Hàm số nghịch biến trên khoảng tầm \(\left(-\infty;0,46\right)\)

a: \(y'=\left(x^2\right)'+\left(3x\right)'-\left(6x^6\right)'+\left(\dfrac{2x-3}{x-1}\right)'\)
\(=2x+3-6\cdot6x^5+\dfrac{\left(2x-3\right)'\left(x-1\right)-\left(2x-3\right)\left(x-1\right)'}{\left(x-1\right)^2}\)
\(=-36x^5+2x+3+\dfrac{2\left(x-1\right)-2x+3}{\left(x-1\right)^2}\)
\(=-36x^5+2x+3+\dfrac{1}{\left(x-1\right)^2}\)
b: \(\left(\sqrt{2x^2-3x+1}\right)'=\dfrac{\left(2x^2-3x+1\right)'}{2\sqrt{2x^2-3x+1}}\)
\(=\dfrac{4x-3}{2\sqrt{2x^2-3x+1}}\)
\(y'=3\cdot2x-4+\dfrac{4x-3}{2\sqrt{2x^2-3x+1}}\)
\(=6x-4+\dfrac{4x-3}{2\sqrt{2x^2-3x+1}}\)
c: \(\left(\sqrt{4x^2-3x+1}\right)'=\dfrac{\left(4x^2-3x+1\right)'}{2\sqrt{4x^2-3x+1}}\)
\(=\dfrac{8x-3}{2\sqrt{4x^2-3x+1}}\)
\(y'=\left(\sqrt{4x^2-3x+1}\right)'-4'=\dfrac{8x-3}{2\sqrt{4x^2-3x+1}}\)

a/ \(y=\left(x^3-3x\right)^{\dfrac{3}{2}}\Rightarrow y'=\dfrac{3}{2}\left(x^3-3x\right)^{\dfrac{1}{2}}\left(x^3-3x\right)'=\dfrac{3}{2}\left(3x^2-3\right)\sqrt{x^3-3x}\)
b/ \(y'=5\left(\sqrt{x^3+1}-x^2+2\right)^4\left(\sqrt{x^3+1}-x^2+2\right)'=5\left(\sqrt{x^3+1}-x^2+2\right)^4\left(\dfrac{3x^2}{\sqrt{x^3+1}}-2x\right)\)c/
\(y'=14\left(x^6+2x-3\right)^6\left(x^6+2x-3\right)'=14\left(x^6+2x-3\right)^6\left(6x^5+2\right)\)
d/ \(y=\left(x^3-1\right)^{-\dfrac{5}{2}}\Rightarrow y'=-\dfrac{5}{2}\left(x^3-1\right)^{-\dfrac{7}{2}}\left(x^3-1\right)'=-\dfrac{15x^2}{2\sqrt{\left(x^3-1\right)^7}}\)

a: \(\lim\limits_{x\rightarrow2^+}\dfrac{\sqrt{x-2}+1}{x^2-3x+2}=+\infty\) vì \(\left\{{}\begin{matrix}\lim\limits_{x\rightarrow2^+}\sqrt{x-2}+1=\sqrt{2-2}+1=1>0\\\lim\limits_{x\rightarrow2^+}x^2-3x+2=\lim\limits_{x\rightarrow2^+}\left(x-1\right)\left(x-2\right)=0\end{matrix}\right.\)
=>x=2 là tiệm cận đứng của đồ thị hàm số \(y=\dfrac{\sqrt{x-2}+1}{x^2-3x+2}\)
b: \(\lim\limits_{x\rightarrow-5^+}\dfrac{\sqrt{5+x}-1}{x^2+4x}=\dfrac{\sqrt{5-5}-1}{\left(-5\right)^2+4\cdot\left(-5\right)}=\dfrac{-1}{25-20}=\dfrac{-1}{5}\)
=>x=-5 không là tiệm cận đứng của đồ thị hàm số \(y=\dfrac{\sqrt{5+x}-1}{x^2+4x}\)
\(\lim\limits_{x\rightarrow\left(-4\right)^+}\dfrac{\sqrt{5+x}-1}{x^2+4x}\)
\(=\lim\limits_{x\rightarrow\left(-4\right)^+}\dfrac{5+x-1}{\left(\sqrt{5+x}+1\right)\left(x^2+4x\right)}=\lim\limits_{x\rightarrow\left(-4\right)^+}\dfrac{x+4}{\left(\sqrt{5+x}+1\right)\cdot x\left(x+4\right)}\)
\(=\lim\limits_{x\rightarrow\left(-4\right)^+}\dfrac{1}{x\left(\sqrt{5+x}+1\right)}=\dfrac{1}{\left(-4\right)\cdot\left(\sqrt{5-4}+1\right)}=\dfrac{1}{-8}=-\dfrac{1}{8}\)
=>x=-4 không là tiệm cận đứng của đồ thị hàm số \(y=\dfrac{\sqrt{5+x}-1}{x^2+4x}\)
\(\lim\limits_{x\rightarrow0^+}\dfrac{\sqrt{5+x}-1}{x^2+4x}=+\infty\) vì \(\left\{{}\begin{matrix}\lim\limits_{x\rightarrow0^+}\sqrt{5+x}-1=\sqrt{5+0}-1=\sqrt{5}-1>0\\\lim\limits_{x\rightarrow0^+}x^2+4x=0\end{matrix}\right.\)
=>Đường thẳng x=0 là tiệm cận đứng của đồ thị hàm số \(y=\dfrac{\sqrt{5+x}-1}{x^2+4x}\)
c: \(\lim\limits_{x\rightarrow0^+}\dfrac{5x+1-\sqrt{x+1}}{x^2+2x}\)
\(=\lim\limits_{x\rightarrow0^+}\dfrac{\dfrac{5x+1-x^2-2x-1}{5x+1+\sqrt{x+1}}}{x\left(x+2\right)}\)
\(=\lim\limits_{x\rightarrow0^+}\dfrac{-x^2+3x}{\left(5x+1+\sqrt{x+1}\right)\cdot x\left(x+2\right)}\)
\(=\lim\limits_{x\rightarrow0^+}\dfrac{-x\left(x-3\right)}{x\left(x+2\right)\left(5x+1+\sqrt{x+1}\right)}\)
\(=\lim\limits_{x\rightarrow0^+}\dfrac{-x+3}{\left(x+2\right)\left(5x+1+\sqrt{x+1}\right)}=\dfrac{-0+3}{\left(0+2\right)\left(5\cdot0+1+\sqrt{0+1}\right)}\)
\(=\dfrac{3}{2\cdot\left(6+1\right)}=\dfrac{3}{14}\)
=>x=0 không là tiệm cận đứng của đồ thị hàm số \(y=\dfrac{5x+1-\sqrt{x+1}}{x^2+2x}\)
\(\lim\limits_{x\rightarrow\left(-2\right)^+}\dfrac{5x+1-\sqrt{x+1}}{x^2+2x}\) không có giá trị vì khi x=-2 thì căn x+1 vô giá trị
=>Đồ thị hàm số \(y=\dfrac{5x+1-\sqrt{x+1}}{x^2+2x}\) không có tiệm cận đứng
d: \(\lim\limits_{x\rightarrow0^+}\dfrac{\sqrt{4x^2-1}+3x^2+2}{x^2-x}\) không có giá trị vì khi x=0 thì \(\sqrt{4x^2-1}\) không có giá trị
\(\lim\limits_{x\rightarrow1^+}\dfrac{\sqrt{4x^2-1}+3x^2+2}{x^2-x}\)
\(=+\infty\) vì \(\left\{{}\begin{matrix}\lim\limits_{x\rightarrow1^+}\sqrt{4x^2-1}+3x^2+2=\sqrt{4-1}+3\cdot1^2+2=5+\sqrt{3}>0\\\lim\limits_{x\rightarrow1^+}x^2-x=0\end{matrix}\right.\)
=>x=1 là tiệm cận đứng của đồ thị hàm số \(y=\dfrac{\sqrt{4x^2-1}+3x^2+2}{x^2-x}\)

a. \(y'=3sin^2x.\left(sinx\right)'=3sin^2x.cosx\)
b. \(y'=3cos^2x.\left(cosx\right)'=-3cos^2x.sinx\)
c. \(y'=cosx.cos^2x+2cosx.\left(-sinx\right).sinx=cos^3x-2cosx.sin^2x\)
d. \(y=x^{\dfrac{1}{3}}+\left(x+1\right)^{\dfrac{2}{3}}\Rightarrow y'=\dfrac{1}{3}x^{-\dfrac{2}{3}}+\dfrac{2}{3}\left(x+1\right)^{-\dfrac{1}{3}}=\dfrac{1}{3\sqrt[3]{x^2}}+\dfrac{2}{3\sqrt[3]{x+1}}\)