Hãy nhập câu hỏi của bạn vào đây, nếu là tài khoản VIP, bạn sẽ được ưu tiên trả lời.

Lời giải:
ĐKXĐ: $x>0; x\neq 4$
\(A=\frac{\sqrt{x}-2+\sqrt{x}+2}{(\sqrt{x}+2)(\sqrt{x}-2)}.\frac{\sqrt{x}-2}{\sqrt{x}}=\frac{2\sqrt{x}}{(\sqrt{x}-2)(\sqrt{x}+2)}.\frac{\sqrt{x}-2}{\sqrt{x}}=\frac{2}{\sqrt{x}+2}\)
\(B=\frac{7}{3}A=\frac{14}{3(\sqrt{x}+2)}\)
Hiển nhiên $B>0$
Với $x>0; x\neq 4\Rightarrow 3(\sqrt{x}+2)\geq 6$
$\Rightarrow B=\frac{14}{3(\sqrt{x}+2)}\leq \frac{14}{6}<3$
Vậy $0< B< 3$. $B$ nguyên $\Leftrightarrow B\in\left\{1;2\right\}$
$\Leftrightarrow \frac{14}{3(\sqrt{x}+2)}\in\left\{1;2\right\}$
$\Leftrightarrow x\in\left\{\frac{64}{9}; \frac{1}{9}\right\}$ (tm)

2.
\(x-2\sqrt{x}=\sqrt{x}(\sqrt{x}-3)+\frac{1}{4}(\sqrt{x}-3)+\frac{3}{4}(\sqrt{x}+1)\)
\(\geq \frac{3}{4}(\sqrt{x}+1)\)
\(\Rightarrow I\leq \frac{\sqrt{x}+1}{\frac{3}{4}(\sqrt{x}+1)}=\frac{4}{3}\)
Vậy $I_{\max}=\frac{4}{3}$ tại $x=9$
1. Với $x\geq \frac{1}{2}$ thì:
\(3x+\sqrt{x}+1=(\sqrt{2x}-1)(\sqrt{\frac{9}{2}x}-1)+(1+\frac{5\sqrt{2}}{2})\sqrt{x}\)
\(\geq (1+\frac{5\sqrt{2}}{2})\sqrt{x}\)
\(\Rightarrow H=\frac{\sqrt{x}}{3x+\sqrt{x}+1}\leq \frac{\sqrt{x}}{(1+\frac{5\sqrt{2}}{2})\sqrt{x}}=\frac{1}{1+\frac{5\sqrt{2}}{2}}=\frac{5\sqrt{2}-2}{23}\)
Đây chính là $H_{\max}$. Giá trị này đạt tại $x=\frac{1}{2}$

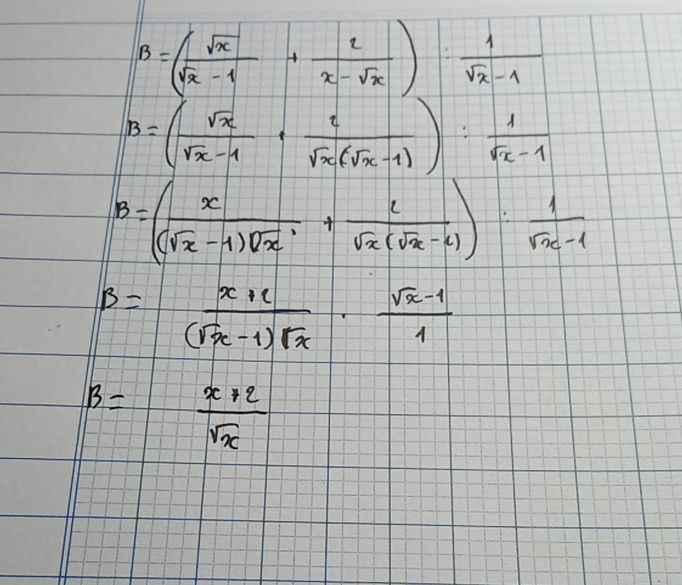
\(=\dfrac{\sqrt{x}+1}{\sqrt{x}\left(\sqrt{x}-1\right)}\cdot\dfrac{\left(\sqrt{x}-1\right)^2}{\sqrt{x}+1}=\dfrac{\sqrt{x}-1}{\sqrt{x}}\)

Bài 2:
a: ĐKXĐ: a>0 và b>0
b: \(P=\dfrac{\left(\sqrt{a}-\sqrt{b}\right)^2}{\sqrt{a}-\sqrt{b}}=\sqrt{a}-\sqrt{b}\)
c: Khi a=4 và b=1 thì P=2-1=1

Có bài ngược của bài này, bạn đăng và đã có lời giải thì chỉ cần đảo lại đáp án là được.
\(E=\sqrt{x}+\dfrac{4}{\sqrt{x}}-2=\dfrac{4\sqrt{x}}{9}+\dfrac{4}{\sqrt{x}}+\dfrac{5}{9}.\sqrt{x}-2\)
\(E\ge2\sqrt{\dfrac{16\sqrt{x}}{9\sqrt{x}}}+\dfrac{5}{9}.\sqrt{9}-2=\dfrac{7}{3}\)
\(E_{min}=\dfrac{7}{3}\) khi \(x=9\)
\(F=3\sqrt{x}+\dfrac{1}{\sqrt{x}}+1=2\sqrt{x}+\dfrac{1}{\sqrt{x}}+\sqrt{x}+1\)
\(F\ge2\sqrt{\dfrac{2\sqrt{x}}{\sqrt{x}}}+1.\sqrt{\dfrac{1}{2}}+1=\dfrac{2+5\sqrt{2}}{2}\)
\(F_{min}=\dfrac{2+5\sqrt{2}}{2}\) khi \(x=\dfrac{1}{2}\)

a.
Đặt \(\sqrt{x}+1=t\Rightarrow t\ge3\)
\(\sqrt{x}=t-1\)
\(\Rightarrow D=\dfrac{\left(t-1\right)^2-\left(t-1\right)+2}{t}=\dfrac{t^2-3t+4}{t}=t+\dfrac{4}{t}-3\)
\(D=\dfrac{4t}{9}+\dfrac{4}{t}+\dfrac{5t}{9}-3\ge2\sqrt{\dfrac{16t}{9t}}+\dfrac{5}{9}.3-3=\dfrac{4}{3}\)
\(D_{min}=\dfrac{4}{3}\) khi \(t=3\) hay \(x=4\)
b.
Đặt \(\sqrt{x}+2=t\Rightarrow t\ge4\)
\(\Rightarrow\sqrt{x}=t-2\)
\(M=\dfrac{\left(t-2\right)^2+8}{t}=\dfrac{t^2-4t+12}{t}=t+\dfrac{12}{t}-4\)
\(M=\dfrac{3t}{4}+\dfrac{12}{t}+\dfrac{1}{4}t-4\)
\(M\ge2\sqrt{\dfrac{36t}{4t}}+\dfrac{1}{4}.4-4=3\)
\(M_{min}=3\) khi \(t=4\) hay \(x=4\)