Hãy nhập câu hỏi của bạn vào đây, nếu là tài khoản VIP, bạn sẽ được ưu tiên trả lời.

a) \(\sqrt{2x+7}\)
Để \(\sqrt{2x+7}\) có nghĩa\(\Leftrightarrow\)2x+7\(\ge\)0
\(\Leftrightarrow\)2x\(\ge\)-7
\(\Leftrightarrow\)x\(\ge\)\(\dfrac{-7}{2}\)
b) \(\sqrt{-3x+4}\)
Để \(\sqrt{-3x+4}\) có nghĩa \(\Leftrightarrow\)-3x+4\(\ge\)0
\(\Leftrightarrow\)-3x\(\ge\)-4
\(\Leftrightarrow\)x\(\le\)\(\dfrac{4}{3}\)
c)\(\sqrt{\dfrac{1}{-1+x}}\)
Để \(\sqrt{\dfrac{1}{-1+x}}\) có nghĩa \(\Leftrightarrow\)\(\dfrac{1}{-1+x}\ge0\)
\(\Leftrightarrow\)-1+x>0
\(\Leftrightarrow\)x>1
d) \(\sqrt{1+x^2}\)
Ta có x2+1\(\ge\)1>0;\(\forall\)x\(\in R\)
Vậy x\(\in R\)

a) \(\sqrt{x+3}+\sqrt{x^2+9}\)
Ta thấy \(x^2\ge0\Rightarrow x^2+9\ge9\Rightarrow\sqrt{x^2+9}\ge3\)(luôn xác định)
Vậy để biểu thức xác định thì \(\sqrt{x+3}\)phải xác định
\(\Rightarrow x+3\ge0\Leftrightarrow x\ge-3\)
Vậy \(ĐKXĐ:x\ge-3\)
b) \(\sqrt{\frac{x-1}{x+2}}\)
Để biểu thức trên xác định thì x - 1 và x + 2 cùng dấu
\(TH1:\hept{\begin{cases}x-1>0\\x+2>0\end{cases}}\Leftrightarrow\hept{\begin{cases}x>1\\x>-2\end{cases}}\Rightarrow x>1\)
\(TH1:\hept{\begin{cases}x-1< 0\\x+2< 0\end{cases}}\Leftrightarrow\hept{\begin{cases}x< 1\\x< -2\end{cases}}\Rightarrow x< -2\)
Vậy \(ĐKXĐ:x>1;x< -2\)

a/ đkxđ: \(x+3\ge0\Leftrightarrow x\ge-3\)
b/ \(\left\{{}\begin{matrix}4x-1\ge0\\x\ne\dfrac{1}{2}\end{matrix}\right.\Leftrightarrow\left\{{}\begin{matrix}x\ge\dfrac{1}{4}\\x\ne\dfrac{1}{2}\end{matrix}\right.\)
c/ \(2-x^2>0\Leftrightarrow x^2< 2\Leftrightarrow-\sqrt{2}< x< \sqrt{2}\)
d/ \(6-x-x^2>0\Leftrightarrow\left(x+3\right)\left(2-x\right)>0\Leftrightarrow\left(x+3\right)\left(x-2\right)< 0\Leftrightarrow-3< x< 2\)

a) Để \(\sqrt{3x-5}\) có nghĩa thì
3x - 5 \(\ge\) 0 <=> 3x \(\ge\) 5 <=> x \(\ge\) \(\dfrac{5}{3}\)
b) Để \(\sqrt{\dfrac{-3}{4-5x}}\) có nghĩa thì
\(\dfrac{-3}{4-5x}\ge0\)
Do -3 < 0 nên \(\dfrac{-3}{4-5x}< 0\)
Khi và chỉ khi 4 - 5x < 0 <=> x > \(\dfrac{4}{5}\)
c) Để \(\sqrt{x^2-5x+4}\) = \(\sqrt{\left(x^2-x\right)-\left(4x-4\right)}=\sqrt{x\left(x-1\right)-4\left(x-1\right)}=\sqrt{\left(x-1\right)\left(x-4\right)}\) có nghĩa thì
\(\left(x-1\right)\left(x-4\right)\ge0\)
Ta có bảng xét dấu :
x (x-1) (x-4) (x-1)(x-4) 1 4 0 0 0 0 - + + - - + + - +
=> x \(\le1\) Hoặc x \(\ge4\)
e) Để \(\sqrt{2x-3}\) có nghĩa thì \(2x-3\ge0< =>2x\ge3\Leftrightarrow x\ge\dfrac{3}{2}\)

\(\frac{1}{\sqrt{x-1}}\)
a) sai đề

\(9-12x+4x^2>0\)
\(\Rightarrow\left(2-2x\right)^2>0\)
\(\Rightarrow2-2x>0\)
\(\Rightarrow-2x>-2\)
\(\Rightarrow x< 1\)
Vậy để A có nghĩa thì \(x< 1\)
B) \(\sqrt{x+2\sqrt{x-1}}\ne0\)
\(x+2\sqrt{x-1}>0\)
\(\Rightarrow x-1+2\sqrt{x-1}+1>0\)
\(\Rightarrow\left(\sqrt{x-1}+1\right)^2>0\)
\(\sqrt{x-1}\ge0\Rightarrow x\ge1\)\(\)
Vậy \(x\ge1\)thì B có nghĩa
C) \(\sqrt{3x-2}.\sqrt{x-1}\ge0\)
\(\orbr{\begin{cases}3x-2\ge0\\x-1\ge0\end{cases}}\Rightarrow\orbr{\begin{cases}x\ge\frac{2}{3}\\x\ge1\end{cases}}\)
Vậy \(x\ge1\)thì C có nghĩa
a) \(\frac{1}{\sqrt{9-12x+4x^2}}=\frac{1}{\sqrt{\left(2x-3\right)^2}}=\frac{1}{2x-3}\)
để căn thức A có nghĩa \(\Rightarrow2x-3\ne0\Leftrightarrow x\ne\frac{3}{2}\)
b)\(\frac{1}{\sqrt{x+2\sqrt{x}+1}}=\frac{1}{\sqrt{\left(\sqrt{x}+1\right)^2}}=\frac{1}{\sqrt{x}+1}\)
để căn thức B có nghĩa => \(\sqrt{x}+1\ne0\) và \(x\ge0\) hay \(\sqrt{x}+1>1\Leftrightarrow x=0\)
Vậy..........
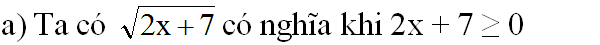
a)Ta có 2 x + 7 có nghĩa khi 2 x + 7 ≥ 0
2 x + 7 ≥ 0 ⇔ 2 x ≥ - 7 ⇔ x ≥ - 7 2
b ) - 3 x + 4 có nghĩa khi - 3 x + 4 ≥ 0
- 3 x + 4 ≥ 0 ⇔ - 3 x ≥ - 4 ⇒ x ⩽ 4 3