K
Khách
Hãy nhập câu hỏi của bạn vào đây, nếu là tài khoản VIP, bạn sẽ được ưu tiên trả lời.
Các câu hỏi dưới đây có thể giống với câu hỏi trên
UI
4 tháng 10 2019
ap dung bdt \(|a|+|b|\ge|a+b|\) voi \(a.b\ge0\)
thi \(A\ge|x-2016+2007-x|=|1|=1\)
vay GTNN cua A = 1 . Dat duoc khi \(\left(x-2016\right)\left(2017-x\right)\ge0\)
<=> \(2016\le x\le2017\)
chuc ban hoc tot
A
3
BN
8 tháng 1 2018
1/
l2x+3l=x+2(1)
ta co l2x+3l=\(\hept{\begin{cases}2x+3voix\ge\frac{-3}{2}\\-2x-3voix< \frac{-3}{2}\end{cases}}\)
TH1: neu x>= -3/2 thi (1) <=>2x+3=x+2=>x=-1(chon)
TH2: neu x<= -3/2 thi (1) <=> -2x-3=x+2=>-3x=5=>x=-5/3(chon)
2/
de A dat gtnn thi lx-2006l va l2007l dat gtnn
ma lx-2006l va l2007-xl >=0
=> gtnn cua lx-2006l=0;l2007-xl=0
=> x=2006 hoac 2007
=> gtnn A=1
2
4
4 tháng 8 2021
'THAM KHẢO
a,
Điều kiện: x+2≥0⇔x≥−2x+2≥0⇔x≥-2
|2x+3|=x+2|2x+3|=x+2
⇔[2x+3=x+22x+3=−x−2⇔[2x+3=x+22x+3=−x−2
⇔[x=−13x=−5⇔[x=−13x=−5
⇔⎡⎣x=−1(t/m)x=−53(t/m)⇔[x=−1(t/m)x=−53(t/m)
Vậy x∈{−1;−53}x∈{-1;-53}
b,
A=|x−2006|+|2007−x|≥|x−2006+2007−x|=|1|=1A=|x−2006|+|2007−x|≥|x−2006+2007−x|=|1|=1
Đẳng thức xảy ra ⇔(x−2006)(2007−x)≥0⇔(x−2006)(2007−x)≥0
⇔(x−2006)(x−2007)≤0⇔(x−2006)(x−2007)≤0
Vì x−2006>x−2007x−2006>x−2007
⇒{x−2006≥0x−2007≤0⇒{x−2006≥0x−2007≤0
⇔{x≥2006x≤2007⇔{x≥2006x≤2007
⇔2006≤x≤2007⇔2006≤x≤2007
Vậy Amin=1⇔2006≤x≤2007
TX
1
TN
21 tháng 6 2016
Áp dụng BĐT |a|+|b|>=|a+b| ta có:
\(\left|x-2006\right|+\left|2007-x\right|\ge\left|x-2006+2007-x\right|=1\)
\(\Rightarrow A\ge1\)
Dấu "=" xảy ra khi \(\hept{\begin{cases}\left|x-2006\right|=0\\\left|2007-x\right|=0\end{cases}\Leftrightarrow}\hept{\begin{cases}x=2006\\x=2007\end{cases}}\)
Vậy MinA=1<=>x=2006 hoặc x=2007
YN
0
5 tháng 2 2022
\(A=\left|x-2006\right|+\left|2007-x\right|\ge\left|x-2006+2007-x\right|=\left|1\right|=1\)
\(minA=1\Leftrightarrow\left(x-2006\right)\left(2007-x\right)\ge0\Leftrightarrow\left[{}\begin{matrix}\left\{{}\begin{matrix}x-2006\ge0\\2007-x\ge0\end{matrix}\right.\\\left\{{}\begin{matrix}x-2006\le0\\2007-x\le0\end{matrix}\right.\end{matrix}\right.\) \(\Leftrightarrow2006\le x\le2007\)
DH
2
30 tháng 1 2022
\(A=\left|x-2006\right|+\left|2007-x\right|\)
Vì \(x>2007\) nên \(2x-4013>4014-4013=1\)
\(\Rightarrow A>1\)
Vậy \(A_{min}=1\Leftrightarrow2006\le x\le2007\)
HH
0
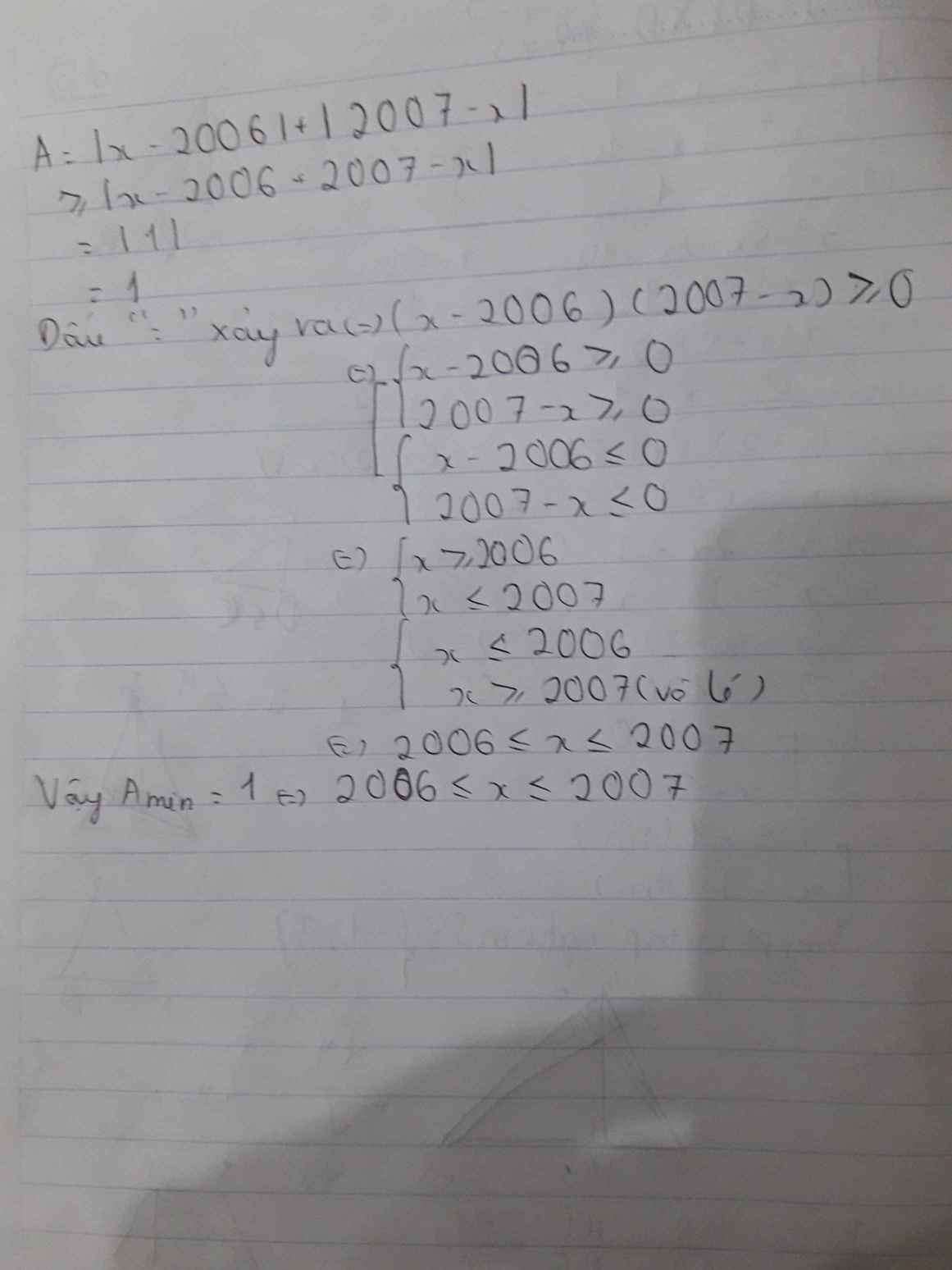
Ta có : A = |x - 2006| + |2007 - x| ≥ |x - 2006 + 2007 - x|
= |(x - x) - 2006 + 2007| = |1| = 1
Dấu "=" xảy ra khi (x - 2006)(2007 - x) ≥ 0 => 2006 ≤ x ≤ 2007
Vậy gtnn của A là 1 tại 2006 ≤ x ≤ 2007