
Hãy nhập câu hỏi của bạn vào đây, nếu là tài khoản VIP, bạn sẽ được ưu tiên trả lời.


Bài 1:
a: \(x^2+5x=x\left(x+5\right)\)
Để biểu thức này âm thì \(x\left(x+5\right)< 0\)
hay -5<x<0
b: \(3\left(2x+3\right)\left(3x-5\right)< 0\)
\(\Leftrightarrow-\dfrac{3}{2}< x< \dfrac{5}{3}\)

\(A=\left(x-4\right)^2+1\)
Ta có: \(\left(x-4\right)^2\ge0\Rightarrow\left(x-4\right)^2+1\ge1\Rightarrow A\ge1\)
\(A_{min}=1\Leftrightarrow x=4\)
\(B=\left|3x-2\right|-5\)
Ta có: \(\left|3x-2\right|\ge0\Rightarrow\left|3x-2\right|-5\ge-5\Rightarrow B\ge-5\)
\(B_{min}=-5\Leftrightarrow x=\dfrac{2}{3}\)
\(C=5-\left(2x-1\right)^4\)
Ta có: \(\left(2x-1\right)^4\ge0\forall x\Rightarrow-\left(2x-1\right)^4\le0\forall x\Rightarrow5-\left(2x-1\right)^4\le5\Rightarrow C\le5\)
\(C_{max}=5\Leftrightarrow x=\dfrac{1}{2}\)
\(D=-3\left(x-3\right)^2-\left(y-1\right)^2-2021\)
Ta có: \(\left\{{}\begin{matrix}-3\left(x-3\right)^2\le0\forall x\\-\left(y-1\right)^2\le0\forall y\end{matrix}\right.\Rightarrow-3\left(x-3\right)^2-\left(y-1\right)^2\le0\forall x,y\Rightarrow-3\left(x-3\right)^2-\left(y-1\right)^2-2021\le-2021\Rightarrow D\le-2021\)
\(D_{max}=-2021\Leftrightarrow\left\{{}\begin{matrix}x=3\\y=1\end{matrix}\right.\)
\(E=-\left|x^2-1\right|-\left(x-1\right)^2-y^2-2020\)
\(=-\left|\left(x-1\right)\left(x+1\right)\right|-\left(x-1\right)^2-y^2-2020\)
Ta có: \(\left\{{}\begin{matrix}\left|\left(x-1\right)\left(x+1\right)\right|\ge0\forall x\Rightarrow-\left|\left(x-1\right)\left(x+1\right)\right|\le0\\\left(x-1\right)^2\ge0\forall x\Rightarrow-\left(x-1\right)^2\le0\\y^2\ge0\Rightarrow-y^2\le0\end{matrix}\right.\Rightarrow E\le-2020\)
\(E_{max}=-2020\Leftrightarrow\left\{{}\begin{matrix}x=1\\y=0\end{matrix}\right.\)

1:
a: \(A=2+3\sqrt{x^2+1}>=3\cdot1+2=5\)
Dấu = xảy ra khi x=0
b: \(B=\sqrt{x+8}-7>=-7\)
Dấu = xảy ra khi x=-8

a)\(\dfrac{4^2.4^3}{2^{10}}=\dfrac{\left(2^2\right)^2.\left(2^2\right)^3}{2^{10}}=\dfrac{2^4.2^6}{2^{10}}=1\)
b)\(\dfrac{\left(0,6\right)^5}{\left(0,2\right)^6}=\dfrac{\left(0,2\right)^5.3^5}{\left(0,2\right)^5.0,2}=\dfrac{3^5}{0,2}=1215\)
c)\(\dfrac{2^7.9^3}{6^5.8^2}=\dfrac{2^7.\left(3^2\right)^3}{\left(2.3\right)^5.\left(2^3\right)^2}=\dfrac{2^7.3^6}{2^5.3^5.2^6}=\dfrac{3}{2^4}=\dfrac{3}{16}\)
d)\(\dfrac{6^3+3.6^2+3^3}{-13}\)=\(\dfrac{2^3.3^3+3.2^2.3^2+3^3}{-13}=\dfrac{3^3.\left(2^3+2^2+1\right)}{-13}=\dfrac{3^3.13}{-13}=-3^3=-27\)

Ta có \(\frac{2n+1}{2n-3}\) \(=\frac{2n-3+4}{2n-3}=1+\frac{4}{2n-3}\)
Để phân số \(\frac{2n+1}{2n-3}\) nguyên thì \(\frac{4}{2n-3}\) nguyên
=> 4 \(⋮\) 2n-3
hay 2n-3 \(\in\) Ư (4)={1;2;4;-1;-2;-4}
Ta có bảng sau
| 2n-3 | 1 | 2 | 4 | -1 | -2 | -4 |
| n | 2 | / | / | 1 | / | / |
Vậy n \(\in\) {2;1}

\(1.\frac{x-7}{2}< 0\)
\(\Leftrightarrow\frac{x-7}{2}.2< 0.2\)
\(\Leftrightarrow x-7< 0\Leftrightarrow x< 7\)
\(S=\left\{xlx< 7\right\}\)
2)\(\)Đề biểu thức sau nhân giá trị âm thì :
\(\frac{x+3}{x-5}< 0\Leftrightarrow x+3< 0\Leftrightarrow x< 3\left(Đk:x\ne5\right)\)
\(S=\left\{xlx< 3\right\}\)
3.Giá trị của x thuộc Z để biểu thức sau nhận giá trị dương:
\(x^2+x\ge0\)
\(\Leftrightarrow x\left(x+1\right)\ge0\)
\(\Leftrightarrow\orbr{\begin{cases}x\ge0\\x+1\ge0\end{cases}\Leftrightarrow\orbr{\begin{cases}x\ge0\\x\ge-1\end{cases}}}\)
\(S=\left\{xlx\ge-1\right\}\)

bài 1:
\(\left(\frac{1}{2}-2\right).\left(\frac{1}{3}-x\right)>0\)
\(\Leftrightarrow\left(-\frac{3}{2}\right)\left(\frac{1}{3}-x\right)>0\)
Để biểu thức \(\left(\frac{1}{2}-2\right)\left(\frac{1}{3}-x\right)\) nhận giá trị dương thì \(-\frac{3}{2}\)và \(\frac{1}{3}-x\)phải cùng âm
\(\Leftrightarrow\frac{1}{3}-x< 0\)
\(\Leftrightarrow x>\frac{1}{3}\)
Vậy \(x>\frac{1}{3}\)thì biểu thức\(\left(\frac{1}{2}-2\right)\left(\frac{1}{3}-x\right)\) nhận giá trị dương
bài 2:
a)Để \(\frac{x^2-2}{5x}\) nhận giá trị âm thì x2-2<0 hoặc 5x<0
+)Nếu x2-2<0
=>x2<2
=>x<\(\sqrt{2}\)
+)Nếu 5x<0
=>x<0
Vậy x<\(\sqrt{2}\)hoặc x<0 thì biểu thức \(\frac{x^2-2}{5x}\)nhận giá trị âm
b)Để E nhận giá trị âm thì \(\frac{x-2}{x-6}\)nhận giá trị âm
=>x-2<0 hoặc x-6<0
+)Nếu x-2<0
=>x<2
+)Nếu x-6<0
=>x<6
Vậy x<2 hoặc x<6 thì biểu thức E nhận giá trị âm

x2+x=x(x+1)x2+x=x(x+1)
x(x+1)x(x+1)dương ⇔⇔x>0x>0 Hoặc x0x0 x+10x+10 Hoặc x−1x−1 x0x0 hoặc \(x
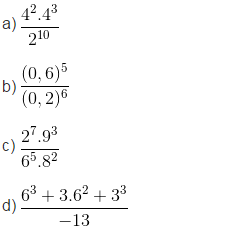
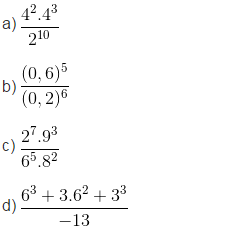
a.
\(\frac{4^2\times4^3}{2^{10}}=\frac{4^2\times4^3}{2^{2\times5}}=\frac{4^2\times4^3}{\left(2^2\right)^5}=\frac{4^5}{4^5}=1\)
b.
\(\frac{\left(0,6\right)^5}{\left(0,2\right)^6}=\frac{\left(0,2\times3\right)^5}{\left(0,2\right)^6}=\frac{\left(0,2\right)^5\times3^5}{\left(0,2\right)^6}=\frac{243}{0,2}=1215\)
c.
\(\frac{2^7\times9^3}{6^5\times8^2}=\frac{2^7\times\left(3^2\right)^3}{\left(2\times3\right)^5\times\left(2^3\right)^2}=\frac{2^7\times3^6}{2^5\times3^5\times2^6}=\frac{3}{2^4}=\frac{3}{16}\)
d.
\(\frac{6^3+3\times6^2+3^3}{-13}=\frac{\left(2\times3\right)^3+3\times\left(3\times2\right)^2+3^3}{-13}=\frac{2^3\times3^3+3\times3^2\times2^2+3^3}{-13}=\frac{8\times3^3+3^3\times4+3^3}{-13}\)\(=\frac{3^3\times\left(8+4+1\right)}{-13}=\frac{27\times13}{-13}=-27\)
a/ \(\frac{4^2.4^3}{2^{10}}=\frac{4^5}{2^{10}}=\frac{\left(2^5\right)^2}{2^{10}}=\frac{2^{10}}{2^{10}}=1\)
b/\(\frac{\left(0,6\right)^5}{\left(0,2\right)^6}=\frac{\left(0,6\right)^5}{\left(0,2\right)^5.\left(0,2\right)}=\left(\frac{0,6}{0,2}\right)^5.\frac{1}{\frac{1}{5}}=3^5.5=243.5=1215\)
c/ \(\frac{2^7.9^3}{6^5.8^2}=\frac{2^7.\left(3^2\right)^3}{\left(2.3\right)^5.\left(2^3\right)^2}=\frac{2^7.3^6}{2^5.3^5.2^6}=\frac{2^7.3.3^5}{2^7.2^4.3^5}=\frac{3}{16}\)
d/ \(\frac{6^3+3.6^2+3^3}{-13}=\frac{\left(2.3\right)^3+3.\left(2.3\right)^2+3^3}{-13}=\frac{2^3.3^3+3.2^2.3^2+3^3}{-13}=\frac{3^3.\left(2^3+2^2+1\right)}{-13}=\frac{27.13}{-13}=-27\)