Hãy nhập câu hỏi của bạn vào đây, nếu là tài khoản VIP, bạn sẽ được ưu tiên trả lời.

`2^x+8.2^{-x}<=9`
`<=>2^x+8.(2^x)^{-1}<=9`
`<=>2^x+8/(2^x)<=9`
`<=>4^x+8<=9.2^x`
`<=>(2^x)^2-9.2x^2+8<=0`
Đặt `t=2^x`
`pt<=>t^2-9t+8<=0`
`<=>(t-1)(t-8)<=0`
`<=>1<=t<=8`
`<=>1<=2^x<=8=>x in {1;2;3}`
`=>` Chọn C.3

Đặt \(\sqrt{-x^2+2x+15}=t\Rightarrow0\le t\le4\)
BPT trở thành:
\(-4t\ge-t^2+2+m\)
\(\Leftrightarrow t^2-4t-2\ge m\)
\(\Rightarrow m\le\min\limits_{\left[0;4\right]}\left(t^2-4t-2\right)\)
Xét \(f\left(t\right)=t^2-4t-2\) trên \(\left[0;4\right]\)
\(-\dfrac{b}{2a}=2\in\left[0;4\right]\)
\(f\left(0\right)=f\left(4\right)=-2\) ; \(f\left(2\right)=-6\)
\(\Rightarrow f\left(t\right)_{min}=-6\Rightarrow m\le-6\)

\(\Delta'=4-5=-1\Rightarrow\left[{}\begin{matrix}z_1=2+i\\z_2=2-i\end{matrix}\right.\)
\(\Rightarrow\left(z_1-1\right)^{2019}+\left(z_2-1\right)^{2019}=\left(i+1\right)^{2019}+\left(i-1\right)^{2019}\)
\(=\left(i+1\right)\left[\left(i+1\right)^2\right]^{1009}+\left(i-1\right)\left[\left(i-1\right)^2\right]^{1009}\)
\(=\left(i+1\right)\left(2i\right)^{1009}+\left(1-i\right)\left(-2i\right)^{1009}=\left(2i\right)^{1009}\left(i+1+i-1\right)=\left(2i\right)^{1009}.2i=\left(2i\right)^{1010}=-2^{1010}\)
=>D
P/s: Sry nó bị trôi thông báo nên toi ko để ý


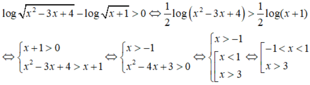
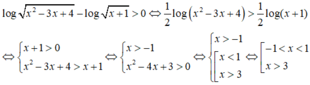

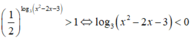
Chọn A