Hãy nhập câu hỏi của bạn vào đây, nếu là tài khoản VIP, bạn sẽ được ưu tiên trả lời.

a.
\(\Leftrightarrow m-cosx\ge0\) ; \(\forall x\)
\(\Leftrightarrow m\ge max\left(cosx\right)\)
\(\Leftrightarrow m\ge1\)
b.
\(\Leftrightarrow2sinx-m\ge0\) ; \(\forall x\)
\(\Leftrightarrow m\le2sinx\) ; \(\forall x\)
\(\Leftrightarrow m\le\min\limits_{x\in R}\left(2sinx\right)\)
\(\Leftrightarrow m\le-2\)
c.
\(\Leftrightarrow cosx+m\ne0\) ; \(\forall x\)
\(\Leftrightarrow\left[{}\begin{matrix}m>\max\limits_R\left(cosx\right)\\m< \min\limits_R\left(cosx\right)\end{matrix}\right.\)
\(\Leftrightarrow\left[{}\begin{matrix}m>1\\m< -1\end{matrix}\right.\)

cot x=-2
=>cosx=-2*sinx
\(A=\dfrac{3sinx+2\cdot sinx}{2sinx+2\cdot sinx}=\dfrac{5}{4}\)

a: ĐKXĐ: 2*sin x+1<>0
=>sin x<>-1/2
=>x<>-pi/6+k2pi và x<>7/6pi+k2pi
b: ĐKXĐ: \(\dfrac{1+cosx}{2-cosx}>=0\)
mà 1+cosx>=0
nên 2-cosx>=0
=>cosx<=2(luôn đúng)
c ĐKXĐ: tan x>0
=>kpi<x<pi/2+kpi
d: ĐKXĐ: \(2\cdot cos\left(x-\dfrac{pi}{4}\right)-1< >0\)
=>cos(x-pi/4)<>1/2
=>x-pi/4<>pi/3+k2pi và x-pi/4<>-pi/3+k2pi
=>x<>7/12pi+k2pi và x<>-pi/12+k2pi
e: ĐKXĐ: x-pi/3<>pi/2+kpi và x+pi/4<>kpi
=>x<>5/6pi+kpi và x<>kpi-pi/4
f: ĐKXĐ: cos^2x-sin^2x<>0
=>cos2x<>0
=>2x<>pi/2+kpi
=>x<>pi/4+kpi/2

1. Do \(\cos x+2>0\forall x\in R\) \(\Rightarrow\) Hàm số xác định \(\forall x\in R\)
\(y=\dfrac{\sin x+1}{\cos x+2}\)
\(\Leftrightarrow\)\(y\cos x-\sin x=1-2y\)
pt có nghiệm \(\Leftrightarrow y^2+\left(-1\right)^2\ge\left(1-2y\right)^2\)
\(\Leftrightarrow3y^2-4y\le0\)
\(\Leftrightarrow0\le y\le\dfrac{4}{3}\)
2. \(y=\dfrac{\cos x+2\sin x+3}{2\cos x-\sin x+4}\)
\(\Leftrightarrow\left(2y-1\right)\cos x-\left(y+2\right)\sin x=3-4y\)
pt có nghiệm \(\Leftrightarrow\left(2y-1\right)^2+\left(y+2\right)^2\ge\left(3-4y\right)^2\)
\(\Leftrightarrow11y^2-24y+4\le0\)
\(\Leftrightarrow\dfrac{2}{11}\le y\le2\)
kiểm tra giúp mình xem có sai sót gì không...

Xét − sin x + 2 cos x + 4 = 0
Ta thấy − 1 2 + 2 2 < 4 2 nên phương trình vô nghiệm.
Do đó − sin x + 2 cos x + 4 ≠ 0 .
Như vậy, y = 2 sin x + cos x + 3 − sin x + 2 cos x + 4
⇔ y − sin x + 2 cos x + 4 = 2 sin x + cos x + 3
⇔ sin x 2 + y + cos x 1 − 2 y + 3 − 4 y = 0
Để phương trình có nghiệm thì 2 + y 2 + 1 − 2 y 2 ≥ 3 − 4 y 2
⇔ 5 y 2 + 5 ≥ 16 y 2 − 24 y + 9
⇔ 11 y 2 − 24 y + 4 ≤ 0
⇔ 2 11 ≤ y ≤ 2
Chọn đáp án D.

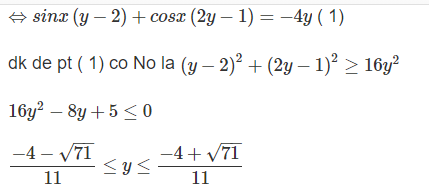


\(y=\dfrac{3sinx-cosx-4}{2sinx+cosx-3} \Leftrightarrow (2sinx+cosx-3)y=3sinx-cosx-4 \Leftrightarrow (3-2y)sinx+(y-1)cosx=4-3y \)
\(\Rightarrow (3-2y)^2+(y-1)^2 ≥ (4-3y)^2 \Leftrightarrow 5y^2−14y+10 ≥ 16−24y+9y^2 \Leftrightarrow 1 ≤ y ≤ \dfrac{3}{2}\)
Vậy hàm số không có giá trị nguyên.