K
Khách
Hãy nhập câu hỏi của bạn vào đây, nếu là tài khoản VIP, bạn sẽ được ưu tiên trả lời.
Các câu hỏi dưới đây có thể giống với câu hỏi trên
6 tháng 12 2018
\(\left(x-1\right)\left(x-4\right)\left(x-5\right)\left(x-8\right)+36=0\)
\(\Rightarrow\left(x-1\right)\left(x-8\right)\left(x-4\right)\left(x-5\right)+36=0\)
\(\Leftrightarrow\left(x^2-8x-x+8\right)\left(x^2-4x-5x+20\right)+36=0\)
\(\Rightarrow\left(x^2-9x+8\right)\left(x^2-9x+20\right)+36=0\)
\(\Rightarrow\left(x^2-9x+14-6\right)\left(x^2-9x+14+6\right)+36=0\)
\(\Rightarrow\left(x^2-9x+14\right)^2-36+36=0\)
\(\Rightarrow x^2-9x+14=0\Leftrightarrow\left(x-2\right)\left(x-7\right)=0\Leftrightarrow\left[{}\begin{matrix}x=2\\x=7\end{matrix}\right.\)
Bài 1.
MA
1
B
30 tháng 10 2020
a) \(5x\left(x+4\right)-x\left(5x+1\right)=0\)
\(\Leftrightarrow x\left[5\left(x+4\right)-5x-1\right]=0\)
\(\Leftrightarrow x\left(5x+20-5x-1\right)=0\Leftrightarrow x=0\)
b) \(3x\left(5-x\right)+4\left(x-5\right)=0\)
\(\Leftrightarrow\left(x-5\right)\left(4-3x\right)=0\)
\(\Leftrightarrow\orbr{\begin{cases}x=5\\x=\frac{4}{3}\end{cases}}\)
c) \(x\left(x-3\right)+4x-12=0\)
\(\Leftrightarrow\left(x-3\right)\left(x+4\right)=0\)
\(\Leftrightarrow\orbr{\begin{cases}x=3\\x=-4\end{cases}}\)
d) \(x^2-36=0\)
\(\Leftrightarrow\left(x+6\right)\left(x-6\right)=0\)
\(\Leftrightarrow\orbr{\begin{cases}x=6\\x=-6\end{cases}}\)
e) \(x^2+3x+1=2\)
\(\Leftrightarrow x^2+3x+1-2=0\)
\(\Leftrightarrow x^2+3x-1=0\)
\(\Leftrightarrow x^2+3x+\frac{3}{2}-\frac{5}{2}=0\)
\(\Leftrightarrow\left(x+\frac{3}{2}\right)^2-\frac{5}{2}=0\)
\(\Leftrightarrow\left(x+\frac{3}{2}+\frac{\sqrt{5}}{\sqrt{2}}\right)\left(x+\frac{3}{2}-\frac{\sqrt{5}}{\sqrt{2}}\right)=0\)
Còn lại ........... Tự lm nất nha
NT
Nguyễn Thị Thương Hoài
Giáo viên
VIP
3 tháng 10 2023
1, \(x^2\) - 9 = 0
(\(x\) - 3)(\(x\) + 3) = 0
\(\left[{}\begin{matrix}x-3=0\\x+3=0\end{matrix}\right.\)
\(\left[{}\begin{matrix}x=3\\x=-3\end{matrix}\right.\)
vậy \(x\) \(\in\) {-3; 3}
NT
Nguyễn Thị Thương Hoài
Giáo viên
VIP
3 tháng 10 2023
5, 4\(x^2\) - 36 = 0
4.(\(x^2\) - 9) = 0
\(x^2\) - 9 = 0
(\(x\) - 3)(\(x\) + 3) = 0
\(\left[{}\begin{matrix}x-3=0\\x+3=0\end{matrix}\right.\)
\(\left[{}\begin{matrix}x=3\\x=-3\end{matrix}\right.\)
Vậy \(x\) \(\in\) {-3; 3}
15 tháng 8 2018
\(1,\left(x-4\right)^2-36=0\)
\(\Leftrightarrow\left(x-4-6\right)\left(x-4+6\right)=0\)
\(\Leftrightarrow\left(x-10\right)\left(x+2\right)=0\)
\(\Leftrightarrow\left[{}\begin{matrix}x=10\\x=-2\end{matrix}\right.\)
\(2,x^2-25-\left(x+5\right)^2\)
\(=\left(x-5\right)\left(x+5\right)-\left(x+5\right)^2\)
\(=\left(x+5\right)\left(x-5-x-5\right)\)
\(=-10\left(x+5\right)\)
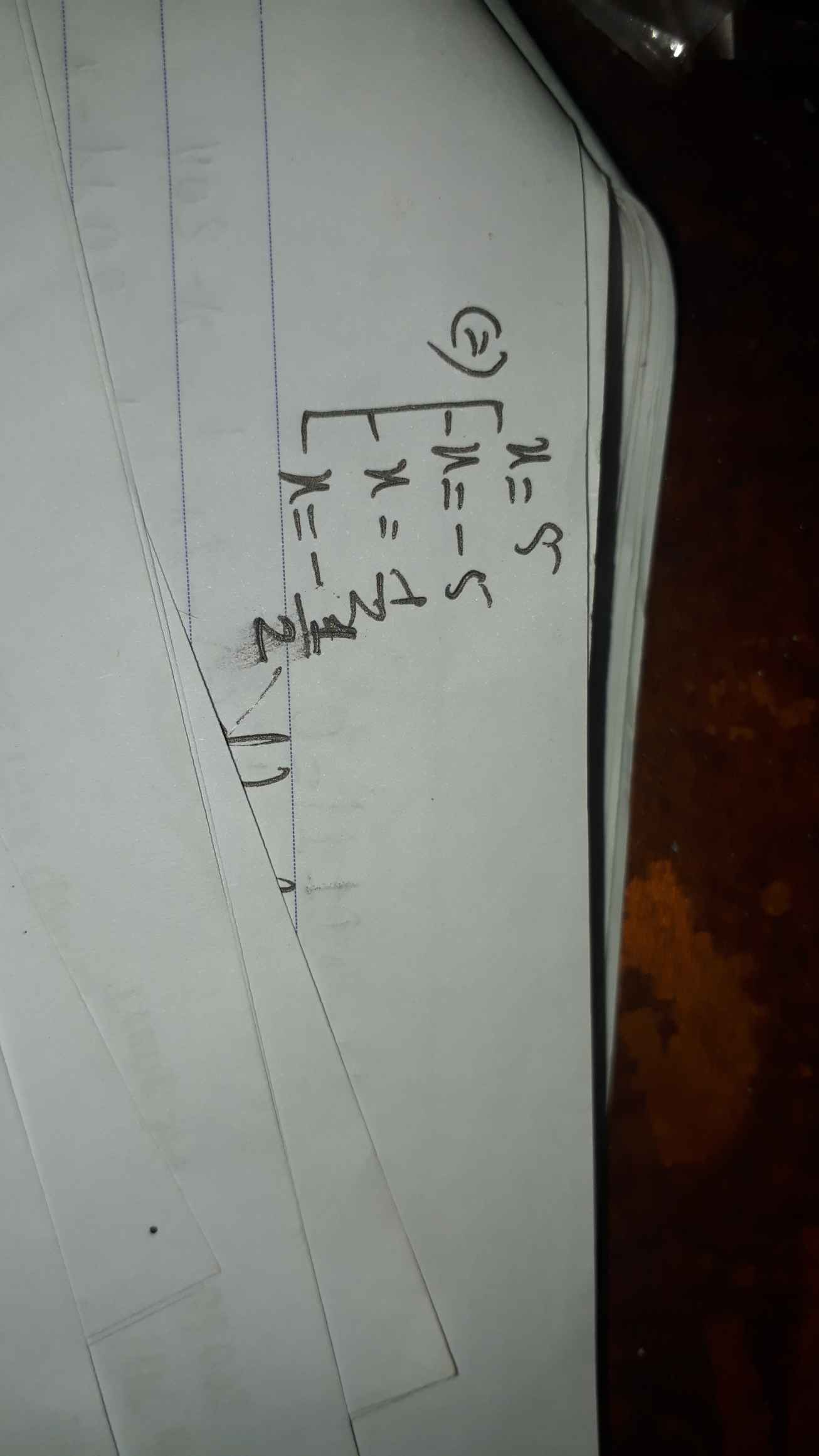
\(3,\left(2x-3\right)^2=\left(x+5\right)^2\)
\(\Leftrightarrow\left(2x-3\right)^2-\left(x+5\right)^2=0\)
\(\Leftrightarrow\left(2x-3-x-5\right)\left(2x-3+x+5\right)=0\)
\(\Leftrightarrow\left(x-8\right)\left(3x+2\right)=0\)
\(\Leftrightarrow\left[{}\begin{matrix}x=8\\x=-\dfrac{2}{3}\end{matrix}\right.\)
\(5,\left(x+8\right)^2=191\)
\(\Leftrightarrow\left(x+8\right)^2-191=0\)
\(\Leftrightarrow\left(x+8-\sqrt{191}\right)\left(x+8+\sqrt{191}\right)=0\)
\(\Leftrightarrow\left[{}\begin{matrix}x=\sqrt{191}-8\\x=-\sqrt{191}-8\end{matrix}\right.\)
\(6,x^2+4-\left(x-2\right)^2=0\)
\(\Leftrightarrow x^2+4-x^2+4x-4=0\)
\(\Leftrightarrow4x=0\Leftrightarrow x=0\)
10 tháng 12 2021
\(a,\Leftrightarrow2x^2+10x-2x^2=12\Leftrightarrow x=\dfrac{12}{10}=\dfrac{6}{5}\\ b,\Leftrightarrow\left(5-2x-4\right)\left(5-2x+4\right)=0\\ \Leftrightarrow\left(1-2x\right)\left(9-2x\right)=0\Leftrightarrow\left[{}\begin{matrix}x=\dfrac{1}{2}\\x=\dfrac{9}{2}\end{matrix}\right.\\ c,\Leftrightarrow3x^2-3x^2+6x=36\Leftrightarrow x=6\\ d,\Leftrightarrow2\left(x+5\right)-x\left(x+5\right)=0\\ \Leftrightarrow\left(2-x\right)\left(x+5\right)=0\Leftrightarrow\left[{}\begin{matrix}x=2\\x=-5\end{matrix}\right.\\ e,\Leftrightarrow4x^2-4x+1-4x^2+196=0\\ \Leftrightarrow-4x=-197\Leftrightarrow x=\dfrac{197}{4}\)
\(f,\Leftrightarrow x^2+8x+16-x^2+1=16\Leftrightarrow8x=-1\Leftrightarrow x=-\dfrac{1}{8}\\ g,Sửa:\left(3x+1\right)^2-\left(x+1\right)^2=0\\ \Leftrightarrow\left(3x+1-x-1\right)\left(3x+1+x+1\right)=0\\ \Leftrightarrow2x\left(4x+2\right)=0\Leftrightarrow\left[{}\begin{matrix}x=0\\x=-\dfrac{1}{2}\end{matrix}\right.\\ h,\Leftrightarrow x^2+8x-x-8=0\\ \Leftrightarrow\left(x+8\right)\left(x-1\right)=0\Leftrightarrow\left[{}\begin{matrix}x=1\\x=-8\end{matrix}\right.\\ i,\Leftrightarrow2x^2-13x+15=0\\ \Leftrightarrow2x^2+2x-15x-15=0\\ \Leftrightarrow\left(x+1\right)\left(2x-15\right)=0\Leftrightarrow\left[{}\begin{matrix}x=-1\\x=\dfrac{15}{2}\end{matrix}\right.\)
PH
4 tháng 4 2020
1) (x+1)2+2x=x(x+1)+6
⇔x2+2x+1+2x=x2+x+6
⇔x2+2x+1+2x-x2-x-6=0
⇔3x-5=0
⇔x=\(\frac{5}{3}\)
Vậy tập nghiệm của phương trình đã cho là:S={\(\frac{5}{3}\)}
HH
tìm x biết :
a,(2x-3)^2 =(x+ 5)^2
b,x^2(x-1) -4x^2 +8x -4 =0
c, (x-4)^2 -36 =0
giúp mik nha mik đang gấp
1
29 tháng 11 2016
a, (2x-3)^2=(x+5)^2
2x-3=x+5
2x-3-x-5=0
x-8=0
x=8
b, x^2(x-1)-4x^2+8x-4=0
x^2(x-1)-(4x^2-8x+4)=0
x^2(x-1)-4(x^2-2x+1)=0
x^2(x-1)-4(x-1)^2=0
(x-1)(x^2-4)(x-1)=0
(x-1)(x-2)(x+2)(x-1)=0
=>x-1=0=>x=1
=>x-2=0=>x=2
=>x+2=0=>x=-2
=>x-1=0=>x=1
Vậy : x=1 ;x=2 và x=-2
c, (x-4)^2-36=0
(x-4)^2-6^2=0
(x-4-6)(x-4+6)=0
(x-10)(x+2)=0
=>x-10=0=>x=10
=>x+2=0=>x=-2
Vậy : x=10 và x=-2
k đúng cho mình nhé bạn !
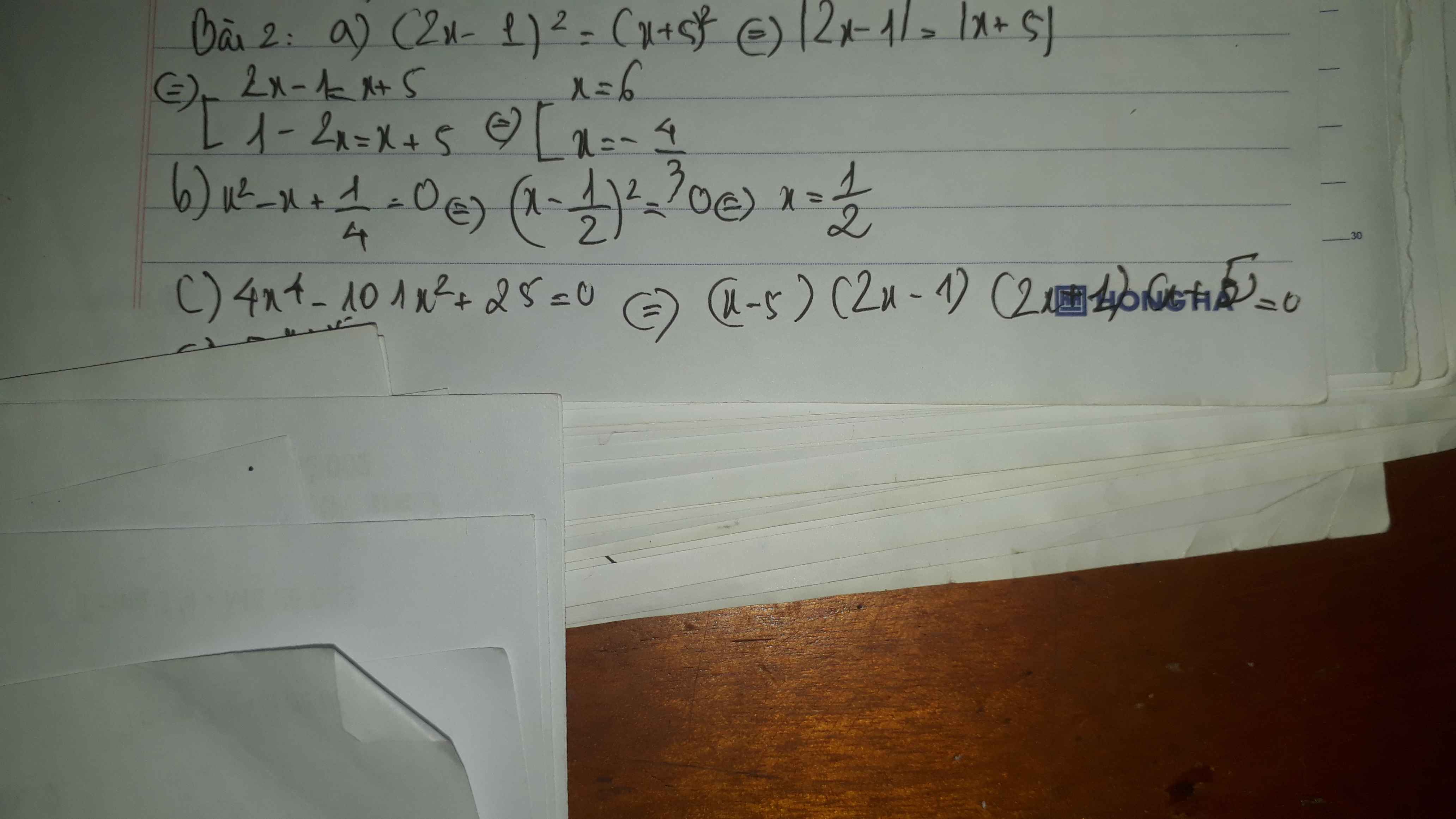
(-5)+(-11)
\(\left(x-1\right)\left(x-4\right)\left(x-5\right)\left(x-8\right)+36=0\)
\(\left[\left(x-1\right)\left(x-8\right)\right]\left[\left(x-4\right)\left(x-5\right)\right]+36=0\)
\(\left(x^2-9x+8\right)\left(x^2-9x+20\right)+36=0\)
Đặt \(a=x^2-9x+14\)ta có :
\(\left(a-6\right)\left(a+6\right)+36=0\)
\(a^2-6^2+36=0\)
\(a^2=0\)
Thay \(a=x^2-9x+14\)ta có :
\(\left(x^2-9x+14\right)^2=0\)
\(\Leftrightarrow x^2-9x+14=0\)
\(\Leftrightarrow x^2-2x-7x+14=0\)
\(\Leftrightarrow x\left(x-2\right)-7\left(x-2\right)=0\)
\(\Leftrightarrow\left(x-2\right)\left(x-7\right)=0\)
\(\Rightarrow\orbr{\begin{cases}x-2=0\\x-7=0\end{cases}\Rightarrow\orbr{\begin{cases}x=2\\x=7\end{cases}}}\)
Vậy,...........