K
Khách
Hãy nhập câu hỏi của bạn vào đây, nếu là tài khoản VIP, bạn sẽ được ưu tiên trả lời.
Các câu hỏi dưới đây có thể giống với câu hỏi trên
AH
Akai Haruma
Giáo viên
28 tháng 5 2020
Câu 8:
$(x-1)(2+x)>0$ thì có 2 TH xảy ra:
TH1: \(\left\{\begin{matrix} x-1>0\\ x+2>0\end{matrix}\right.\Leftrightarrow \left\{\begin{matrix} x>1\\ x>-2\end{matrix}\right.\Rightarrow x>1\)
TH2: \(\left\{\begin{matrix} x-1< 0\\ x+2< 0\end{matrix}\right.\Leftrightarrow \left\{\begin{matrix} x< 1\\ x< -2\end{matrix}\right.\Rightarrow x< -2\)
Vậy $x\in (1;+\infty)$ hoặc $x\in (-\infty; -2)$
AH
Akai Haruma
Giáo viên
28 tháng 5 2020
Câu 7:
$|x^2+x-12|=|(x-3)(x+4)|$
Nếu $x\geq 3$ thì $(x-3)(x+4)\geq 0$
$\Rightarrow |x^2+x-12|=x^2+x-12$
BPT trở thành: $x^2+x-12< x^2+x+12$ (luôn đúng)
Nếu $3> x> -4(1)$ thì $(x-3)(x+4)< 0$
$\Rightarrow |x^2+x-12|=-(x^2+x-12)$
BPT trở thành: $-(x^2+x-12)< x^2+x+12$
$\Leftrightarrow 2(x^2+x)>0\Leftrightarrow x>0$ hoặc $x< -1$
Kết hợp với $(1)$ suy ra $3>x>0$ hoặc $-1> x> -4$
Nếu $x\leq -4$ thì $(x-3)(x+4)\geq 0$
$\Rightarrow |x^2+x-12|=x^2+x-12$
BPT trở thành: $x^2+x-12< x^2+x+12$ (luôn đúng)
Vậy BPT có nghiệm $x\in (+\infty; 0)$ hoặc $x\in (-\infty; -1)$
15 tháng 4 2017
a) <=>
Miền nghiệm của hệ bất phương trình là miền không bị gạch sọc ở hình bên (không kể các điểm).
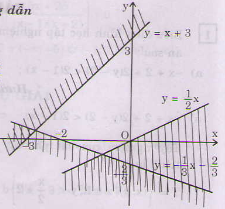
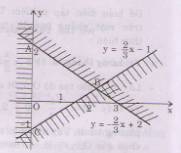
b) <=>
Miền nghiệm của hệ bất phương trình là miền tam giác ABC bao gồm cả các điểm trên cạnh AC và cạnh BC (không kể các điểm của cạnh AB).
16 tháng 2 2021
a, \(\dfrac{x-2}{x+1}\ge\dfrac{x+1}{x-2}\)
⇔ \(\dfrac{\left(x-2\right)^2-\left(x+1\right)^2}{\left(x-1\right)\left(x+2\right)}\ge0\)
⇔ \(\dfrac{3-6x}{\left(x+1\right)\left(x-2\right)}\) ≥ 0
⇔ \(\dfrac{2x-1}{\left(x+1\right)\left(x-2\right)}\) ≤ 0
⇔ \(\left[{}\begin{matrix}\left\{{}\begin{matrix}x\ge\dfrac{1}{2}\\-1< x< 2\end{matrix}\right.\\\left\{{}\begin{matrix}x\le\dfrac{1}{2}\\\left[{}\begin{matrix}x< -1\\x>2\end{matrix}\right.\end{matrix}\right.\end{matrix}\right.\Leftrightarrow\left[{}\begin{matrix}\dfrac{1}{2}\le x< 2\\x< -1\end{matrix}\right.\)
Vậy tập nghiệm là \(\left(-\infty;-1\right)\cup\) \(\left[\dfrac{1}{2};2\right]\)\ {2}
Bạn có thể biến cái ngoặc vuông kia (ở chỗ số 2) thành ngoặc tròn
Còn vì sao mình không biến cái ngoặc vuông kia (ở chỗ số 2) thành ngoặc tròn thì đó là một câu chuyện dài
b, tương tự, chuyển vế đổi dấu
6 tháng 4 2017
Ta có: điều kiện xác định của bpt \(x+3-\dfrac{1}{x+7}< -\dfrac{1}{x+7}\) là \(x\ne-7\)
\(\Rightarrow x=-7\) không phải là nghiệm của bpt trên
Lại có: \(x+3< 2\\ \Leftrightarrow x< 2-3\\ \Leftrightarrow x< -1\)
\(\Rightarrow x=-7\) thỏa mãn bpt \(x+3< 2\) \(\left(-7< -1\right)\)
DN
1
NV
Nguyễn Việt Lâm
Giáo viên
9 tháng 5 2020
\(3x^2+10x+3< 0\)
\(\Rightarrow-3< x< -\frac{1}{3}\)
Đáp án C đúng
PH
1)tập nghiệm của bất phương trình x -1 < \(\left|x+1\right|\)là: a) (0; +∞) b) (1;+∞) c) (-∞;1) d) R
1
NV
Nguyễn Việt Lâm
Giáo viên
8 tháng 3 2020
- Với \(x< 1\Rightarrow\left\{{}\begin{matrix}VT< 0\\VP\ge0\end{matrix}\right.\) BPT luôn đúng
- Với \(x\ge1\Rightarrow x+1>0\) BPT tương đương:
\(x-1< x+1\Leftrightarrow-1< 1\) (luôn đúng)
Vậy tập nghieemh của BPT là R
NT
2
MH
10 tháng 5 2017
TXĐ:D=R\{-2;1}
BPT<=>\(\dfrac{\left(x-1\right)^2-\left(x+2\right)^2}{\left(x-1\right)\left(x+2\right)}\ge0\)
<=>\(\dfrac{-3\left(2x+1\right)}{\left(x-1\right)\left(x+2\right)}\ge0\)
Cho 2x+1=0<=>x=-0,5
cho (x-1)(x+2)=0 <=>x=1 hoặc x=-2
Bảng xét dấu:
x -\(\infty\) -2 -0,5 1 +\(\infty\)
f(x) + kxđ - 0 + kxđ -
=>Tập nghiệm T=(-\(\infty\);-2)\(\cup\)[-0,5;1]
HK
6 tháng 5 2020
Vô nghiệm với mọi x?
a/ \(\Leftrightarrow\left\{{}\begin{matrix}m-3< 0\\\Delta\le0\end{matrix}\right.\Leftrightarrow\left\{{}\begin{matrix}m< 3\\\left(m+2\right)^2+16\left(m-3\right)\le0\end{matrix}\right.\)
\(\Leftrightarrow m^2+20m-44\le0\)
\(\Leftrightarrow-22\le m\le2\)
b/ \(\Leftrightarrow\left\{{}\begin{matrix}m< 0\\\Delta'< 0\end{matrix}\right.\Leftrightarrow\left\{{}\begin{matrix}m< 0\\\left(m-1\right)^2-4m< 0\end{matrix}\right.\)
\(\Leftrightarrow\left\{{}\begin{matrix}m< 0\\3-2\sqrt{2}< m< 3+2\sqrt{2}\end{matrix}\right.\)
=> ko tồn tại m thoả mãn
c/ \(\Leftrightarrow\left\{{}\begin{matrix}m^2+2m-3>0\\\Delta'\le0\end{matrix}\right.\Leftrightarrow\left\{{}\begin{matrix}\left[{}\begin{matrix}m>1\\m< -3\end{matrix}\right.\\\left(m-1\right)^2-\left(m^2+2m-3\right)\le0\end{matrix}\right.\)
\(\Leftrightarrow\left\{{}\begin{matrix}\left[{}\begin{matrix}m>1\\m< -3\end{matrix}\right.\\m\ge1\end{matrix}\right.\Rightarrow m>1\)
\(\dfrac{1-x}{1+x}< 0 \Leftrightarrow\left[{}\begin{matrix}\left\{{}\begin{matrix}1-x< 0\\1+x>0\end{matrix}\right.\\\left\{{}\begin{matrix}1-x>0\\1+x< 0\end{matrix}\right.\end{matrix}\right.\Leftrightarrow\left[{}\begin{matrix}\left\{{}\begin{matrix}x>1\\x>-1\end{matrix}\right.\\\left\{{}\begin{matrix}x< 1\\x< -1\end{matrix}\right.\end{matrix}\right.\Leftrightarrow\left[{}\begin{matrix}x\in\left(1;+\infty\right)\\x\in\left(-\infty;-1\right)\end{matrix}\right.\)
Vậy \(x\in\left(-\infty;-1\right)\cup\left(1;+\infty\right)\) thỏa mãn