Hãy nhập câu hỏi của bạn vào đây, nếu là tài khoản VIP, bạn sẽ được ưu tiên trả lời.

a, Để 3/(n-1) nguyên
<=> 3 chia hết cho n-1
Mà n-1 nguyên
=> n-1 thuộc Ư(3)={-3,-1,1,3}
=> n=-2,0,2,4

Bài 1.
Giải
a) Ta có: \(A=\dfrac{3n+9}{n-4}=\dfrac{3n-12+21}{n-4}=\dfrac{3\left(n-4\right)+21}{n-4}=3+\dfrac{21}{n-4}\)
Để \(A\in Z\) thì \(\dfrac{21}{n-4}\in Z\)
\(\Rightarrow21⋮\left(n-4\right)\)
\(\Rightarrow\left(n-4\right)\inƯ\left(21\right)\)
\(\Rightarrow\left(n-4\right)\in\left\{\pm1;\pm3;\pm7;\pm21\right\}\)
Ta có bẳng sau:
| \(n-4\) | \(-21\) | \(-7\) | \(-3\) | \(-1\) | \(1\) | \(3\) | \(7\) | \(21\) |
| \(n\) | \(-17\) | \(-3\) | \(1\) | \(3\) | \(5\) | \(7\) | \(11\) | \(25\) |
Vậy \(n\in\left\{-17;-3;1;3;5;7;11;25\right\}\) thì \(A\in Z.\)
b) Ta có: \(B=\dfrac{6n+5}{2n-1}=\dfrac{6n-3+8}{2n-1}=\dfrac{3\left(2n-1\right)+8}{2n-1}=3+\dfrac{8}{2n-1}\)
Để \(B\in Z\) thì \(\dfrac{8}{2n-1}\in Z\)
\(\Rightarrow8⋮\left(2n-1\right)\)
\(\Rightarrow\left(2n-1\right)\inƯ\left(8\right)\)
\(\Rightarrow\left(2n-1\right)\in\left\{\pm1;\pm2;\pm4;\pm8\right\}\)
Ta có bảng sau:
| \(2n-1\) | \(-8\) | \(-4\) | \(-2\) | \(-1\) | \(1\) | \(2\) | \(4\) | \(8\) |
| \(2n\) | \(-7\) | \(-3\) | \(-1\) | \(0\) | \(2\) | \(3\) | \(5\) | \(9\) |
| \(n\) | \(\dfrac{-7}{2}\) | \(\dfrac{-3}{2}\) | \(\dfrac{-1}{2}\) | \(0\) | \(1\) | \(\dfrac{3}{2}\) | \(\dfrac{5}{2}\) | \(\dfrac{9}{2}\) |
Vậy \(n\in\left\{\dfrac{-7}{2};\dfrac{-3}{2};\dfrac{-1}{2};0;1;\dfrac{3}{2};\dfrac{5}{2};\dfrac{9}{2}\right\}\)
Bạn Nguyen Thi Huyen giải bài 1 rồi nên mình giải tiếp các bài kia nhé!
Bài 2:
\(\dfrac{x-18}{2000}+\dfrac{x-17}{2001}=\dfrac{x-16}{2002}+\dfrac{x-15}{2003}\)
\(\Leftrightarrow\left(\dfrac{x-18}{2000}-1\right)+\left(\dfrac{x-17}{2001}-1\right)=\left(\dfrac{x-16}{2002}-1\right)+\left(\dfrac{x-15}{2003}-1\right)\)
\(\Leftrightarrow\dfrac{x-2018}{2000}+\dfrac{x-2018}{2001}=\dfrac{x-2018}{2002}+\dfrac{x-2018}{2003}\)
\(\Leftrightarrow\dfrac{x-2018}{2000}+\dfrac{x-2018}{2001}-\dfrac{x-2018}{2002}-\dfrac{x-2018}{2003}=0\)
\(\Leftrightarrow\left(x-2018\right)\left(\dfrac{1}{2000}+\dfrac{1}{2001}-\dfrac{1}{2002}-\dfrac{1}{2003}\right)=0\)
Dễ thấy \(\dfrac{1}{2000}>\dfrac{1}{2001}>\dfrac{1}{2002}>\dfrac{1}{2003}\) nên:
\(\dfrac{1}{2000}+\dfrac{1}{2001}+\dfrac{1}{2002}+\dfrac{1}{2003}\ne0\). Do đó:
\(x-2018=0\Leftrightarrow x=2018\)
Bài 3:
a) \(\dfrac{5}{x}+\dfrac{y}{4}=\dfrac{1}{8}\Leftrightarrow\dfrac{20}{4x}+\dfrac{xy}{4x}=\dfrac{20+xy}{4x+4x}=\dfrac{20+xy}{8x}=\dfrac{1}{8}\)
Hoán vị ngoại tỉ ta có: \(\dfrac{20+xy}{8x}=\dfrac{1}{8}\Leftrightarrow\dfrac{8}{8x}=\dfrac{1}{x}=\dfrac{1}{8}\Leftrightarrow x=8\)
Thế x = 8 vào : \(\dfrac{5}{x}+\dfrac{y}{4}=\dfrac{1}{8}\) .Ta có: \(\dfrac{5}{8}+\dfrac{y}{4}=\dfrac{1}{8}\Leftrightarrow\dfrac{y}{4}=\dfrac{1}{8}-\dfrac{5}{8}=\dfrac{-2}{4}\). Ta có: \(\dfrac{y}{4}=\dfrac{-2}{4}\Leftrightarrow y=-2\)
Vậy: \(\left[{}\begin{matrix}x=8\\y=-2\end{matrix}\right.\)
b) \(\dfrac{1}{x}-\dfrac{2}{y}=\dfrac{3}{1}\Rightarrow\dfrac{y}{x}-2=\dfrac{3}{1}\) (hoán vị ngoại tỉ)
\(\Leftrightarrow\dfrac{y}{x}=\dfrac{5}{1}\). Suy ra nghiệm x,y có dạng \(\left[{}\begin{matrix}x=1k\\y=5k\end{matrix}\right.\left(k\in Z\right)\). Bằng các phép thử lại ta dễ dàng suy ra x,y vô nghiệm.

1.a) để A là số hữu tỉ thì 2n+3 nguyên và n - 1 khác 0
từ hai điều kiện trên suy ra n nguyên và n khác 1
b) để A nguyên thì 2n+3 ⋮ n - 1
⇒ 2(n - 1) +5 ⋮ n - 1
⇒ 5 ⋮ n - 1
⇒n ∈ {-4; 0; 2; 6}
2. x < y ⇔ \(\dfrac{a}{n}< \dfrac{b}{n}\)
\(\Rightarrow\dfrac{2a}{2n}< \dfrac{a+b}{2n}< \dfrac{2b}{2n}\Leftrightarrow x< z< y\)

Bài 1:
a) \(\left|3x-5\right|=4\)
\(\Leftrightarrow\left[{}\begin{matrix}3x-5=4\\3x-5=-4\end{matrix}\right.\)
\(\Leftrightarrow\left[{}\begin{matrix}3x=9\\3x=1\end{matrix}\right.\)
\(\Leftrightarrow\left[{}\begin{matrix}x=3\\x=\dfrac{1}{3}\end{matrix}\right.\)
Vậy \(x_1=\dfrac{1}{3};x_2=3\)
b) \(\dfrac{x+1}{10}+\dfrac{x+1}{11}+\dfrac{x+1}{12}=\dfrac{x+1}{13}+\dfrac{x+1}{14}\)
cho đáp án tự làm (vì cách lm của mik bị ném đá khá nhiều lần òi :D)
\(x=-1\)
c) như câu b nhé :D
\(x=-2004\)

3a)Vì A là số nguyên
=>\(3n+9⋮n-4=>3n-12+21⋮n-4=>3.\left(n-4\right)+21⋮n-4\)
Mà \(\text{3 . (n - 4)}⋮n-4\)
=>\(21⋮n-4=>n-4\inƯ\left(21\right)=\left\{-21;-7;-3;-1;1;3;7;21\right\}\)
(Vì n là số nguyên => n - 4 là 1 số nguyên)
=>\(n\in\left\{-17;-3;1;3;5;9;11;25\right\}\)
Ta có bảng sau:
| n | -17 | -3 | 1 | 3 | 5 | 9 | 11 | 25 |
| 3n + 9 | -42 | 0 | 12 | 18 | 24 | 36 | 42 | 84 |
| n - 4 | -21 | -7 | -3 | -1 | 1 | 3 | 7 | 21 |
| \(A=\dfrac{3n+9}{n-4}\) | 2 | 0 | -4 | -18 | 24 | 12 | 6 | 4 |
Vậy.....
b)Vì B là số nguyên
=>\(2n-1⋮n+5=>2n+10-11⋮n+5=>2\left(n+5\right)-11⋮n+5\)
Mà \(\text{2 ( n + 5)}⋮n+5\)
=>\(11⋮n+5=>n+5\in\left\{-11;-1;1;11\right\}\)
(Vì n là số nguyên=> n + 5 là số nguyên)
=> \(n\in\left\{-16;-6;-4;6\right\}\)
Ta có bảng sau:
| n | -16 | -6 | -4 | 6 |
| 2 n - 1 | -33 | -13 | -9 | 11 |
| n + 5 | -11 | -1 | 1 | 11 |
| \(B=\dfrac{2n-1}{n+5}\) | 3 | 13 | -9 |
1 |
Vậy.......
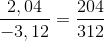

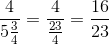
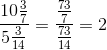
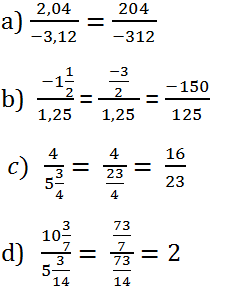
Cái này dễ mà em
a ) Để \(\dfrac{3}{n-1}\) là một số nguyên thì => 3 \(⋮\) (n - 1) hay n - 1 \(\in\) Ư (3) = { \(\pm\)1 , \(\pm\)3 }
=> n-1 = 1 => n= 2
n-1 = 3 => n= 4
n-1 = -1 => n= 0
n-1 = -3 => n= -2
Vậy n = 2 , n= -2 , n= 0 , n= 4
câu b ) tương tự nha em