LT
2
K
Khách
Hãy nhập câu hỏi của bạn vào đây, nếu là tài khoản VIP, bạn sẽ được ưu tiên trả lời.
Các câu hỏi dưới đây có thể giống với câu hỏi trên
OK
2
21 tháng 6 2019
\(2M=\frac{2\sqrt{x}+2}{\sqrt{x}+2}\)
để 2M có giá trị nguyên thì \(2\sqrt{x}+2⋮\sqrt{x}+2\)(1)
Lại có \(2\sqrt{x}+4⋮\sqrt{x}+2\)(2)
\(\Rightarrow2⋮\sqrt{x}+2\)(lấy (2) trừ (1))
mà \(\sqrt{x}+2\ge2\)
\(\Rightarrow\sqrt{x}+2=2\) ( vì x thuộc Z)
=> x=0
TP
21 tháng 6 2019
Ta có: \(M=\frac{\sqrt{x}+1}{\sqrt{x}+2}\) ( ĐK: \(x\ge0\) )
\(\Leftrightarrow2M=\frac{2\left(\sqrt{x}+1\right)}{\sqrt{x}+2}\)
\(\Leftrightarrow2M=\frac{2\sqrt{x}+2}{\sqrt{x}+2}\)
\(\Leftrightarrow2M=\frac{2\sqrt{x}+4-2}{\sqrt{x}+2}\)
\(\Leftrightarrow2M=\frac{2\sqrt{x}+4}{\sqrt{x}+2}-\frac{2}{\sqrt{x}+2}\)
\(\Leftrightarrow2M=2-\frac{2}{\sqrt{x}+2}\)
Để 2M có giá trị nguyên <=> \(2⋮\sqrt{x}+2\)
\(\Leftrightarrow\sqrt{x}+2\inƯ\left(2\right)\)
\(\Leftrightarrow\sqrt{x}+2\in\left\{-1;-2;1;2\right\}\)
Vì \(x\ge0\Leftrightarrow\sqrt{x}+2\ge2\)
\(\Rightarrow\sqrt{x}+2=2\)
\(\Leftrightarrow\sqrt{x}=0\Rightarrow x=0\)
Vậy khi x = 0 thì 2M có giá trị nguyên!
Chúc bạn học tốt! :))
AP
0
L
1
4 tháng 9 2021
Để A nguyên thì \(2\sqrt{x}+3⋮3\sqrt{x}-1\)
\(\Leftrightarrow6\sqrt{x}+9⋮3\sqrt{x}-1\)
\(\Leftrightarrow3\sqrt{x}-1\in\left\{-1;1;11\right\}\)
\(\Leftrightarrow3\sqrt{x}\in\left\{0;12\right\}\)
hay \(x\in\left\{0;16\right\}\)
NC
25 tháng 8 2020
a) đk: \(x\ge0\)
Ta có:
+ Nếu: x không là số chính phương => A vô tỉ (loại)
+ Nếu: x là số chính phương => \(\sqrt{x}\) nguyên
Ta có: \(A=\frac{2\sqrt{x}+10}{\sqrt{x}-3}=\frac{\left(2\sqrt{x}-6\right)+16}{\sqrt{x}-3}=2+\frac{16}{\sqrt{x}-3}\)
Để A nguyên => \(\frac{16}{\sqrt{x}-3}\inℤ\Rightarrow\sqrt{x}-3\inƯ\left(16\right)\)
Mà \(\sqrt{x}-3\ge-3\left(\forall x\right)\Rightarrow\sqrt{x}-3\in\left\{-2;-1;1;2;4;8;16\right\}\)
\(\Rightarrow\sqrt{x}\in\left\{1;2;4;5;7;12;20\right\}\)
\(\Rightarrow x\in\left\{1;4;16;25;49;144;400\right\}\)
NC
25 tháng 8 2020
b) đk: \(x\ge0\)
Ta có:
+ Nếu: x không là số chính phương => A vô tỉ (loại)
+ Nếu: x là số chính phương => \(\sqrt{x}\) nguyên
Ta có: \(B=\frac{\sqrt{x}+8}{2\sqrt{x}+1}\Rightarrow2B=\frac{2\sqrt{x}+16}{2\sqrt{x}+1}=1+\frac{15}{2\sqrt{x}+1}\)
Để 2B nguyên => \(\frac{15}{2\sqrt{x}+1}\inℤ\Rightarrow2\sqrt{x}+1\inƯ\left(15\right)\)
Mà 1 lẻ nên để B nguyên => \(\frac{15}{2\sqrt{x}+1}\) lẻ, mặt khác: \(2\sqrt{x}+1\ge1\left(\forall x\right)\)
=> \(2\sqrt{x}+1\in\left\{1;3;5;15\right\}\Leftrightarrow2\sqrt{x}\in\left\{0;2;4;14\right\}\)
\(\Leftrightarrow\sqrt{x}\in\left\{0;1;2;7\right\}\Rightarrow x\in\left\{0;1;4;49\right\}\)
27 tháng 11 2018
\(Q=\frac{\sqrt{x}\cdot\left(\sqrt{x}-1\right)\cdot\left(x+\sqrt{x}+1\right)}{x+\sqrt{x}+1}-\frac{\sqrt{x}\cdot\left(2\sqrt{x}+1\right)}{\sqrt{x}}+\frac{2\left(\sqrt{x}-1\right)\cdot\left(\sqrt{x}-1\right)}{\sqrt{x}-1}\)
\(Q=x-\sqrt{x}-2\sqrt{x}-1+2\sqrt{x}+2\)
\(Q=x+1\)
Không thể tìm được GTLN hay GTNN của Q.
b)
\(\frac{3x+3}{\sqrt{x}}=3\sqrt{x}+\frac{3}{\sqrt{x}}\)
Để \(\frac{3Q}{\sqrt{x}}\) nguyên thì \(\frac{3}{\sqrt{x}}\)nguyên hay \(\sqrt{x}\inƯ\left(3\right)=\left\{\pm1;\pm3\right\}\)
Vì \(\sqrt{x}\)dương nên \(\sqrt{x}\in\left\{1;3\right\}\)
Vậy x=1, x=9 là các giá trị cần tìm
GM
2
11 tháng 7 2021
Để biểu thức nguyên thì \(3⋮\sqrt{x}+2\)
\(\Leftrightarrow\sqrt{x}+2=3\)
\(\Leftrightarrow\sqrt{x}=1\)
hay x=1
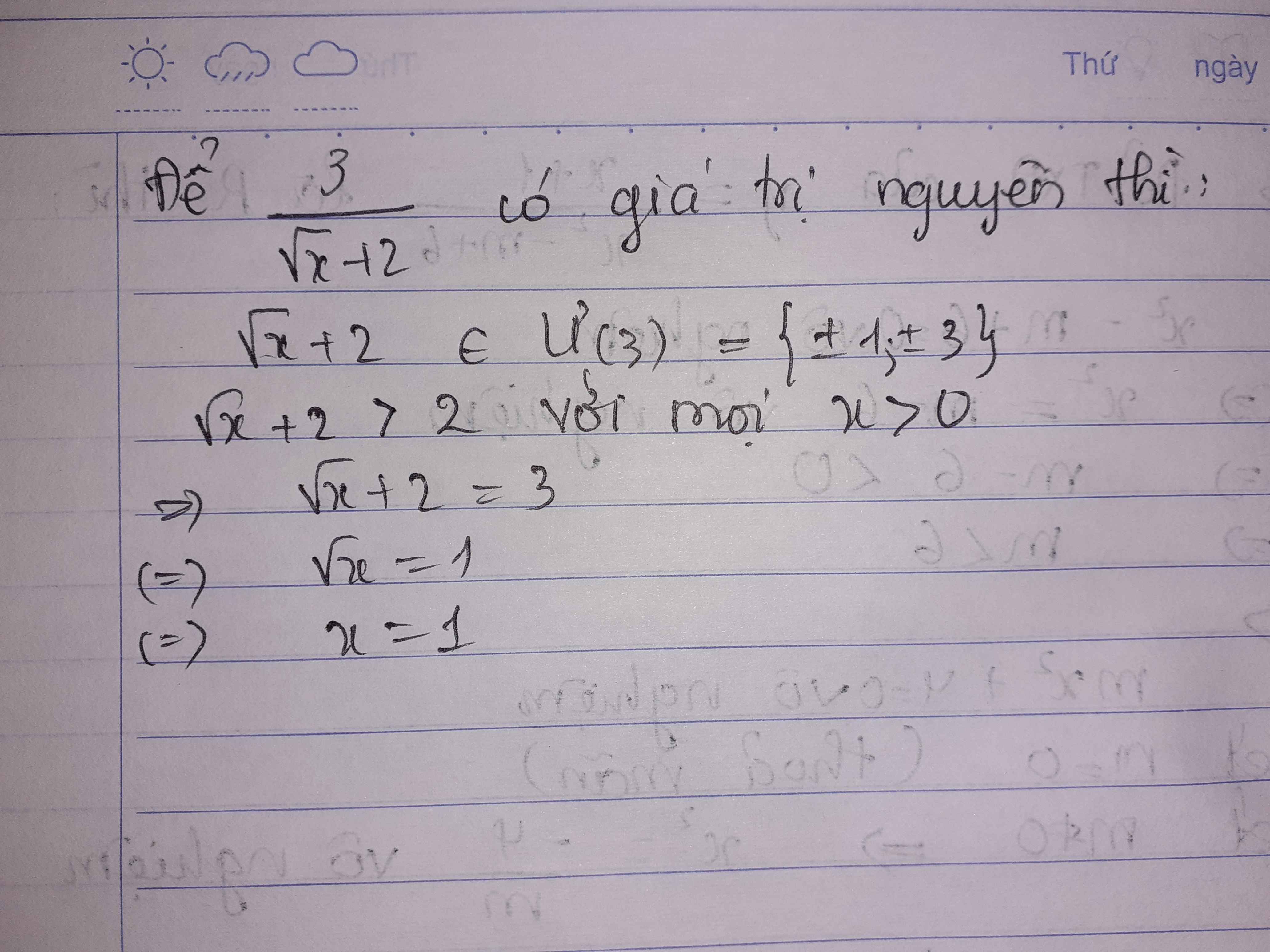
\(\dfrac{x+1}{x+3}=\dfrac{x+3-2}{x+3}=1-\dfrac{2}{x+3}\)
\(Để.P\in Z\Rightarrow\dfrac{2}{x+3}\in Z\\ \Rightarrow x+3\inƯ\left(2\right)=\left\{-2;-1;1;2\right\}\\ \Rightarrow x\in\left\{-5;-4;-2;-1\right\}\)
x thuộc Ư của 2
x+3=2
x+3=-2
x+3=1
x+3=-1
=> x thuộc (-1,-2,-4,-5)