Hãy nhập câu hỏi của bạn vào đây, nếu là tài khoản VIP, bạn sẽ được ưu tiên trả lời.

\(a)\) \(xy-y\sqrt{x}+\sqrt{x}-1\)
= \(y\sqrt{x}.(\sqrt{x}-1)+\sqrt{x}-1\)
=\((\sqrt{x}-1).(y\sqrt{x}+1)\).
\(b)\)\(\sqrt{ax}-\sqrt{by}+\sqrt{bx}-\sqrt{ay}\)
=\(\sqrt{a}.\sqrt{x}-\sqrt{b}.\sqrt{y}+\sqrt{b}.\sqrt{x}-\sqrt{a}.\sqrt{y}\)
=\(\sqrt{a}.\sqrt{x}+\sqrt{b}.\sqrt{x}-\sqrt{a}.\sqrt{y}-\sqrt{b}.\sqrt{y}\)
=\(\sqrt{x}.(\sqrt{a}+\sqrt{b})-\sqrt{y}.(\sqrt{a}+\sqrt{b})\)
=\((\sqrt{x}-\sqrt{y}).(\sqrt{a}+\sqrt{b})\).
\(c)\)\(\sqrt{a+b}+\sqrt{a^2-b^2}\)
=\(\sqrt{a+b}+\sqrt{(a+b).(a-b)}\)
=\(\sqrt{a+b}+\sqrt{a+b}.\sqrt{a-b}\)
=\(\sqrt{a+b}.\left(1+\sqrt{a-b}\right)\).
\(d)\) \(12-\sqrt{x}-x\)
=\(12-4\sqrt{x}+3\sqrt{x}-x\)
=\(4.\left(3-\sqrt{x}\right)+\sqrt{x}\left(3-\sqrt{x}\right)\)
=\(\left(3-\sqrt{x}\right).\left(4+\sqrt{3}\right)\).

\(B=\frac{9-x}{\sqrt{x}+3}-\frac{x-6\sqrt{x}+9}{\sqrt{x}-3}-6\)(đk: x ≥ 0 và x ≠ 9)
\(B=\frac{\left(3-\sqrt{x}\right)\left(3+\sqrt{x}\right)}{\sqrt{x}+3}-\frac{\left(\sqrt{x}-3\right)^2}{\sqrt{x}-3}-6\)
\(B=\left(3-\sqrt{x}\right)-\left(\sqrt{x}-3\right)-6\)
\(B=3-\sqrt{x}-\sqrt{x}+3-6\)
\(B=-2\sqrt{x}\)
\(A=\frac{\sqrt{x}}{\sqrt{x}-6}-\frac{3}{\sqrt{x}+6}+\frac{x}{36-x}\)(đk: x ≥ 0 và x ≠ 36)
\(=\frac{\sqrt{x}}{\sqrt{x}-6}-\frac{3}{\sqrt{x}+6}-\frac{x}{x-36}\)
\(=\frac{\sqrt{x}}{\sqrt{x}-6}-\frac{3}{\sqrt{x}+6}-\frac{x}{x-36}\)
\(=\frac{\sqrt{x}\left(\sqrt{x}+6\right)-3\left(\sqrt{x-6}\right)-x}{(\sqrt{x}-6)\left(\sqrt{x}+6\right)}\)
\(=\frac{x+6\sqrt{x}-3\sqrt{x}+18-x}{(\sqrt{x}-6)\left(\sqrt{x}+6\right)}\)
\(=\frac{3\sqrt{x}+18}{(\sqrt{x}-6)\left(\sqrt{x}+6\right)}\)
\(=\frac{3(\sqrt{x}+6)}{(\sqrt{x}-6)\left(\sqrt{x}+6\right)}\)
\(=\frac{3}{\sqrt{x}-6}\)

2/
a/ \(\sqrt{a}+\frac{1}{\sqrt{a}}\ge2\sqrt{\sqrt{a}.\frac{1}{\sqrt{a}}}=2\), dấu "=" khi \(a=1\)
b/ \(a+b+\frac{1}{2}=a+\frac{1}{4}+b+\frac{1}{4}\ge2\sqrt{a.\frac{1}{4}}+2\sqrt{b.\frac{1}{4}}=\sqrt{a}+\sqrt{b}\)
Dấu "=" khi \(a=b=\frac{1}{4}\)
c/ Có lẽ bạn viết đề nhầm, nếu đề đúng thế này thì mình ko biết làm
Còn đề như vậy: \(\frac{1}{x}+\frac{1}{y}+\frac{1}{z}\ge\frac{1}{\sqrt{xy}}+\frac{1}{\sqrt{yz}}+\frac{1}{\sqrt{xz}}\) thì làm như sau:
\(\frac{1}{x}+\frac{1}{y}\ge\frac{2}{\sqrt{xy}}\) ; \(\frac{1}{y}+\frac{1}{z}\ge\frac{2}{\sqrt{yz}}\); \(\frac{1}{x}+\frac{1}{z}\ge\frac{2}{\sqrt{yz}}\)
Cộng vế với vế ta được:
\(\frac{2}{x}+\frac{2}{y}+\frac{2}{z}\ge\frac{2}{\sqrt{xy}}+\frac{2}{\sqrt{yz}}+\frac{2}{\sqrt{xz}}\Leftrightarrow\frac{1}{x}+\frac{1}{y}+\frac{1}{z}\ge\frac{1}{\sqrt{xy}}+\frac{1}{\sqrt{yz}}+\frac{1}{\sqrt{xz}}\)
Dấu "=" khi \(x=y=z\)
d/ \(\frac{\sqrt{3}+2}{\sqrt{3}-2}-\frac{\sqrt{3}-2}{\sqrt{3}+2}=\frac{\left(\sqrt{3}+2\right)\left(\sqrt{3}+2\right)}{\left(\sqrt{3}-2\right)\left(\sqrt{3}+2\right)}-\frac{\left(\sqrt{3}-2\right)\left(\sqrt{3}-2\right)}{\left(\sqrt{3}+2\right)\left(\sqrt{3}-2\right)}\)
\(=\frac{7+4\sqrt{3}}{3-4}-\frac{7-4\sqrt{3}}{3-4}=-7-4\sqrt{3}+7-4\sqrt{3}=-8\sqrt{3}\)
e/ \(\frac{a\sqrt{a}+b\sqrt{b}}{\sqrt{ab}}:\frac{1}{\sqrt{a}-\sqrt{b}}=\frac{\left(\sqrt{a}\right)^3+\left(\sqrt{b}\right)^3}{\sqrt{ab}}.\left(\sqrt{a}-\sqrt{b}\right)\)
\(=\frac{\left(\sqrt{a}+\sqrt{b}\right)\left(a-\sqrt{ab}+b\right)\left(\sqrt{a}-\sqrt{b}\right)}{\sqrt{ab}}=\frac{\left(a-b\right)\left(a+b-\sqrt{ab}\right)}{\sqrt{ab}}\)
\(=\frac{a^2-b^2}{\sqrt{ab}}-\left(a-b\right)\) (bạn chép đề sai)


 cau c í mk thấy bn chép sai đề nên mk sửa lại đề rồi bạn xem lại đề rồi so với bài làm của mk nha có j ko hiểu thì ib mk nha
cau c í mk thấy bn chép sai đề nên mk sửa lại đề rồi bạn xem lại đề rồi so với bài làm của mk nha có j ko hiểu thì ib mk nha
\(a)VT = \dfrac{{{{\left( {\sqrt a + 1} \right)}^2} - 4\sqrt a }}{{\sqrt a - 1}} + \dfrac{{a + \sqrt a }}{{\sqrt a }}\\ = \dfrac{{a + 2\sqrt a + 1 - 4\sqrt a }}{{\sqrt a - 1}} + \dfrac{{\sqrt a \left( {\sqrt a + 1} \right)}}{{\sqrt a }}\\ = \dfrac{{a - 2\sqrt a + 1}}{{\left( {\sqrt a - 1} \right)}} + \sqrt a + 1\\ = \dfrac{{{{\left( {\sqrt a - 1} \right)}^2}}}{{\sqrt a - 1}} + \sqrt a + 1\\ = \sqrt a - 1 + \sqrt a + 1\\ = 2\sqrt a = VP (đpcm) \)
\(b)VT = \dfrac{{x\sqrt x + y\sqrt y }}{{\sqrt x + \sqrt y }} - {\left( {\sqrt x - \sqrt y } \right)^2}\\ = \dfrac{{\left( {\sqrt x + \sqrt y } \right)\left( {x - \sqrt {xy} + y} \right)}}{{\sqrt x + \sqrt y }} - \left( {x - 2\sqrt {xy} + y} \right)\\ = x - \sqrt {xy} + y - x + 2\sqrt {xy} - y\\ = \sqrt {xy} (đpcm)\\ c)VT = \dfrac{{a\sqrt b - b\sqrt a }}{{\sqrt {ab} }}:\dfrac{{a - b}}{{\sqrt a + \sqrt b }}\\ = \dfrac{{\sqrt {ab} \left( {\sqrt a - \sqrt b } \right)}}{{\sqrt {ab} }}.\dfrac{{\sqrt a + \sqrt b }}{{a - b}}\\ = \sqrt a - \sqrt b .\dfrac{{\sqrt a + \sqrt b }}{{a - b}}\\ = \dfrac{{\left( {\sqrt a - \sqrt b } \right)\left( {\sqrt a + \sqrt b } \right)}}{{a - b}}\\ = \dfrac{{a - b}}{{a - b}} = 1 (đpcm)\\ d)VT = \left[ {\dfrac{{{{\left( {\sqrt a - \sqrt b } \right)}^2} + 4\sqrt {ab} }}{{\sqrt a + \sqrt b }} - \dfrac{{a\sqrt b - b\sqrt a }}{{\sqrt {ab} }}} \right]:\sqrt b \\ = \dfrac{{a - 2\sqrt {ab} + b + 4\sqrt {ab} }}{{\sqrt a + \sqrt b }} - \dfrac{{\sqrt {ab} \left( {\sqrt a - \sqrt b } \right)}}{{\sqrt {ab} }}:\sqrt b \\ = \dfrac{{{{\left( {\sqrt a + \sqrt b } \right)}^2}}}{{\sqrt a + \sqrt b }} - \left( {\sqrt a - \sqrt b } \right):\sqrt b \\ = \sqrt a + \sqrt b - \sqrt a + \sqrt b :\sqrt b \\ = \dfrac{{2\sqrt b }}{{\sqrt b }} = 2 (đpcm) \)
Câu c đề sai (đã sửa)

Bài 2:
a: \(\sqrt{ax}+\sqrt{by}-\sqrt{bx}-\sqrt{ay}\)
\(=\sqrt{a}\left(\sqrt{x}-\sqrt{y}\right)-\sqrt{b}\left(\sqrt{x}-\sqrt{y}\right)\)
\(=\left(\sqrt{x}-\sqrt{y}\right)\left(\sqrt{a}-\sqrt{b}\right)\)
b: \(\sqrt{a-b}-\sqrt{a^2-b^2}\)
\(=\sqrt{a-b}-\sqrt{a-b}\cdot\sqrt{a+b}\)
\(=\sqrt{a-b}\left(1-\sqrt{a+b}\right)\)

Bài 2:
a: \(\sqrt{ax}-\sqrt{bx}+\sqrt{by}-\sqrt{ay}\)
\(=\sqrt{x}\left(\sqrt{a}-\sqrt{b}\right)-\sqrt{y}\left(\sqrt{a}-\sqrt{b}\right)\)
\(=\left(\sqrt{a}-\sqrt{b}\right)\left(\sqrt{x}-\sqrt{y}\right)\)
b: \(\sqrt{a-b}-\sqrt{a^2-b^2}\)
\(=\sqrt{a-b}-\sqrt{a-b}\cdot\sqrt{a+b}\)
\(=\sqrt{a-b}\left(1-\sqrt{a+b}\right)\)

a, \(7\sqrt{AB}+7B-\sqrt{A}-\sqrt{B}=7\sqrt{B}\left(\sqrt{A}+\sqrt{B}\right)-\left(\sqrt{A}+\sqrt{B}\right)\)\(=\left(\sqrt{A}+\sqrt{B}\right)\left(7\sqrt{B}-1\right)\)
b, \(a\sqrt{b}-b\sqrt{a}+\sqrt{a}-\sqrt{b}=\sqrt{ab}\left(\sqrt{a}-\sqrt{b}\right)+\left(\sqrt{a}-\sqrt{b}\right)\)
\(=\left(\sqrt{a}-\sqrt{b}\right)\left(\sqrt{ab}+1\right)\)
c,\(\sqrt{x^2-25y^2}-\sqrt{x-5y}=\sqrt{x-5y}.\sqrt{x+5y}-\sqrt{x-5y}\)
\(=\sqrt{x-5y}\left(\sqrt{x+5y}-1\right)\)
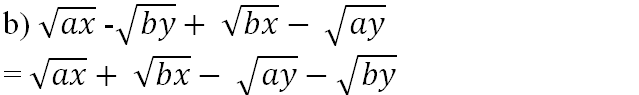
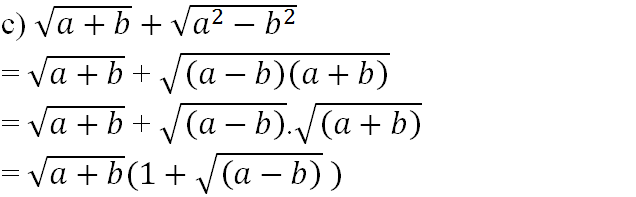
a) \(\sqrt{ax}-\sqrt{by}+\sqrt{bx}-\sqrt{ay}\)
\(=\sqrt{a}\left(\sqrt{x}-\sqrt{y}\right)+\sqrt{b}\left(\sqrt{x}-\sqrt{y}\right)\)
\(=\left(\sqrt{x}-\sqrt{y}\right)\left(\sqrt{a}+\sqrt{b}\right)\)
b) \(7\sqrt{ab}+7b-\sqrt{a}-\sqrt{b}\)
\(=7\sqrt{b}\left(\sqrt{a}+\sqrt{b}\right)-\left(\sqrt{a}+\sqrt{b}\right)\)
\(=\left(\sqrt{a}+\sqrt{b}\right)\left(7\sqrt{b}-1\right)\)
c) \(a\sqrt{b}-b\sqrt{a}+\sqrt{a}-\sqrt{b}\)
\(=\sqrt{a}\left(\sqrt{ab}+1\right)-\sqrt{b}\left(\sqrt{ab}+1\right)\)
\(=\left(\sqrt{ab}+1\right)\left(\sqrt{a}-\sqrt{b}\right)\)
d) \(\sqrt{x^2-25y^2}-\sqrt{x-5y}\)
\(=\sqrt{\left(x-5y\right)\left(x+5y\right)}-\sqrt{x-5y}\)
\(=\sqrt{x-5y}\left(\sqrt{x+5y}-1\right)\)