Hãy nhập câu hỏi của bạn vào đây, nếu là tài khoản VIP, bạn sẽ được ưu tiên trả lời.

Theo hệ thức Viet: \(\left\{{}\begin{matrix}x_1+x_2=-\dfrac{5}{3}\\x_1x_2=-2\end{matrix}\right.\)
Ta có: \(\left\{{}\begin{matrix}y_1+y_2=2x_1-x_2+2x_2-x_1\\y_1y_2=\left(2x_1-x_2\right)\left(2x_2-x_1\right)\end{matrix}\right.\)
\(\Rightarrow\left\{{}\begin{matrix}y_1+y_2=x_1+x_2\\y_1y_2=-2x_1^2-2x_2^2+5x_1x_2\end{matrix}\right.\)
\(\Rightarrow\left\{{}\begin{matrix}y_1+y_2=-\dfrac{5}{3}\\y_1y_2=-2\left(x_1+x_2\right)^2+9x_1x_2\end{matrix}\right.\)
\(\Rightarrow\left\{{}\begin{matrix}y_1+y_2=-\dfrac{5}{3}\\y_1y_2=-2.\left(-\dfrac{5}{3}\right)^2+9.\left(-2\right)=-\dfrac{212}{9}\end{matrix}\right.\)
\(\Rightarrow y_1;y_2\) là nghiệm của:
\(y^2+\dfrac{5}{3}y-\dfrac{212}{9}=0\Leftrightarrow9y^2+10y-212=0\)

\(2x^2-6x-3=0\)
\(\Delta'=3^2+3.2=15>0\)
⇒ Phương trình có hai nghiệm phân biệt.
Theo hệ thức viét có : \(\left\{{}\begin{matrix}x_1+x_2=3\\x_1x_2=-\dfrac{3}{2}\end{matrix}\right.\)
Ta có : \(A=x_1^2x_2^2-2x_1-2x_2=\left(x_1x_2\right)^2-2\left(x_1+x_2\right)=\left(-\dfrac{3}{2}\right)^2-2.3=-\dfrac{15}{4}\)
Vậy \(A=-\dfrac{15}{4}\) thì thỏa mãn điều kiện bài ra.

a) Do a = 3; c = -7 nên a và c trái dấu
Vậy phương trình luôn có hai nghiệm phân biệt
b) Theo Viét ta có:
x₁ + x₂ = -4/3
x₁x₂ = -7/3
Ta có:
2x₁ - (x₁ - x₂ - x₁x₂)
= 2x₁ - x₁ + x₂ + x₁x₂
= x₁ + x₂ + x₁x₂
= -4/3 - 7/3
= -11/3
\(3x^2+4x-7=0\)
\(a,\) Để pt có 2 nghiệm phân biệt thì \(\Delta>0\Rightarrow4^2-4.3.\left(-7\right)=100>0\)
Vậy pt có 2 nghiệm phân biệt \(x_1,x_2\)
\(b,\)Theo Vi-ét, ta có :
\(\left\{{}\begin{matrix}x_1+x_2=-\dfrac{b}{a}=-\dfrac{4}{3}\\x_1x_2=\dfrac{c}{a}=-\dfrac{7}{3}\end{matrix}\right.\)
Ta có : \(2x_1-\left(x_1-x_2-x_1x_2\right)\)
\(=2x_1-x_1+x_2-x_1x_2\)
\(=x_1+x_2-x_1x_2\)
\(=-\dfrac{4}{3}-\left(-\dfrac{7}{3}\right)\)
\(=-\dfrac{4}{3}+\dfrac{7}{3}\)
\(=\dfrac{3}{3}=1\)
Vậy giá trị của biểu thức là \(1\)

A=(x1-x2)^2-x1^2+x1(x1+x2)
=(x1-x2)^2+x1x2
=(x1+x2)^2-x1x2
=(1/2)^2-(-1/4)=1/4+1/4=1/2

Theo định lí Vi-et , ta có : \(\begin{cases}x_1+x_2=1\\x_1.x_2=-5\end{cases}\)
- \(A=x_1^2+x_2^2=\left(x_1+x_2\right)^2-2x_1x_2=1-2.\left(-5\right)=11\)
- \(B=x_1^3+x_2^3=\left(x_1+x_2\right)^3-3x_1x_2\left(x_1+x_2\right)=1-3.\left(-5\right).1=16\)
- \(C=\left(2x_1+x_2\right)\left(2x_2+x_1\right)=\left(1+x_1\right)\left(1+x_2\right)=\left(x_1+x_2\right)+x_1.x_2+1=1-5+1=-3\)

delta= \(\left(-5\right)^2-4.2.\left(-1\right)=25+8=33>0..\)
=> pt có 2 nghiệm phân biệt
Áp dụng hệ thức Vi-et:
\(\hept{\begin{cases}x_1+x_2=-\frac{5}{2}\\x_1x_2=\frac{-1}{2}\end{cases}}\)
A= \(x_1^2-2x_1-2x_2+x_2^2=x_1^2+x_2^2+2x_1x_2-2x_1x_2-2\left(x_1+x_2\right)..\)
\(\Leftrightarrow A=\left(x_1+x_2\right)^2-2x_1x_2-2\left(x_1+x_2\right)..\)
Thay vào A ta được: \(A=\left(-\frac{5}{2}\right)^2-2.\left(-\frac{1}{2}\right)-2.\left(-\frac{5}{2}\right).\)
\(=\frac{25}{4}+1+5=\frac{49}{4}.\)
Học tốt
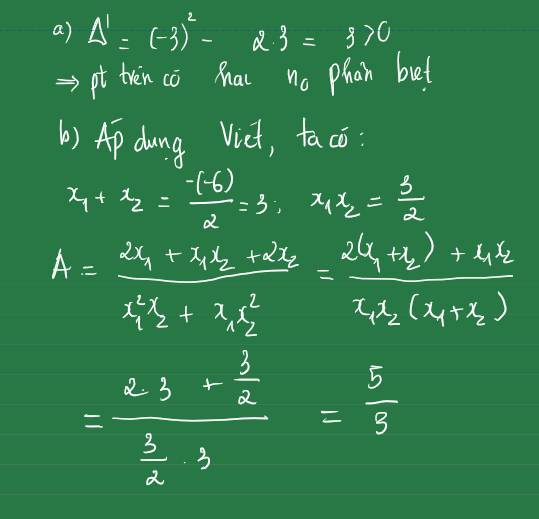

Lời giải:
Theo định lý Viet:
$x_1+x_2=2$
$x_1x_2=-6$
Khi đó:
$A=2x_1-x_1x_2+2x_2=2(x_1+x_2)-x_1x_2$
$=2.2-(-6)=4+6=10$