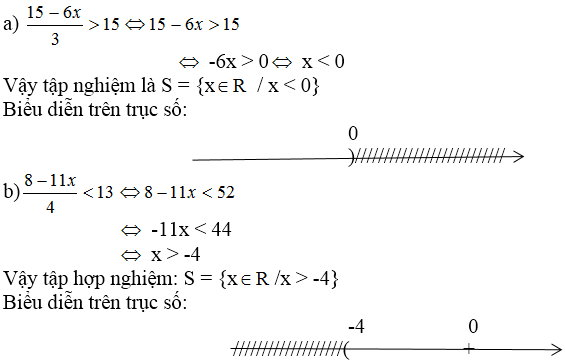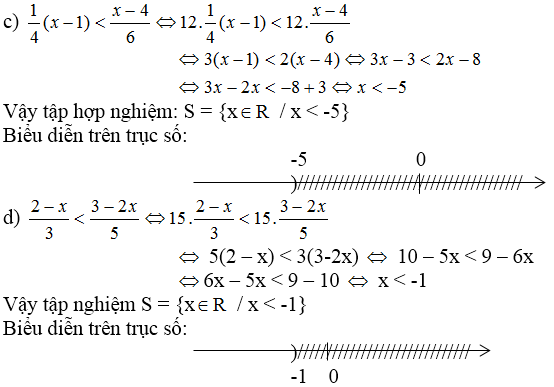Hãy nhập câu hỏi của bạn vào đây, nếu là tài khoản VIP, bạn sẽ được ưu tiên trả lời.

\(a,x\left(x-5\right)+6< 0\Leftrightarrow\left(x+6\right)\left(x-5\right)< 0\)
\(\orbr{\begin{cases}x+6< 0\\x-5< 0\end{cases}\Leftrightarrow\orbr{\begin{cases}x< -6\\x< 5\end{cases}}}\)
\(b,x^2+\left(x-2\right)\left(x+2\right)>2x\left(x-2\right)\)
\(\Leftrightarrow x^2+x^2-4>2x^2-4x\Leftrightarrow-4>-4x\)
\(\Leftrightarrow-4x< -4\Rightarrow x>1\)
\(c,\left(x-3\right)\left(x-3\right)+\left(x+5\right)\left(x+5\right)< 2\left(x-3\left(x+5\right)\right)\)
\(\Leftrightarrow x^2-6x+9+x^2+10x+25< 2x^2+4x-30\)
\(\Leftrightarrow2x^2-2x^2+4x-4x< -30-34\)
\(\Leftrightarrow0x< -64\)
bất phương trình vô nghiệm

Bài 1 :
\(A=\left(x-1\right)\left(x-2\right)\left(x+7\right)\left(x+8\right)+8\)
\(A=\left[\left(x-1\right)\left(x+7\right)\right]\left[\left(x-2\right)\left(x+8\right)\right]+8\)
\(A=\left(x^2+6x-7\right)\left(x^2+6x-16\right)+8\)
Đặt \(a=x^2+6x-7\)
\(A=a\left(a-9\right)+8\)
\(A=a^2-9a+8\)
\(A=a^2-8a-a+8\)
\(A=a\left(a-8\right)-\left(a-8\right)\)
\(A=\left(a-8\right)\left(a-1\right)\)
Thay a vào là xong bạn :)

a) Ta có: \(3x+2< x+8\)
\(\Leftrightarrow3x-x< 8-2\)
\(\Leftrightarrow2x< 6\)
\(\Leftrightarrow x< 3\)
- Vậy tập nghiệm của bất phương trình là \(\left\{x|x< 3\right\}\)
b) Ta có: \(8x+3.\left(x+1\right)>5x-\left(2x-6\right)\)
\(\Leftrightarrow8x+3x+3>5x-2x+6\)
\(\Leftrightarrow8x+3x-5x+2x>6-3\)
\(\Leftrightarrow8x>3\)
\(\Leftrightarrow x>\frac{3}{8}\)
- Vậy tập nghiệm của bất phương trình là \(\left\{x|x>\frac{3}{8}\right\}\)
c) Ta có: \(\frac{1-2x}{4}-2\le\frac{1-5x}{8}\)
\(\Leftrightarrow\frac{1-2x-8}{4}\le\frac{1-5x}{8}\)
\(\Rightarrow2.\left(-2x-7\right)\le1-5x\)
\(\Leftrightarrow-4x-14\le1-5x\)
\(\Leftrightarrow-4x+5x\le1+14\)
\(\Leftrightarrow x\le15\)
- Vậy tập nghiệm của bất phương trình là \(\left\{x|x\le15\right\}\)
d) Ta có: \(\left(x+1\right)^2\ge\left(x-2\right).\left(x+3\right)-2\)
\(\Leftrightarrow x^2+2x+1\ge x^2+x-6-2\)
\(\Leftrightarrow\left(x^2-x^2\right)+\left(2x-x\right)\ge-6-2-1\)
\(\Leftrightarrow x\ge-9\)
- Vậy tập nghiệm của bất phương trình là \(\left\{x|x\ge-9\right\}\)
e) Ta có: \(\frac{2x-1}{x+1}>2\)
\(\Rightarrow2x-1>2.\left(x+1\right)\)
\(\Leftrightarrow2x-1>2x+2\)
\(\Leftrightarrow2x-2x>2+1\)
\(\Leftrightarrow0x>3\)( vô nghiệm )
- Vậy bất phương trình trên vô nghiệm
f) Ta có: \(\frac{3-8x}{2+2x} +4< 0\)
\(\Leftrightarrow\frac{3-8x}{2+2x}< -4\)
\(\Rightarrow3-8x< -4.\left(2+2x\right)\)
\(\Leftrightarrow3-8x< -8-8x\)
\(\Leftrightarrow-8x+8x< -8-3\)
\(\Leftrightarrow0x< -11\)( vô nghiệm )
- Vậy bất phương trình trên vô nghiệm
!!@@# ^_^ Chúc bạn hok tốt ^_^#@@!!

1a
x^2-8x<0
<=> x(x-8)<0
th1: x<0 và x-8>0
x<0 và x>8
<=> 8<x<0 ( vô lý)
th2: x>0 và x-8<0
<=> x>0 và x<8
<=> 0<x<8( tm)
vậy........
a) \(x^2-8x< 0\)
\(\Leftrightarrow x\left(x-8\right)< 0\)
\(\Leftrightarrow\hept{\begin{cases}x>0\\x-8< 0\end{cases}}\) hoặc \(\hept{\begin{cases}x< 0\\x-8>0\end{cases}}\)
\(\Leftrightarrow\hept{\begin{cases}x>0\\x< 8\end{cases}}\) hoặc \(\hept{\begin{cases}x< 0\\x>8\end{cases}}\) (loại)
\(\Leftrightarrow0< x< 8\)
b) \(x^2< 6x-5\)
\(\Leftrightarrow x^2-6x+5< 0\)
\(\Leftrightarrow x^2-x-5x+5< 0\)
\(\Leftrightarrow x\left(x-1\right)-5\left(x-1\right)< 0\)
\(\Leftrightarrow\left(x-1\right)\left(x-5\right)< 0\)
\(\Leftrightarrow\hept{\begin{cases}x-1>0\\x-5< 0\end{cases}}\) hoặc \(\hept{\begin{cases}x-1< 0\\x-5>0\end{cases}}\)
\(\Leftrightarrow\hept{\begin{cases}x>1\\x< 5\end{cases}}\) hoặc \(\hept{\begin{cases}x< 1\\x>5\end{cases}}\) (loại)
\(\Leftrightarrow1< x< 5\)
c) \(\frac{x-3}{x-2}< 0\)
\(\Leftrightarrow\hept{\begin{cases}x-3>0\\x-2< 0\end{cases}}\) hoặc \(\hept{\begin{cases}x-3< 0\\x-2>0\end{cases}}\)
\(\Leftrightarrow\hept{\begin{cases}x>3\\x< 2\end{cases}}\) (loại) hoặc \(\hept{\begin{cases}x< 3\\x>2\end{cases}}\)
\(\Leftrightarrow2< x< 3\)
d) \(\frac{x+1}{x-3}>2\) (ĐK: \(x\ne3\) )
\(\Leftrightarrow\frac{x+1}{x-3}-2>0\)
\(\Leftrightarrow\frac{x+1-2\left(x-3\right)}{x-3}>0\)
\(\Leftrightarrow\frac{-x+7}{x-3}>0\)
\(\Leftrightarrow\hept{\begin{cases}-x+7>0\\x-3>0\end{cases}}\) hoặc \(\hept{\begin{cases}-x+7< 0\\x-3< 0\end{cases}}\)
\(\Leftrightarrow\hept{\begin{cases}-x>-7\\x>3\end{cases}}\) hoặc \(\hept{\begin{cases}-x< -7\\x< 3\end{cases}}\)
\(\Leftrightarrow\hept{\begin{cases}x< 7\\x>3\end{cases}}\) hoặc \(\hept{\begin{cases}x>7\\x< 3\end{cases}}\) (loại)
\(\Leftrightarrow3< x< 7\)