Hãy nhập câu hỏi của bạn vào đây, nếu là tài khoản VIP, bạn sẽ được ưu tiên trả lời.

Lời giải:
Theo đề ta có: $\overrightarrow{BM}=2\overrightarrow{MC}=-2\overrightarrow{CM}$
$\overrightarrow{AM}=\overrightarrow{AB}+\overrightarrow{BM}(1)$
$=\overrightarrow{AB}-2\overrightarrow{CM}$
$\overrightarrow{AM}=\overrightarrow{AC}+\overrightarrow{CM}$
$\Rightarrow 2\overrightarrow{AM}=2\overrightarrow{AC}+2\overrightarrow{CM}(2)$
Lấy $(1)+(2)\Rightarrow 3\overrightarrow{AM}=\overrightarrow{AB}+2\overrightarrow{AC}$
$\Rightarrow \overrightarrow{AM}=\frac{1}{3}\overrightarrow{AB}+\frac{2}{3}\overrightarrow{AC}$

Ta có: \(\overrightarrow{MB}=3\overrightarrow{MC}\Rightarrow\overrightarrow{MB}=3\left(\overrightarrow{MB}+\overrightarrow{BC}\right)\)
\(\Rightarrow\overrightarrow{MB}=3\overrightarrow{MB}+3\overrightarrow{BC}\)
\(\Rightarrow-\overrightarrow{MB}=3\overrightarrow{BC}\)
\(\Rightarrow\overrightarrow{BM}=\dfrac{2}{3}\overrightarrow{BC}\). Mà \(\overrightarrow{BC}=\overrightarrow{AC}-\overrightarrow{AB}\) nên \(\overrightarrow{BM}=\dfrac{2}{3}\left(\overrightarrow{AC}-\overrightarrow{AB}\right)\)
Theo quy tắc 3 điểm, ta có
\(\overrightarrow{AM}=\overrightarrow{AB}+\overrightarrow{BM}\Rightarrow\overrightarrow{AM}=\overrightarrow{AB}+\dfrac{3}{2}\overrightarrow{AC}-\dfrac{3}{2}\overrightarrow{AB}\)
\(\Rightarrow\overrightarrow{AM}=-\dfrac{1}{2}\overrightarrow{AB}+\dfrac{3}{2}\overrightarrow{AC}\) hay \(\overrightarrow{AM}=-\dfrac{1}{2}\overrightarrow{u}+\dfrac{3}{2}\overrightarrow{v}\)

Câu 1:
vecto AM+vecto BN+vecto CP
=1/2(vecto AB+vecto AC+vecto BA+vecto BC+vecto CA+vecto CB)
=1/2*vecto 0
=vecto 0

a/ tự vẽ: M,A,B thẳng hàng, MA=2MB=> B là TĐ MA
N,A,C thẳng hàng, NA= 2CN/3
b/ tính cái j theo vecto AC và AB z?
c/ G là cái j
VT lại đề bài cái coi :))

A B C M N K D
Do K là trung điểm của MN nên \(\overrightarrow{AK}=\dfrac{1}{2}\left(\overrightarrow{AM}+\overrightarrow{AN}\right)=\dfrac{1}{2}\left(\dfrac{1}{2}\overrightarrow{AB}+\dfrac{2}{3}\overrightarrow{AC}\right)\)
\(=\dfrac{1}{4}\overrightarrow{AB}+\dfrac{1}{3}\overrightarrow{AC}\).
\(\overrightarrow{AD}=\dfrac{1}{2}\left(\overrightarrow{AB}+\overrightarrow{AC}\right)\).
\(\overrightarrow{KD}=\overrightarrow{KA}+\overrightarrow{AD}=\)\(-\dfrac{1}{4}\overrightarrow{AB}-\dfrac{1}{3}\overrightarrow{AC}+\dfrac{1}{2}\left(\overrightarrow{AB}+\overrightarrow{AC}\right)\)
\(=\dfrac{1}{4}\overrightarrow{AB}+\dfrac{1}{6}\overrightarrow{AC}\).

 = 3
= 3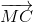 =>
=> 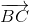 )
)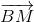 =
= 
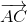 -
- 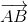 nên
nên 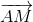 =
= 

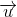 +
+ 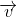
\(\overrightarrow{AB}+\overrightarrow{BC}=\overrightarrow{AC}\Rightarrow\overrightarrow{BC}=\overrightarrow{AC}-\overrightarrow{AB}=\overrightarrow{v}-\overrightarrow{u}\)
\(\overrightarrow{AM}=\overrightarrow{AB}+\overrightarrow{BM}=\overrightarrow{AB}+\frac{3}{4}\overrightarrow{BC}=\overrightarrow{u}+\frac{3}{4}\left(\overrightarrow{v}-\overrightarrow{u}\right)=\frac{1}{4}\overrightarrow{u}+\frac{3}{4}\overrightarrow{v}\)