Hãy nhập câu hỏi của bạn vào đây, nếu là tài khoản VIP, bạn sẽ được ưu tiên trả lời.


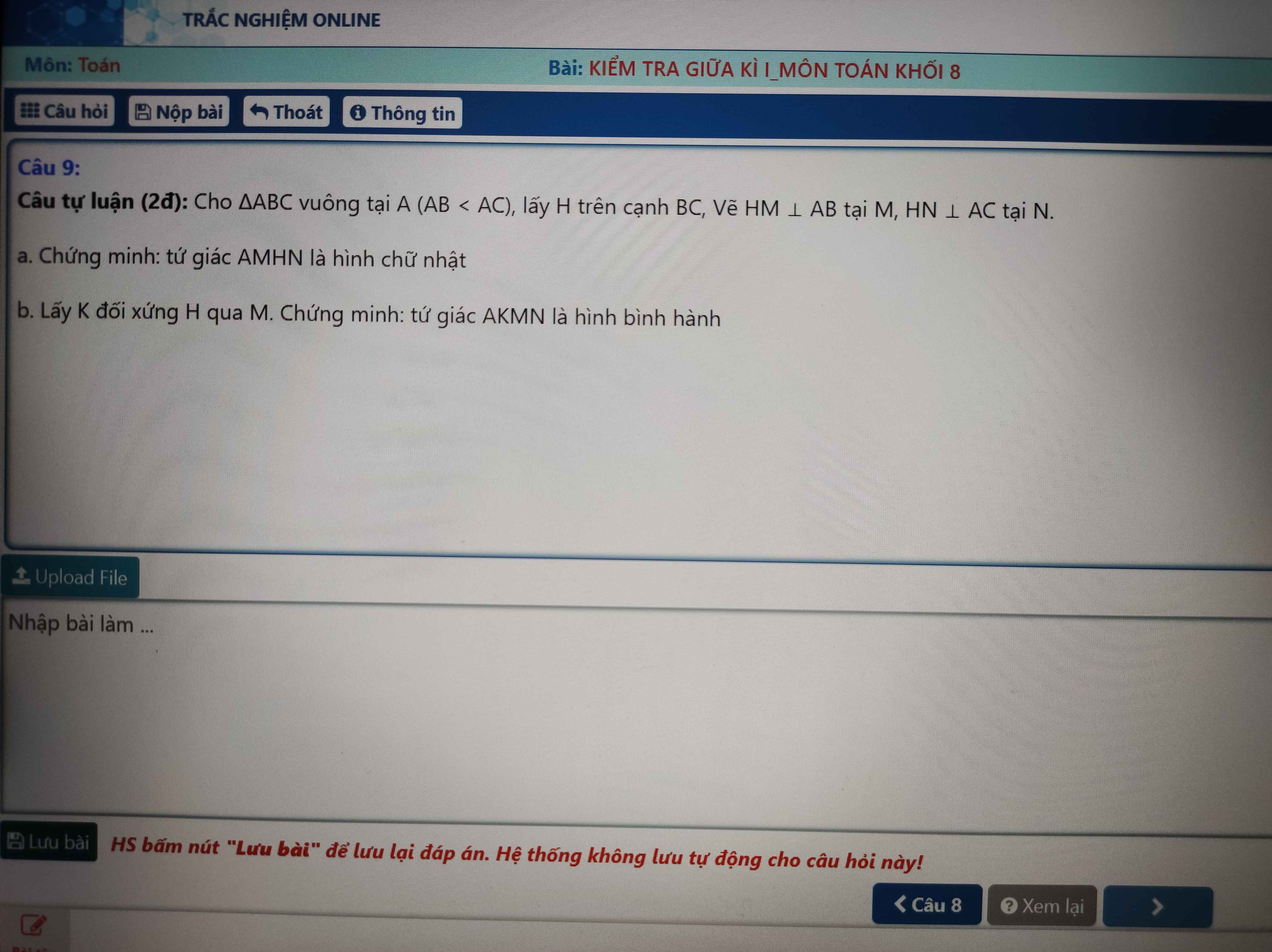
Câu 10:
a: ĐKXĐ: \(\left\{{}\begin{matrix}x\notin\left\{2;-1\right\}\\y\ne-5\end{matrix}\right.\)
\(A=\dfrac{y+5}{x^2-4x+4}\cdot\dfrac{x^2-4}{x+1}\cdot\dfrac{x-2}{y+5}\)
\(=\dfrac{y+5}{y+5}\cdot\dfrac{\left(x^2-4\right)}{x^2-4x+4}\cdot\dfrac{x-2}{x+1}\)
\(=\dfrac{\left(x^2-4\right)\cdot\left(x-2\right)}{\left(x+1\right)\left(x^2-4x+4\right)}\)
\(=\dfrac{\left(x+2\right)\left(x-2\right)\cdot\left(x-2\right)}{\left(x+1\right)\left(x-2\right)^2}=\dfrac{x+2}{x+1}\)
b: \(A=\dfrac{x+2}{x+1}\)
=>A không phụ thuộc vào biến y
Khi x=1/2 thì \(A=\left(\dfrac{1}{2}+2\right):\left(\dfrac{1}{2}+1\right)=\dfrac{5}{2}:\dfrac{3}{2}=\dfrac{5}{2}\cdot\dfrac{2}{3}=\dfrac{5}{3}\)
Câu 12:
a: \(A=\dfrac{x}{x+3}+\dfrac{2x}{x-3}+\dfrac{9-3x^2}{x^2-9}\)
\(=\dfrac{x}{x+3}+\dfrac{2x}{x-3}+\dfrac{9-3x^2}{\left(x+3\right)\left(x-3\right)}\)
\(=\dfrac{x\left(x-3\right)+2x\left(x+3\right)+9-3x^2}{\left(x+3\right)\left(x-3\right)}\)
\(=\dfrac{x^2-3x+2x^2+6x+9-3x^2}{\left(x+3\right)\left(x-3\right)}\)
\(=\dfrac{3x+9}{\left(x+3\right)\left(x-3\right)}=\dfrac{3\left(x+3\right)}{\left(x+3\right)\left(x-3\right)}=\dfrac{3}{x-3}\)
b: Khi x=1 thì \(A=\dfrac{3}{1-3}=\dfrac{3}{-2}=-\dfrac{3}{2}\)
\(x+\dfrac{1}{3}=\dfrac{10}{3}\)
=>\(x=\dfrac{10}{3}-\dfrac{1}{3}\)
=>\(x=\dfrac{9}{3}=3\left(loại\right)\)
Vậy: Khi x=3 thì A không có giá trị
c: \(B=A\cdot\dfrac{x-3}{x^2-4x+5}\)
\(=\dfrac{3}{x-3}\cdot\dfrac{x-3}{x^2-4x+5}\)
\(=\dfrac{3}{x^2-4x+5}\)
\(x^2-4x+5=x^2-4x+4+1=\left(x-2\right)^2+1>=1\forall x\) thỏa mãn ĐKXĐ
=>\(B=\dfrac{3}{x^2-4x+5}< =\dfrac{3}{1}=3\forall x\) thỏa mãn ĐKXĐ
Dấu '=' xảy ra khi x-2=0
=>x=2

a) \(\dfrac{A}{x-2}=\dfrac{x^2+3x+2}{x^2-4}\)
\(\Leftrightarrow\dfrac{A}{x-2}=\dfrac{\left(x+2\right)\left(x+1\right)}{\left(x-2\right)\left(x+2\right)}\)
\(\Leftrightarrow\dfrac{A}{x-2}=\dfrac{x+1}{x-2}\Leftrightarrow A=x+1\)
b) \(\dfrac{M}{x-1}=\dfrac{x^2+3x+2}{x+1}\)
\(\Leftrightarrow\dfrac{M}{x-1}=\dfrac{\left(x+1\right)\left(x+2\right)}{x+1}\)
\(\Leftrightarrow\dfrac{M}{x-1}=x+2\Leftrightarrow M=\left(x-1\right)\left(x+2\right)=x^2+x-2\)

a) Xét \(\Delta ABH\) và \(\Delta AKC\) có:
+ \(\widehat{BAH}=\widehat{CAK}\left(gt\right)\)
+ \(\widehat{AHB}=\widehat{ACK}\left(=90^o\right)\)
=> \(\Delta ABH\sim\Delta AKC\left(g-g\right)\) abc
=> \(\dfrac{AB}{AK}=\dfrac{AH}{AC}\) (2 cặp cạch tương ứng)
=> AB.AC = AK.AH
b) Gọi I là giao điểm của BC và AK
Có \(\Delta ABH\sim\Delta AKC\)
=> \(\widehat{ABH}=\widehat{AKC}\) (2 góc tương ứng)
hay \(\widehat{ABI}=\widehat{IKC}\)
Xét \(\Delta ABI\) và \(\Delta CKI\) có:
+ \(\widehat{ABI}=\widehat{IKC}\)
+ \(\widehat{AIB}=\widehat{CIK}\) (2 góc đối đỉnh)
=> \(\Delta ABI\sim\Delta CKI\left(g-g\right)\)
=> \(\dfrac{AI}{CI}=\dfrac{BI}{KI}\) (2 cặp cạnh tương ứng)
Xét \(\Delta AIC\) và \(\Delta BIK\) có:
\(+\dfrac{AI}{CI}=\dfrac{BI}{KI}\)
+ \(\widehat{AIC}=\widehat{BIK}\) (2 góc đối đỉnh)
=> \(\Delta AIC\sim\Delta BIK\left(c-g-c\right)\)
=> \(\widehat{IAC}=\widehat{IBK}\) (2 góc tương ứng)
=> \(\widehat{IBK}=\widehat{BAH}\)
Mà \(\widehat{BAH}+\widehat{ABH}=90^o\)
=> \(\widehat{ABH}+\widehat{IBK}=90^o=>\widehat{ABK}=90^o\)
Xét tứ giác ABKC có:
\(\widehat{ABK}+\widehat{ACK}+\widehat{BAC}+\widehat{BKC}=360^o\)
=> \(\widehat{BAC}+\widehat{BKC}=180^o\)

3) \(\sqrt{\left(x-2\right)\left(x+1\right)}\) thì (x-2)(x+1)>0
=> x2 -x-2>0
=> x2 - x - \(\dfrac{1}{2}\)- \(\dfrac{3}{2}\)>0
= (x+\(\dfrac{1}{4}\))2 - 3/2 >0
=> x+ 1/4>3/2
=> x>5/4
4) Có x đâu mà tìm bạn??

a: Xét tứ giác AMHN có
\(\widehat{AMH}=\widehat{ANH}=\widehat{NAM}=90^0\)
DO đó: AMHN là hình chữ nhật


\(\left(x-2y\right)\left(x^2+2xy+4y^2\right)-\left(x-y\right)\left(x^2+8y^2\right)\)
\(=x^3-8y^3-\left(x^3-x^2y+8xy^2-8y^3\right)\)
\(=x^3-8y^3-x^3+x^2y-8xy^2+8y^3\)
\(=x^2y-8xy^2\)
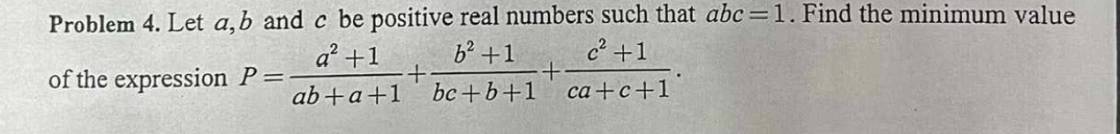

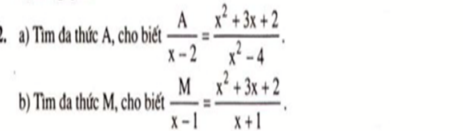 mọi người giải giúp em bài này với ạ em đang cần gấp ạ
mọi người giải giúp em bài này với ạ em đang cần gấp ạ