K
Khách
Hãy nhập câu hỏi của bạn vào đây, nếu là tài khoản VIP, bạn sẽ được ưu tiên trả lời.
Các câu hỏi dưới đây có thể giống với câu hỏi trên
PM
1
26 tháng 10 2021
Bài 3:
\(a,=\sqrt[3]{\left(x-1\right)^3}-\sqrt[3]{\left(5x+1\right)^3}=x-1-5x-1=-4x-2\\ b,=6a-6a+20a=20a\)
Bài 2:
\(a,=2\sqrt[3]{6}+3\sqrt[3]{5}-4\sqrt[3]{6}-2\sqrt[3]{5}=\sqrt[3]{5}-2\sqrt[3]{6}\\ b,=\sqrt[3]{8}-4\sqrt[3]{27}+2\sqrt[3]{64}=2-12+16=6\\ c,=\sqrt[3]{64}+\sqrt[3]{48}+\sqrt[3]{36}-\sqrt[3]{48}-\sqrt[3]{36}-\sqrt[3]{27}=4-3=1\\ d,=\sqrt[3]{162\left(-2\right)\cdot\dfrac{2}{3}}=\sqrt[3]{-216}=-6\)
PM
1
PM
1
19 tháng 10 2021
Bài 3:
a: Ta có: \(C=\dfrac{a^2+\sqrt{a}}{a-\sqrt{a}+1}-\dfrac{2a+\sqrt{a}}{\sqrt{a}}+1\)
\(=a+\sqrt{a}-2\sqrt{a}-1+1\)
\(=a-\sqrt{a}\)
b: Để C=2 thì \(\sqrt{a}-2=0\)
hay a=4
PM
1
6 tháng 10 2021
\(4,\\ a,ĐK:x>0;x\ne4;x\ne9\\ B=\dfrac{x+4\sqrt{x}+4-x+4\sqrt{x}-4+4x}{\left(2-\sqrt{x}\right)\left(2+\sqrt{x}\right)}\cdot\dfrac{\sqrt{x}\left(2-\sqrt{x}\right)}{\sqrt{x}-3}\\ B=\dfrac{4\sqrt{x}\left(\sqrt{x}+2\right)}{\left(2-\sqrt{x}\right)\left(2+\sqrt{x}\right)}\cdot\dfrac{\sqrt{x}\left(2-\sqrt{x}\right)}{\sqrt{x}-3}\\ B=\dfrac{4x}{\sqrt{x}-3}\)
\(b,B=1\Leftrightarrow4x=\sqrt{x}-3\Leftrightarrow4x-\sqrt{x}+3=0\\ \Leftrightarrow\left(4x-2\cdot2\cdot\dfrac{1}{4}\sqrt{x}+\dfrac{1}{16}\right)+\dfrac{47}{16}=0\\ \Leftrightarrow\left(2\sqrt{x}-\dfrac{1}{4}\right)^2+\dfrac{47}{16}=0\\ \Leftrightarrow x\in\varnothing\)
PM
2
17 tháng 9 2021
bài 1
\(\widehat{B}=90-\widehat{C}=90-30=60\)
\(sinC=\dfrac{AB}{BC}\Rightarrow BC=\dfrac{AB}{sinC}=\dfrac{30}{sin30}=60\)
áp dụng pytago vào \(\Delta ABC\)
\(AC=\sqrt{BC^2-AB^2}\)=\(\sqrt{60^2-30^2}\)=\(30\sqrt{3}\)=51,96
bài 2
\(\widehat{B}=90-\widehat{C}=90-30=60\)
\(sinC=\dfrac{AB}{BC}\Rightarrow AB=sinC.BC=sin30.5=2,5\)
áp
17 tháng 9 2021
áp dụng pytago vào \(\Delta ABC\)
\(AC=\sqrt{BC^2-AB^2}=\sqrt{5^2-2,5^2}\)=4,33
bài 3
\(\widehat{E}=90-\widehat{F}=90-47=43\)
\(sinF=\dfrac{ED}{EF}\Rightarrow EF=\dfrac{ED}{sinF}=\dfrac{9}{sin47}=12,31\)
áp dụng pytago vào \(\Delta DEF\)
\(DF=\sqrt{EF^2-ED^2}=\sqrt{12,31^2-9^2}\)=8,4
bài 4
áp dụng pytago vào \(\Delta ABC\)
\(AB=\sqrt{BC^2-AC^2}=\sqrt{32^2-27^2}=17,18\)
\(sinB=\dfrac{AC}{BC}=\dfrac{27}{32}\Rightarrow\widehat{B}=57\)
\(\widehat{C}=90-\widehat{B}=90-57=33\)
9 tháng 9 2021
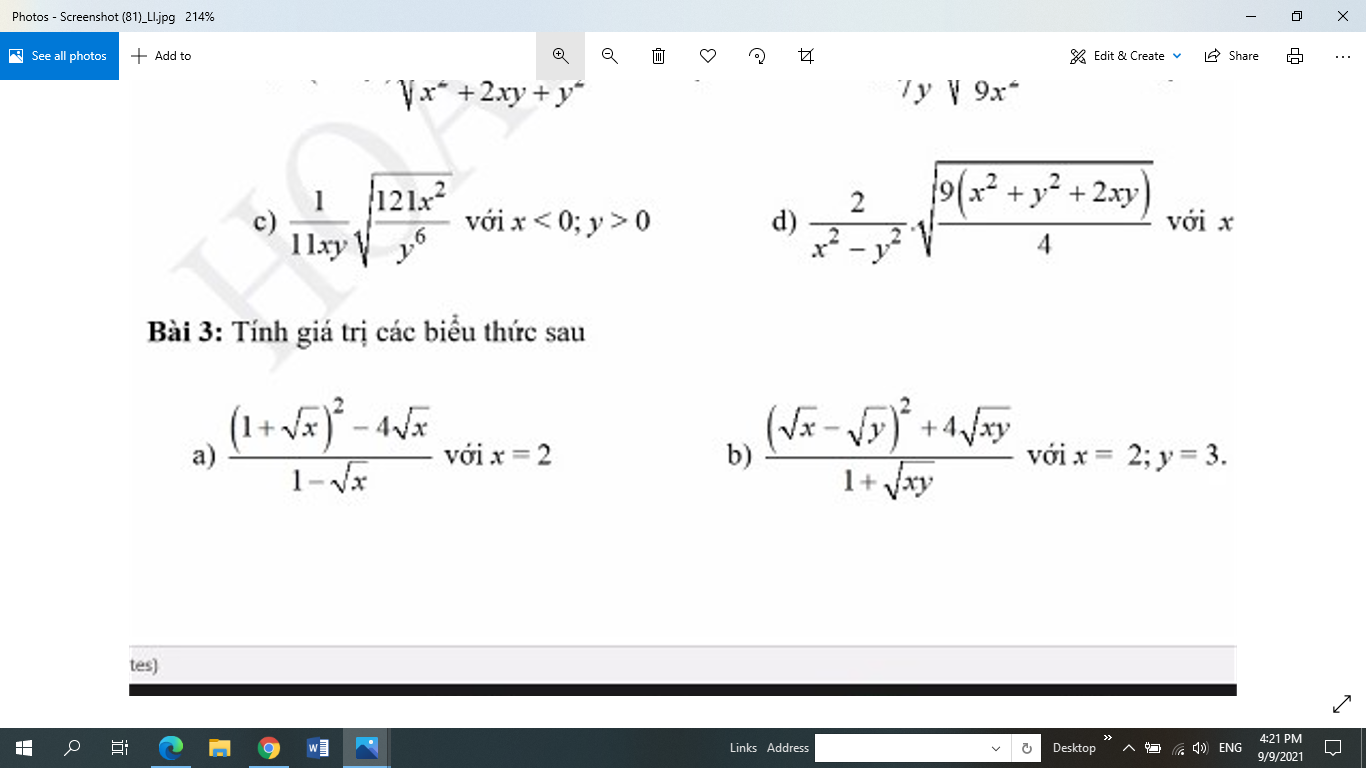
\(3,\\ a,\dfrac{\left(1+\sqrt{x}\right)^2-4\sqrt{x}}{1-\sqrt{x}}\\ =\dfrac{\sqrt{x}-2\sqrt{x}+1}{1-\sqrt{x}}=\dfrac{\left(1-\sqrt{x}\right)^2}{1-\sqrt{x}}=1-\sqrt{x}=1-\sqrt{2}\)
\(b,\dfrac{\left(\sqrt{x}-\sqrt{y}\right)^2+4\sqrt{xy}}{1+\sqrt{xy}}\\ =\dfrac{x+2\sqrt{xy}+y}{1+\sqrt{xy}}=\dfrac{\left(\sqrt{x}+\sqrt{y}\right)^2}{1+\sqrt{xy}}\\ =\dfrac{\left(\sqrt{2}+\sqrt{3}\right)^2}{1+\sqrt{6}}=\dfrac{5+2\sqrt{6}}{1+\sqrt{6}}\\ =\dfrac{\left(5+2\sqrt{6}\right)\left(\sqrt{6}-1\right)}{5}\\ =\dfrac{3\sqrt{6}+7}{5}\)
PM
2
B
22 tháng 8 2021
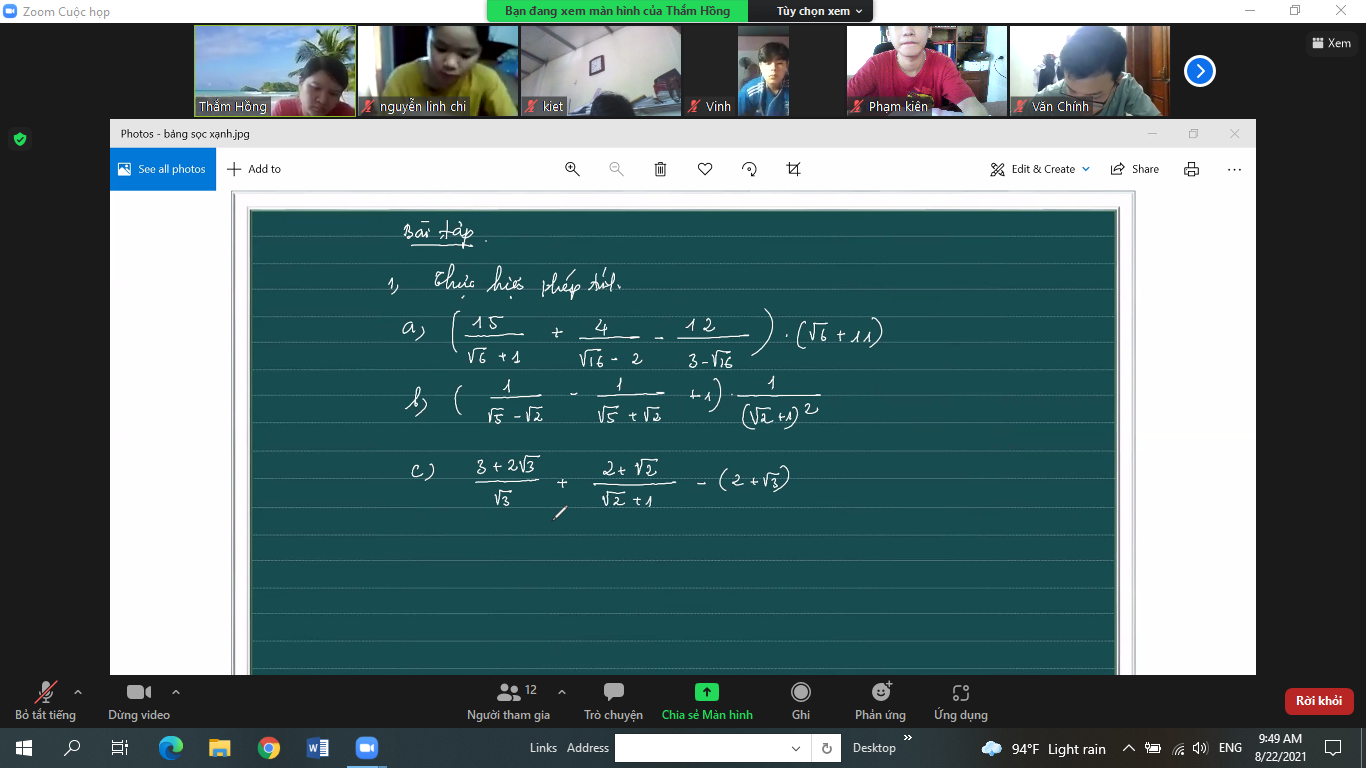
a)
=15(can6-1)/(6-1)+4/(4-2)-12/(3-4)
=3(can6-1)+2+12
=3\(\sqrt{6}\)-3+2+12
=17+3can6
các câu còn lại tương tự liên hợp mẫu
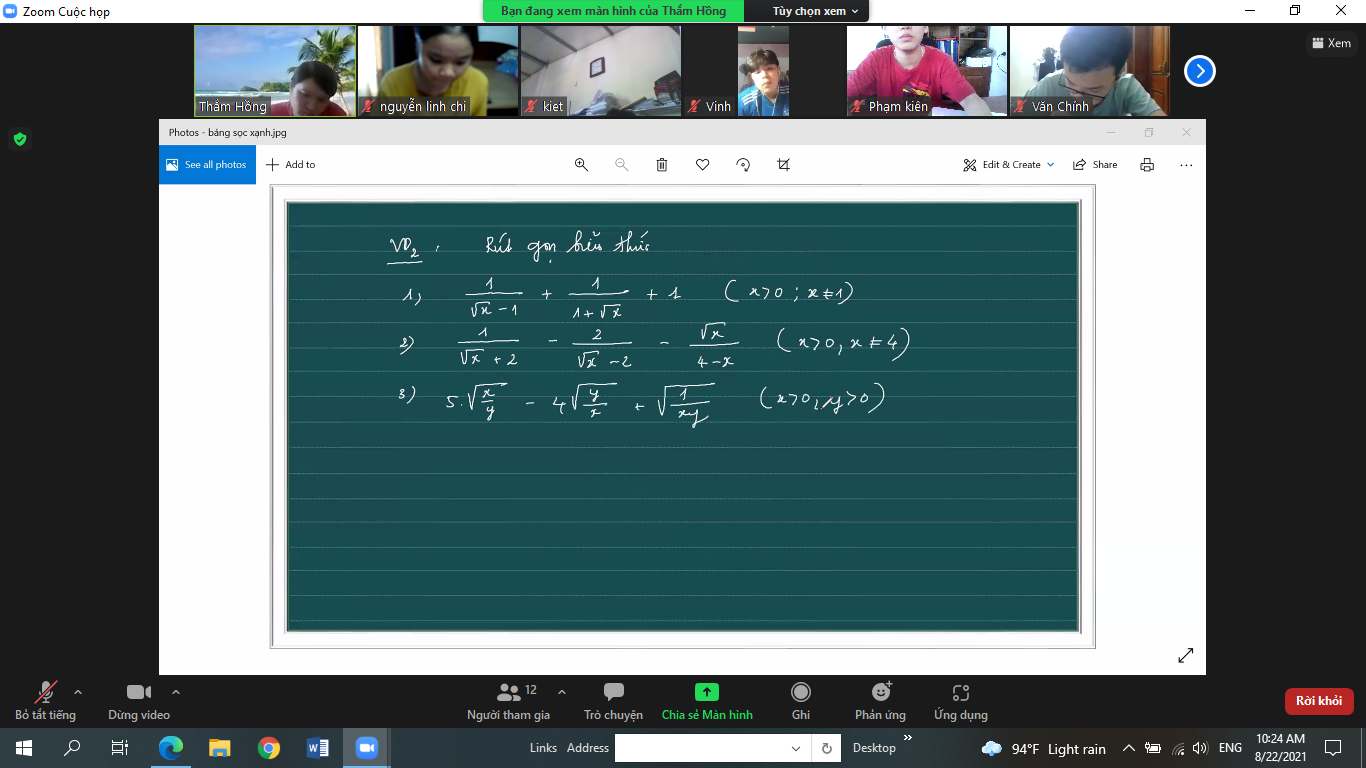
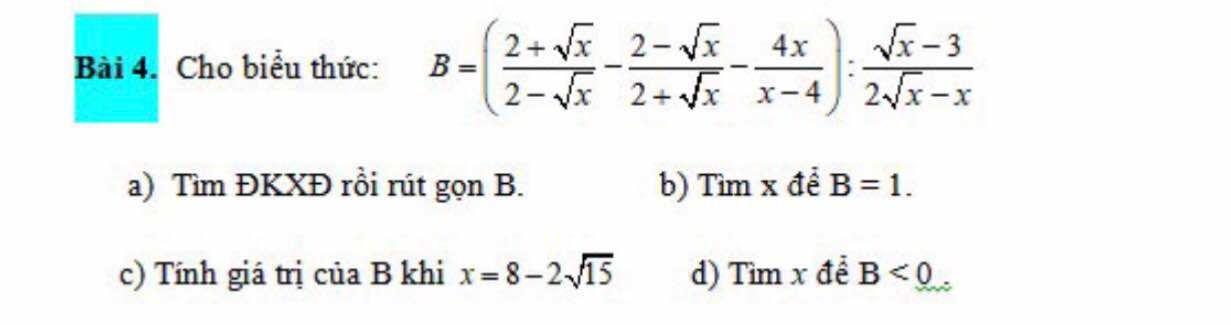
1: Ta có: \(\dfrac{1}{\sqrt{x}-1}+\dfrac{1}{\sqrt{x}+1}+1\)
\(=\dfrac{\sqrt{x}+1+\sqrt{x}-1+x-1}{\left(\sqrt{x}-1\right)\left(\sqrt{x}+1\right)}\)
\(=\dfrac{x+2\sqrt{x}-1}{x-1}\)