
Hãy nhập câu hỏi của bạn vào đây, nếu là tài khoản VIP, bạn sẽ được ưu tiên trả lời.



a: Khi m=3 thì (1): x^2-6x+4=0
=>x^2-6x+9-5=0
=>(x-3)^2=5
=>\(x=3\pm\sqrt{5}\)

\(1,\\ 1,M=6+5=11\\ N=\sqrt{5}-1-\sqrt{5}=-1\\ 2,\\ a,P=1+\dfrac{x-\sqrt{x}}{\sqrt{x}-1}\left(x\ge0;x\ne1\right)\\ P=1+\dfrac{\sqrt{x}\left(\sqrt{x}-1\right)}{\sqrt{x}-1}=1+\sqrt{x}\\ b,P>3\Leftrightarrow1+\sqrt{x}>3\\ \Leftrightarrow\sqrt{x}>2\Leftrightarrow x>4\)

10: =>1/2x=3/4 và x+y=2
=>x=3/4*2=3/2 và y=1/2
11:=>4x+5y=3 và 4x-12y=20
=>17y=-17 và x-3y=5
=>y=-1 và x=3y+5=-3+5=2
12: =>7x-2y=1 và 6x+2y=12
=>13x=13 và 3x+y=6
=>x=1 và y=3
13:=>2/x=1 và 1/x-1/y=1/5
=>x=2 và 1/y=1/2-1/5=3/10
=>y=10/3 và x=2
14: =>12/x-16/y=8 và 12/x-15/y=9
=>-1/y=-1 và 4/x-5/y=3
=>y=1 và 4/x=3+5=8
=>x=1/2 và y=1

a.
Đặt \(\left\{{}\begin{matrix}\sqrt{x^2+2x+3}=a>0\\\sqrt{x^2+x+2}=b>0\end{matrix}\right.\) \(\Rightarrow a^2-b^2=x+1\)
Pt trở thành:
\(a+b=2\left(a^2-b^2\right)\)
\(\Leftrightarrow a+b=\left(2a-2b\right)\left(a+b\right)\)
\(\Leftrightarrow2a-2b=1\) (do \(a+b>0\))
\(\Leftrightarrow2a=2b+1\)
\(\Leftrightarrow2\sqrt{x^2+2x+3}=2\sqrt{x^2+x+2}+1\)
\(\Leftrightarrow4\left(x^2+2x+3\right)=4\left(x^2+x+2\right)+1+4\sqrt{x^2+x+2}\)
\(\Leftrightarrow4x+3=4\sqrt{x^2+x+2}\)
\(\Leftrightarrow\left\{{}\begin{matrix}x\ge-\dfrac{3}{4}\\16\left(x^2+x+2\right)=\left(4x+3\right)^2\end{matrix}\right.\)
\(\Leftrightarrow\left\{{}\begin{matrix}x\ge-\dfrac{3}{4}\\8x=23\end{matrix}\right.\) \(\Rightarrow x=\dfrac{23}{8}\)
b.
ĐKXĐ: \(x\ge3\)
Đặt \(\left\{{}\begin{matrix}\sqrt{x-3}=a\ge0\\\sqrt{x+2}=b>0\end{matrix}\right.\) \(\Rightarrow a^2-b^2=-5\)
Phương trình trở thành:
\(\left(a-b\right)\left(ab+1\right)=a^2-b^2\)
\(\Leftrightarrow\left(a-b\right)\left(ab+1\right)=\left(a-b\right)\left(a+b\right)\)
\(\Leftrightarrow\left[{}\begin{matrix}a=b\left(vô-nghiệm\right)\\ab+1=a+b\end{matrix}\right.\)
\(\Rightarrow ab-a-b+1=0\)
\(\Leftrightarrow\left(a-1\right)\left(b-1\right)=0\)
\(\Rightarrow\left[{}\begin{matrix}a=1\\b=1\end{matrix}\right.\)
\(\Rightarrow\left[{}\begin{matrix}\sqrt{x-3}=1\\\sqrt{x+2}=1\end{matrix}\right.\)
\(\Rightarrow\left[{}\begin{matrix}x=4\\x=-1\left(ktm\right)\end{matrix}\right.\)

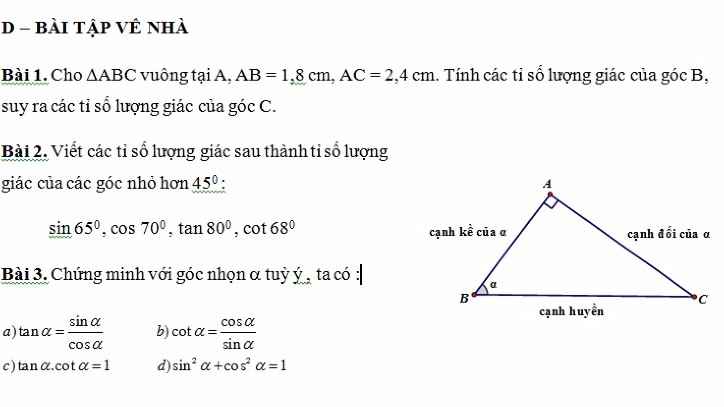
Bài 2:
\(\sin65^0=\cos25^0\)
\(\cos70^0=\sin20^0\)
\(\tan80^0=\cot10^0\)
\(\cot68^0=\tan22^0\)

\(a,\sqrt{-5x-10}\) có nghĩa \(\Leftrightarrow-5x-10\ge0\Leftrightarrow-5x\ge10\Leftrightarrow x\le-2\)
\(b,\sqrt{\dfrac{-2}{3x-1}}\) có nghĩa \(\Leftrightarrow\dfrac{-2}{3x-1}\ge0\Leftrightarrow3x-1< 0\Leftrightarrow x< \dfrac{1}{3}\)
\(c,\sqrt{\dfrac{2x-3}{2x^2+1}}\) có nghĩa \(\Leftrightarrow\left\{{}\begin{matrix}2x-3\ge0\\2x^2+1>0\end{matrix}\right.\)
\(\Leftrightarrow\left\{{}\begin{matrix}2x\ge3\\2x^2>-1\end{matrix}\right.\)
\(\Leftrightarrow\left\{{}\begin{matrix}x\ge\dfrac{3}{2}\\x^2>-\dfrac{1}{2}\left(loại\right)\end{matrix}\right.\)
\(\Leftrightarrow x\ge\dfrac{3}{2}\)
\(d,\sqrt{\dfrac{3x-2}{x^2-2x+4}}\) có nghĩa \(\Leftrightarrow\left\{{}\begin{matrix}3x-2\ge0\\x^2-2x+4>0\end{matrix}\right.\)
\(\Leftrightarrow3x\ge2\)
\(\Leftrightarrow x\ge\dfrac{2}{3}\)
\(e,\sqrt{x^2-8x-9}\) có nghĩa \(\Leftrightarrow x^2-8x-9\ge0\)
\(\Leftrightarrow x^2+x-9x-9\ge0\)
\(\Leftrightarrow x\left(x+1\right)-9\left(x+1\right)\ge0\)
\(\Leftrightarrow\left(x-9\right)\left(x+1\right)\ge0\)
\(\Leftrightarrow\left[{}\begin{matrix}\left\{{}\begin{matrix}x-9\ge0\\x+1\ge0\end{matrix}\right.\\\left\{{}\begin{matrix}x-9\le0\\x+1\le0\end{matrix}\right.\end{matrix}\right.\)
\(\Leftrightarrow\left[{}\begin{matrix}\left\{{}\begin{matrix}x\ge9\\x\ge-1\end{matrix}\right.\\\left\{{}\begin{matrix}x\le9\\x\le-1\end{matrix}\right.\end{matrix}\right.\)
\(\Leftrightarrow\left\{{}\begin{matrix}x\ge9\\x\le-1\end{matrix}\right.\)
\(f,\sqrt{\dfrac{2x-4}{5-x}}\) có nghĩa \(\Leftrightarrow\left\{{}\begin{matrix}2x-4\ge0\\5-x>0\end{matrix}\right.\)
\(\Leftrightarrow\left\{{}\begin{matrix}x\ge2\\x< 5\end{matrix}\right.\)
a: ĐKXĐ: -5x-10>=0
=>x<=-2
b: ĐKXĐ: 3x-1<0
=>x<1/3
c: ĐKXĐ: 2x-3>=0
=>x>=3/2
e: ĐKXĐ: (x-9)(x+1)>=0
=>x>=9 hoặc x<=-1
d: ĐKXĐ: 3x-2>=0
=>x>=2/3

Bài 9:
a: Xét tứ giác OPMN có
góc OPM+góc ONM=180 độ
=>OPMN là tứ giác nội tiếp
b: \(MN=\sqrt{10^2-6^2}=8\left(cm\right)\)
c: ΔOAB cân tại O
mà OH là đường trung tuyến
nên OH vuông góc AB
Xét tứ giác OHNM có
góc OHM=goc ONM=90 độ
=>OHNM là tứ giác nội tiép
=>góc MHN=góc MON





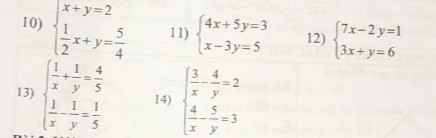
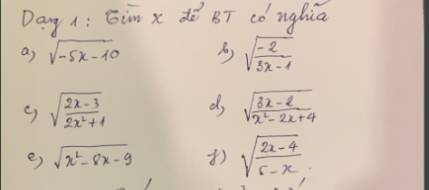

2.5
Thay x=1 và y=2 vào y=ax+5, ta được:
a+5=2
hay a=-3
Câu này mk lm r nha!
Cũng xin cảm ơn bn đã giúp mk nha.Cảm ơn nhìu🥰