Hãy nhập câu hỏi của bạn vào đây, nếu là tài khoản VIP, bạn sẽ được ưu tiên trả lời.

Ta có:
\(r^2+p^2+4Rr=\left(\dfrac{S}{p}\right)^2+p^2+\dfrac{abc}{S}.\dfrac{S}{p}\)
\(=\dfrac{\left(p-a\right)\left(p-b\right)\left(p-c\right)}{p}+p^2+\dfrac{abc}{p}\)
\(=\dfrac{p^3+\left(ab+bc+ac\right)p-p^2\left(a+b+c\right)-abc+p^3+abc}{p}\)
\(=ab+bc+ca\)
Do đó:
\(\dfrac{ab+bc+ca}{4R^2}=\dfrac{r^2+p^2+4Rr}{4R^2}\)
\(\Leftrightarrow sinAsinB+sinBsinC+sinCsinA=\dfrac{r^2+p^2+4Rr}{4R^2}\)\(\left(đpcm\right)\)
bạn giải thích chi tiết đoạn này hộ mình được ko ạ
p^3+(ab+bc+ac)p−p^2(a+b+c)−abc+p^3+abc/p
=ab+bc+ca

1.
\(sinA+sinB-sinC=2sin\dfrac{A+B}{2}.cos\dfrac{A-B}{2}-sin\left(A+B\right)\)
\(=2sin\dfrac{A+B}{2}.cos\dfrac{A-B}{2}-2sin\dfrac{A+B}{2}.cos\dfrac{A+B}{2}\)
\(=2sin\dfrac{A+B}{2}.\left(cos\dfrac{A-B}{2}-cos\dfrac{A+B}{2}\right)\)
\(=2sin\dfrac{A+B}{2}.2sin\dfrac{A}{2}.sin\dfrac{B}{2}\)
\(=4sin\dfrac{A}{2}.sin\dfrac{B}{2}.cos\dfrac{C}{2}\)
Sao t lại đc như này v, ai check hộ phát

\(S=sinx+siny+sin\left(3x+y\right)-sin\left(3x+y\right)-sin\left(x+y\right)\)
\(=sinx+siny-sin\left(x+y\right)\)
\(S^2=\left(sinx+siny-sin\left(x+y\right)\right)^2\le3\left(sin^2x+sin^2y+sin^2\left(x+y\right)\right)\)
\(S^2\le3\left(1-\dfrac{1}{2}\left(cos2x+cos2y\right)+sin^2\left(x+y\right)\right)\)
\(S^2\le3\left[1-cos\left(x+y\right)cos\left(x-y\right)+1-cos^2\left(x-y\right)\right]\)
\(S^2\le3\left[2+\dfrac{1}{4}cos^2\left(x+y\right)-\left[cos\left(x-y\right)-\dfrac{1}{2}cos\left(x+y\right)\right]^2\right]\le3\left[2+\dfrac{1}{4}cos^2\left(x+y\right)\right]\)
\(S^2\le3\left(2+\dfrac{1}{4}\right)=\dfrac{27}{4}\)
\(\Rightarrow S\le\dfrac{3\sqrt{3}}{2}\)
\(\Rightarrow\left\{{}\begin{matrix}a=3\\b=3\\c=2\end{matrix}\right.\)

- Áp dụng định lý sin ta được :
\(\dfrac{a}{sinA}=\dfrac{b}{sinB}=\dfrac{c}{sinC}=2R\)
\(\Rightarrow\left\{{}\begin{matrix}sinC=\dfrac{c}{2R}\\sinB=\dfrac{b}{2R}\\sinA=\dfrac{a}{2R}\end{matrix}\right.\)
VT = \(\dfrac{a^2}{2R}+\dfrac{b^2}{2R}+\dfrac{c^2}{2R}=\dfrac{a^2+b^2+c^2}{2R}\)
Lại có \(\left\{{}\begin{matrix}m_a^2=\dfrac{b^2+c^2}{2}-\dfrac{a^2}{4}\\....\end{matrix}\right.\)
\(\Rightarrow VP=\dfrac{b^2+c^2+c^2+a^2+a^2+b^2-\dfrac{a^2}{2}-\dfrac{b^2}{2}-\dfrac{c^2}{2}}{3R}\)
\(=\dfrac{\dfrac{3}{2}\left(a^2+b^2+c^2\right)}{3R}=\dfrac{a^2+b^2+c^2}{2R}=VT\)
=> ĐPCM
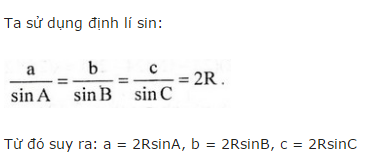
a)