
Hãy nhập câu hỏi của bạn vào đây, nếu là tài khoản VIP, bạn sẽ được ưu tiên trả lời.


\(\dfrac{1}{\sqrt[3]{4}+\sqrt[3]{2}+1}=\dfrac{\sqrt[3]{2}-1}{\left(\sqrt[3]{2}-1\right)\left(\sqrt[3]{4}+\sqrt[3]{2}+1\right)}\)
\(=\dfrac{\sqrt[3]{2}-1}{2-1}=\sqrt[3]{2}-1\)


1/2x^2=4-x
=>x^2=8-2x
=>x^2+2x-8=0
=>(x+4)(x-2)=0
=>x=2; x=-4

1) Với x=4 thì
\(A=\dfrac{2\sqrt{4}}{\sqrt{4}+3}=\dfrac{4}{2+3}=\dfrac{4}{5}\)
2) \(P=A+B\)
\(=\dfrac{2\sqrt{x}}{\sqrt{x}+3}+\dfrac{\sqrt{x}+1}{\sqrt{x}-3}+\dfrac{11\sqrt{x}-3}{x-9}\)
\(=\dfrac{2\sqrt{x}\left(\sqrt{x}-3\right)+\left(\sqrt{x}+1\right)\left(\sqrt{x}+3\right)+11\sqrt{x}-3}{\left(\sqrt{x}-3\right)\left(\sqrt{x+3}\right)}\)
\(=\dfrac{2x-6\sqrt{x}+x+4\sqrt{x}+3+11\sqrt{x}-3}{\left(\sqrt{x}+3\right)\left(\sqrt{x}-3\right)}\)
\(=\dfrac{3x+9\sqrt{x}}{\left(\sqrt{x}-3\right)\left(\sqrt{x}+3\right)}=\dfrac{3\sqrt{x}\left(\sqrt{x}+3\right)}{\left(\sqrt{x}-3\right)\left(\sqrt{x}+3\right)}\)
\(=\dfrac{3\sqrt{x}}{\sqrt{x}-3}\)
3) Để P< 3 thì
\(\dfrac{3\sqrt{x}}{\sqrt{x}-3}< 3\)
\(\Leftrightarrow\dfrac{3\sqrt{x}}{\sqrt{x}-3}-\dfrac{3\left(\sqrt{x}-3\right)}{\sqrt{x}-3}< 0\)
\(\Leftrightarrow\dfrac{9}{\sqrt{x}-3}< 0\)
\(\Rightarrow\sqrt{x}-3< 0\) ( vì 9>0)
<=> x<9
Vậy giá trị nguyên lớn nhất của x để P <3 là 8

Do pt có 2 nghiệm \(x_1,x_2\) nên ta có :
\(\left\{{}\begin{matrix}S=x_1+x_2=-\dfrac{b}{a}=-\dfrac{5}{2}\\P=x_1x_2=\dfrac{c}{a}=-\dfrac{1}{2}\end{matrix}\right.\)
Ta có :
\(P=x_1\left(3+x_2\right)+x_2\left(3+x_1\right)+3x^2_1+3x^2_2-10\)
\(=3x_1+x_1x_2+3x_2+x_1x_2+3\left(x_1^2+x_2^2\right)-10\)
\(=3\left(x_1+x_2\right)+2x_1x_2+3\left(x^2_1+x^2_2\right)-10\)
\(=3S+2P+3\left(S^2-2P\right)-10\)
\(=3.\left(-\dfrac{5}{2}\right)+2.\left(-\dfrac{1}{2}\right)+3\left(\left(-\dfrac{5}{2}\right)^2-2\left(-\dfrac{1}{2}\right)\right)-10\)
\(=\dfrac{13}{4}\)
Vậy \(P=\dfrac{13}{4}\)


\(\left\{{}\begin{matrix}x\ne-\dfrac{5}{3}\\-\dfrac{2}{3x+5}>0\end{matrix}\right.\Leftrightarrow\left\{{}\begin{matrix}x\ne-\dfrac{5}{3}\\3x+5< 0\end{matrix}\right.\Leftrightarrow\left\{{}\begin{matrix}x\ne-\dfrac{5}{3}\\x< -\dfrac{5}{3}\end{matrix}\right.\Leftrightarrow x< -\dfrac{5}{3}\)

\(tanx+\frac{cosx}{1+sinx}=\frac{sinx}{cosx}+\frac{cosx}{1+sinx}=\frac{sinx+sin^2x+cos^2x}{\left(1+sinx\right)cosx}=\frac{1+sinx}{\left(1+sinx\right)cosx}=\frac{1}{cosx}\)
\(tanx+\frac{cosx}{1+sinx}\)
\(=\frac{cosx}{1+sinx}+\frac{sinx}{cosx}\)
\(=\frac{cos^2x}{cosx.\left(sinx+1\right)}+\frac{sinx.\left(sinx+1\right)}{cosx.\left(sinx+1\right)}\)
\(=\frac{cos^2x+sinx.\left(sinx+1\right)}{cosx.\left(sinx+1\right)}\)
\(=\frac{1-sin^2x+\left(1+sinx\right)sinx}{\left(1+sinx\right).cosx}\)
\(=\frac{sinx+1}{cosx.\left(sinx+1\right)}\)
\(=\frac{1}{cosx}\)

`1/((sqrtx-1)(sqrtx+2))-1/((sqrtx-1)(3-sqrtx))`
`=1/((sqrtx-1)(sqrtx+2))+1/((sqrtx-1)(sqrtx-3))`
`=(sqrtx-3+sqrtx+2)/((sqrtx-1)(sqrtx+2)(sqrtx-3))`
`=(2sqrtx-1)/((sqrtx-1)(sqrtx+2)(sqrtx-3))`
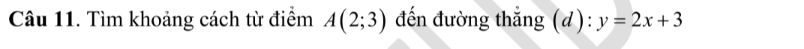
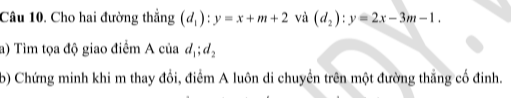 ai giup mik vs :<
ai giup mik vs :< 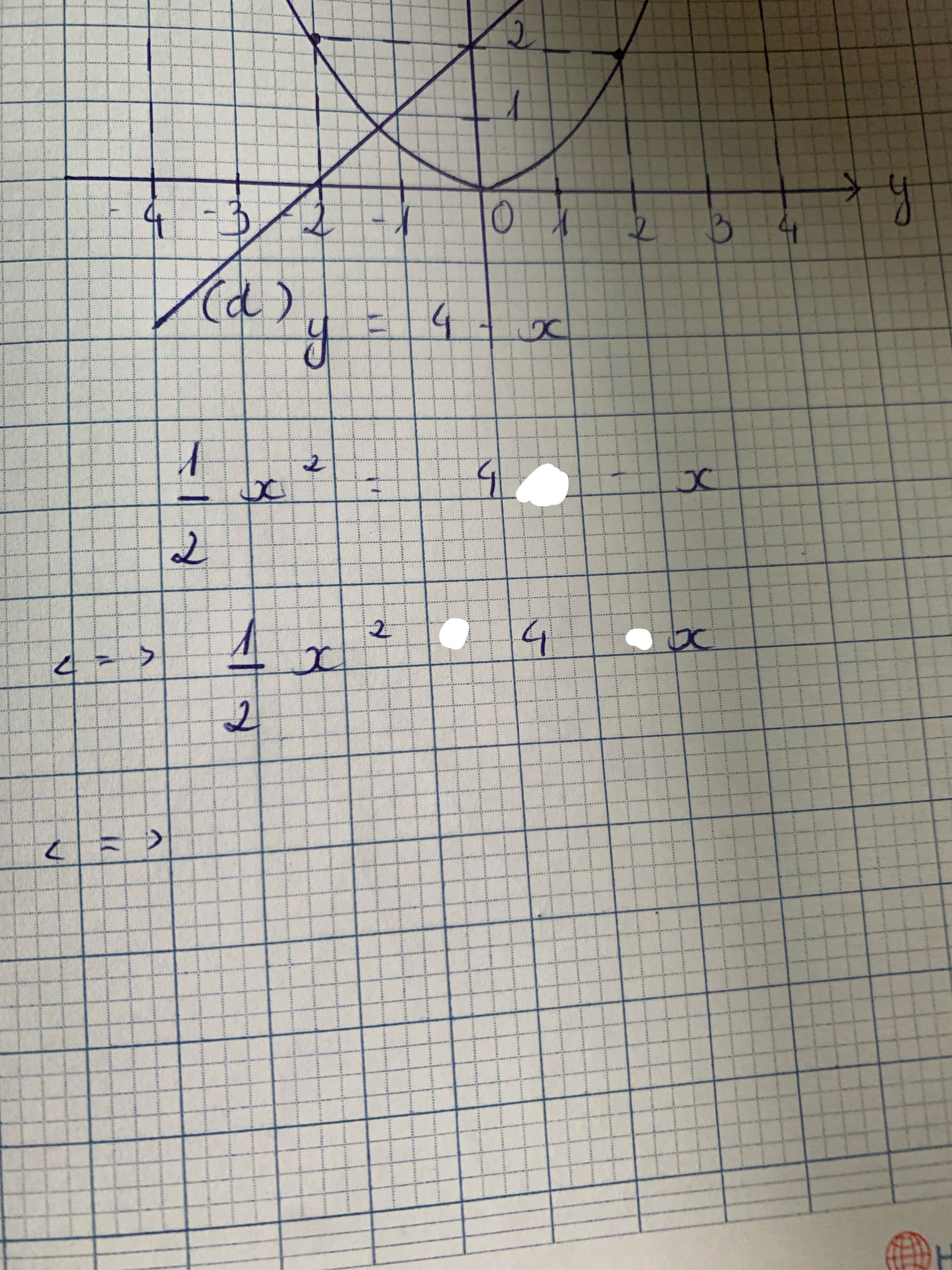

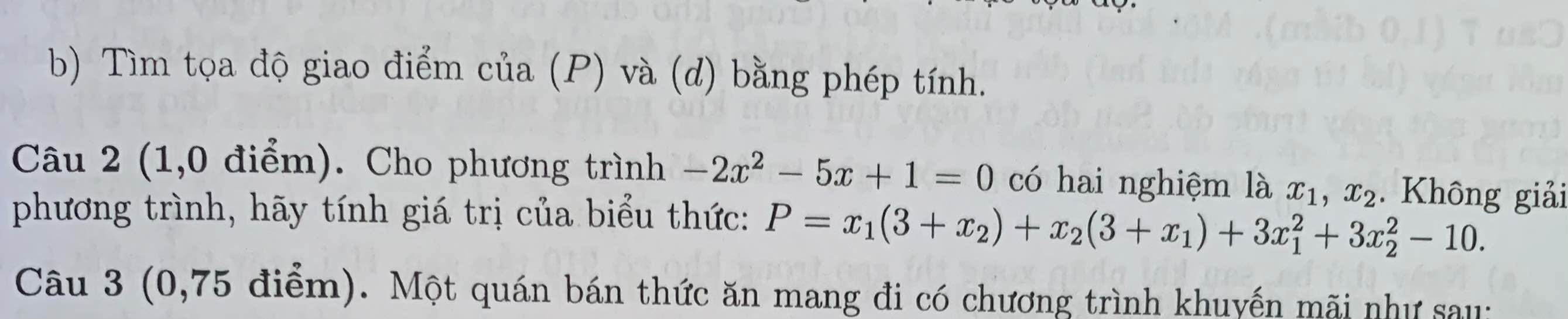

ai giup vs huhu