Hãy nhập câu hỏi của bạn vào đây, nếu là tài khoản VIP, bạn sẽ được ưu tiên trả lời.

Đk để pt trên có 2 nghiệm phân biệt x1,x2 : a>0 và denta>0
suy ra denta= (2m+1)^2-4.(m^2+1)>0
suy ra : m>3/4
Ta có P=x1x2/x1+x2=(m^2+1)/(2m+1)
Ta có: P∈Z
⇒4P∈Z
⇒(4m^2+4)/2m+1=(2m-1)+5/2m+1∈Z
⇒2m+1=Ư(5)={−5;−1;1;5}
⇒m={−3;−1;0;2}
Kết hợp đk m>3/4 ta được m=2

Để phương trình có hai nghiệm phân biệt âm :
\(\left\{{}\begin{matrix}\Delta>0\\S< 0\\P>0\end{matrix}\right.\Leftrightarrow\left\{{}\begin{matrix}\left(m^2-1\right)^2-9>0\left(1\right)\\\dfrac{-2\left(m^2-1\right)}{9.2}< 0\left(2\right)\\\dfrac{1}{9}>0\end{matrix}\right.\)\(\Leftrightarrow\left\{{}\begin{matrix}\left(m^2-1\right)^2>9\\m^2-1>0\end{matrix}\right.\)
Với \(m>2\) thì \(\left(m^2-1\right)^2-9>\left(2^2-1\right)^2-9=0\) nên (1) thỏa mãn.
Với \(m>2\) thì \(m^2-1>2^2-1=3>0\) nên (2) thỏa mãn.
Vậy \(m>2\) phương trình có hai nghiệm âm.
Để phương trình có hai nghiệm thì:
\(\left\{{}\begin{matrix}a\ne0\\\Delta\ge0\end{matrix}\right.\)\(\Leftrightarrow\left\{{}\begin{matrix}\left(m^2-1\right)^2-9\ge0\\9\ne0\end{matrix}\right.\)
Áp dụng định lý Viet ta được:
\(x_1+x_2=\dfrac{-2\left(m^2-1\right)}{9}=4\) \(\Leftrightarrow m^2-1=-18\)
\(\Leftrightarrow m^2=-17\) (loại)
Vậy không có giá trị m thỏa mãn.

a) \(\Delta\)=\((m)^{2} -4(m-2)=m^2-4m+8=(m-2)^2+4 >0\)với mọi m \(\Rightarrow\)pt (1) luôn có nghiệm phân biệt với mọi m.
b)Do pt (1) có 2 ng pb với mọi m \(\Rightarrow\)áp dụng Vi_et ta có:
\(\begin{cases} x1+x2=m\\ x1.x2=m-2\end{cases}\).Pt (1) trở thành :
\(2[(x1+x2)^2-2x1.x2]-x1.x2=2(m-\frac{5}{4})^2+\frac{55}{8} \geq \frac{55}{8}\)với mọi m. GTNN của (1) là 55/8 khi và chỉ khi m=5/4

a) \(det=\left|\begin{matrix}1&-m\\m&1\end{matrix}\right|=1+m^2\ne0\) với mọi m => Hệ phương trình bậc nhất hai ẩn luôn có nghiệm
b) Ta có:
x0 - my0 = 2 - 4m
mx0 + y0 = 3m + 1
Hay là:
x0 - 2 = m (y0 - 4)
y0 - 1 = m (3 - x0)
=> Chia hai vế cho nhau ta được
\(\frac{x_0-2}{y_0-1}=\frac{y_0-4}{3-x_0}\)
=> (x0 - 2)(3 - x0) = (y0 - 4)(y0 - 1)
=> -x02 + 5x0 - 6 = y02 - 5y0 + 4
=> x02 + y02 - 5(x0 + y0) = -10
ĐPCM

để pt có 2 nghiệm phân biệt thì: đenta > 0
mà ddeenta = m2 - 6m - 7 > 0
giải ra ta đc: m<-1 hay m>7 (1)
áp dụng hệ thức vi-et đc x1 + x2 = m-1 và x1.x2= m+2
kết 2 biểu thức trên dễ dàng làm đc x12 + x22 = m2-4m-3
bđt trên (=) (x12+x22)/x12.x22 - 1 > 0
thay vào đc (-16m -7)/(m2+4m+4) > 0 =) m khác -2 và m<-7/16
kết hợp vs (1) =) m<-1 và m khác -2

\(\Delta=\left(3m+2\right)^2-12m=9m^2+4>0\)
Theo hệ thức Viet: \(\left\{{}\begin{matrix}x_1+x_2=-3m-2\\x_1x_2=3m\end{matrix}\right.\)
\(\Leftrightarrow\left\{{}\begin{matrix}x_1+1+x_2+1=-3m\\x_1x_2+x_1+x_2+1=-1\end{matrix}\right.\)
\(\Leftrightarrow\left\{{}\begin{matrix}x_1+1+x_2+1=-3m\\\left(x_1+1\right)\left(x_2+1\right)=-1\end{matrix}\right.\)
Đặt \(\left\{{}\begin{matrix}x_1+1=a\\x_2+1=b\end{matrix}\right.\) \(\Rightarrow\left\{{}\begin{matrix}a+b=-3m\\ab=-1\end{matrix}\right.\)
\(Q=a^4+b^4\ge2a^2b^2=2\)
Dấu "=" xảy ra khi \(a^2=b^2\Rightarrow\left[{}\begin{matrix}a=b\left(loại\right)\\a=-b\end{matrix}\right.\)
\(\Rightarrow-3m=0\Rightarrow m=0\)
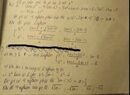

b) Theo hệ thức Vi ét ta có:
\(\left\{{}\begin{matrix}x_1+x_2=-\dfrac{2m-2}{m}\\x_1.x_2=\dfrac{m-1}{m}\end{matrix}\right.\)
\(\Leftrightarrow\left\{{}\begin{matrix}x_1+x_2=\dfrac{2-2m}{m}\\x_1.x_2=\dfrac{m-1}{m}\end{matrix}\right.\)
Ta có:
\(Q=\dfrac{1013}{x_1}+\dfrac{1013}{x_2}+1=1013\left(\dfrac{1}{x_1}+\dfrac{1}{x_2}\right)+1\)
\(=1013\left(\dfrac{x_1+x_2}{x_1.x_2}\right)+1=1013\left(\dfrac{\dfrac{2-2m}{m}}{\dfrac{m-1}{m}}\right)+1\)
\(=1013.\dfrac{-2\left(m-1\right)}{m-1}+1=-2026+1=-2025\), luôn là hằng số (đpcm)