TQ
1
K
Khách
Hãy nhập câu hỏi của bạn vào đây, nếu là tài khoản VIP, bạn sẽ được ưu tiên trả lời.
Các câu hỏi dưới đây có thể giống với câu hỏi trên
12 tháng 5 2022
*vn:vô nghiệm.
a. \(\left(x^2-2\right)\left(x^2+x+1\right)=0\)
\(\Leftrightarrow\left[{}\begin{matrix}x^2-2=0\\x^2+x+1=0\end{matrix}\right.\)
\(\Leftrightarrow\left[{}\begin{matrix}\left(x-\sqrt{2}\right)\left(x+\sqrt{2}\right)=0\\\left(x+\dfrac{1}{2}\right)^2+\dfrac{3}{4}=0\left(vn\right)\end{matrix}\right.\)
\(\Leftrightarrow x=\pm\sqrt{2}\)
-Vậy \(S=\left\{\pm\sqrt{2}\right\}\).
b. \(16x^2-8x+5=0\)
\(\Leftrightarrow16x^2-8x+1+4=0\)
\(\Leftrightarrow\left(4x-1\right)^2+4=0\) (vô lí)
-Vậy S=∅.
c. \(2x^3-x^2-8x+4=0\)
\(\Leftrightarrow x^2\left(2x-1\right)-4\left(2x-1\right)=0\)
\(\Leftrightarrow\left(2x-1\right)\left(x^2-4\right)=0\)
\(\Leftrightarrow\left(2x-1\right)\left(x-2\right)\left(x+2\right)=0\)
\(\Leftrightarrow\left[{}\begin{matrix}x=\dfrac{1}{2}\\x=\pm2\end{matrix}\right.\)
-Vậy \(S=\left\{\dfrac{1}{2};\pm2\right\}\).
d. \(3x^3+6x^2-75x-150=0\)
\(\Leftrightarrow3x^2\left(x+2\right)-75\left(x+2\right)=0\)
\(\Leftrightarrow3\left(x+2\right)\left(x^2-25\right)=0\)
\(\Leftrightarrow3\left(x+2\right)\left(x+5\right)\left(x-5\right)=0\)
\(\Leftrightarrow\left[{}\begin{matrix}x=-2\\x=\pm5\end{matrix}\right.\)
-Vậy \(S=\left\{-2;\pm5\right\}\)
2 tháng 2
a: 3(x+7)-2x+5>0
=>3x+21-2x+5>0
=>x+26>0
=>x>-26
Sửa đề: \(\dfrac{x+2}{18}-\dfrac{x+3}{8}< \dfrac{x-1}{9}-\dfrac{x-4}{24}\)
=>\(\dfrac{4\left(x+2\right)}{72}-\dfrac{9\left(x+3\right)}{72}< \dfrac{8\left(x-1\right)}{72}< \dfrac{3\left(x-4\right)}{72}\)
=>\(4\left(x+2\right)-9\left(x+3\right)< 8\left(x-1\right)-3\left(x-4\right)\)
=>\(4x+8-9x-27< 8x-8-3x+12\)
=>-5x-19<5x+4
=>-10x<23
=>\(x>-\dfrac{23}{10}\)
b: \(3x+2+\left|x+5\right|=0\left(1\right)\)
TH1: x>=-5
(1) trở thành: 3x+2+x+5=0
=>4x+7=0
=>\(x=-\dfrac{7}{4}\left(nhận\right)\)
TH2: x<-5
=>x+5<0
=>|x+5|=-x-5
Phương trình (1) sẽ trở thành:
\(3x+2-x-5=0\)
=>2x-3=0
=>2x=3
=>\(x=\dfrac{3}{2}\)
13 tháng 6 2023
b: =>1/4x+4/5-x-5=1/3x+1-1/2x+1
=>-3/4x+1/6x=2+5-4/5=24/5
=>x=-288/35
c: =>6x^2+3x-30x-15=6x^2+10x-21x-35
=>-27x-15=-11x-35
=>-16x=-20
=>x=5/4
19 tháng 4 2023
2:
a: =>x-1=0 hoặc 3x+1=0
=>x=1 hoặc x=-1/3
b: =>x-5=0 hoặc 7-x=0
=>x=5 hoặc x=7
c: =>\(\left[{}\begin{matrix}x-1=0\\x+5=0\\3x-8=0\end{matrix}\right.\Leftrightarrow x\in\left\{1;-5;\dfrac{8}{3}\right\}\)
d: =>x=0 hoặc x^2-1=0
=>\(x\in\left\{0;1;-1\right\}\)
8 tháng 3 2021
a) Ta có: \(x^2-2x+1=0\)
\(\Leftrightarrow\left(x-1\right)^2=0\)
\(\Leftrightarrow x-1=0\)hay x=1
Vậy: S={1}
c) Ta có: \(x+x^4=0\)
\(\Leftrightarrow x\left(x^3+1\right)=0\)
\(\Leftrightarrow x\left(x+1\right)\left(x^2-x+1\right)=0\)
mà \(x^2-x+1>0\forall x\)
nên x(x+1)=0
\(\Leftrightarrow\left[{}\begin{matrix}x=0\\x+1=0\end{matrix}\right.\Leftrightarrow\left[{}\begin{matrix}x=0\\x=-1\end{matrix}\right.\)
Vậy: S={0;-1}
2 tháng 3 2022
a. \(x^2-25-3.\left(x-5\right)=0\\ \Leftrightarrow\left(x-5\right)\left(x+5\right)-3.\left(x-5\right)=0\\ \Leftrightarrow\left(x-5\right)\left(x+5-3\right)=0\\ \Leftrightarrow\left[{}\begin{matrix}x-5=0\\x+2=0\end{matrix}\right.\Leftrightarrow\left[{}\begin{matrix}x=5\\x=-2\end{matrix}\right.\)
b. \(\left(3x+1\right)^2=\left(2x-5\right)\\ \Leftrightarrow9x^2+6x+1=2x-5\\ \Leftrightarrow9x^2+6x-2x=-5-1\\ \Leftrightarrow9x^2+4x=-6\\ \Leftrightarrow x\left(9x+4\right)=-6\\ \Leftrightarrow\left[{}\begin{matrix}x=-6\\9x+4=-6\end{matrix}\right.\Leftrightarrow\left[{}\begin{matrix}x=-6\\x=-\dfrac{10}{9}\end{matrix}\right.\)
c. \(2x^2-7x+6=0\\ \Leftrightarrow2x^2-7x=-6\\ \Leftrightarrow x\left(2x-7\right)=-6\\ \Leftrightarrow\left[{}\begin{matrix}x=-6\\x=\dfrac{1}{2}\end{matrix}\right.\)
2 tháng 3 2022
a, \(\left(x-5\right)\left(x+5\right)-3\left(x-5\right)=0\Leftrightarrow\left(x-5\right)\left(x+2\right)=0\Leftrightarrow x=-2;x=5\)
b, bạn ktra lại đề, thường thường ngta hay cho 2 vế cùng bình phương
c, \(2x^2-7x+6=0\Leftrightarrow\left(2x-3\right)\left(x-2\right)=0\Leftrightarrow x=\dfrac{3}{2};x=2\)
NN
9 tháng 6 2021
a) \(2\chi-3=3\left(\chi+1\right)\)
\(\Leftrightarrow2\chi-3=3\chi+3\)
\(\Leftrightarrow2\chi-3\chi=3+3\)
\(\Leftrightarrow\chi=-6\)
Vậy phương trình có tập nghiệm S= \(\left\{-6\right\}\)
\(3\chi-3=2\left(\chi+1\right)\)
\(\Leftrightarrow3\chi-3=2\chi+2\)
\(\Leftrightarrow3\chi-2\chi=2+3\)
\(\Leftrightarrow\chi=5\)
Vậy phương trình có tập nghiệm S= \(\left\{5\right\}\)
b) \(\left(3\chi+2\right)\left(4\chi-5\right)=0\)
\(\Leftrightarrow\left[{}\begin{matrix}3\chi+2=0\\4\chi-5=0\end{matrix}\right.\)
\(\Leftrightarrow\left[{}\begin{matrix}3\chi=-2\\4\chi=5\end{matrix}\right.\)
\(\Leftrightarrow\left[{}\begin{matrix}\chi=\dfrac{-2}{3}\\\chi=\dfrac{5}{4}\end{matrix}\right.\)
Vậy phương trình có tập nghiệm S= \(\left\{\dfrac{-2}{3};\dfrac{5}{4}\right\}\)
\(\left(3\chi+5\right)\left(4\chi-2\right)=0\)
\(\Leftrightarrow\left[{}\begin{matrix}3\chi+5=0\\4\chi-2=0\end{matrix}\right.\)
\(\Leftrightarrow\left[{}\begin{matrix}3\chi=-5\\4\chi=2\end{matrix}\right.\)
\(\Leftrightarrow\left[{}\begin{matrix}\chi=\dfrac{-5}{3}\\\chi=\dfrac{1}{2}\end{matrix}\right.\)
Vậy phương trình có tập nghiệm S= \(\left\{\dfrac{-5}{3};\dfrac{1}{2}\right\}\)
c) \(\left|\chi-7\right|=2\chi+3\)
Trường hợp 1:
Nếu \(\chi-7\ge0\Leftrightarrow\chi\ge7\)
Khi đó:\(\left|\chi-7\right|=2\chi+3\)
\(\Leftrightarrow\chi-7=2\chi+3\)
\(\Leftrightarrow\chi-2\chi=3+7\)
\(\Leftrightarrow\chi=-10\) (KTMĐK)
Trường hợp 2:
Nếu \(\chi-7\le0\Leftrightarrow\chi\le7\)
Khi đó: \(\left|\chi-7\right|=2\chi+3\)
\(\Leftrightarrow-\chi+7=2\chi+3\)
\(\Leftrightarrow-\chi-2\chi=3-7\)
\(\Leftrightarrow-3\chi=-4\)
\(\Leftrightarrow\chi=\dfrac{4}{3}\)(TMĐK)
Vậy phương trình có tập nghiệm S=\(\left\{\dfrac{4}{3}\right\}\)
\(\left|\chi-4\right|=5-3\chi\)
Trường hợp 1:
Nếu \(\chi-4\ge0\Leftrightarrow\chi\ge4\)
Khi đó: \(\left|\chi-4\right|=5-3\chi\)
\(\Leftrightarrow\chi-4=5-3\chi\)
\(\Leftrightarrow\chi+3\chi=5+4\)
\(\Leftrightarrow4\chi=9\)
\(\Leftrightarrow\chi=\dfrac{9}{4}\)(KTMĐK)
Trường hợp 2: Nếu \(\chi-4\le0\Leftrightarrow\chi\le4\)
Khi đó: \(\left|\chi-4\right|=5-3\chi\)
\(\Leftrightarrow-\chi+4=5-3\chi\)
\(\Leftrightarrow-\chi+3\chi=5-4\)
\(\Leftrightarrow2\chi=1\)
\(\Leftrightarrow\chi=\dfrac{1}{2}\)(TMĐK)
Vậy phương trình có tập nghiệm S=\(\left\{\dfrac{1}{2}\right\}\)
12 tháng 2 2022
a: \(\Leftrightarrow x^2-4-4x^2-4x-1-2x+3x^2=0\)
=>-6x-5=0
=>-6x=5
hay x=-5/6
b: \(\Leftrightarrow2x^3+8x^2+8x-8x^2-2x^3+16=0\)
=>8x+16=0
hay x=-2
c: \(\Leftrightarrow x^3-6x^2+12x-8+9x^2-1-x^3-3x^2-3x-1=0\)
=>9x-10=0
hay x=10/9
d: \(\Leftrightarrow10x-15-20x+28=19-2x^2-4x-2\)
\(\Leftrightarrow-10x+13+2x^2+4x-17=0\)
\(\Leftrightarrow2x^2-6x-4=0\)
\(\Leftrightarrow x^2-3x-2=0\)
\(\text{Δ}=\left(-3\right)^2-4\cdot1\cdot\left(-2\right)=9+8=17>0\)
Do đó: Phương trình có hai nghiệm phân biệt là:
\(\left\{{}\begin{matrix}x_1=\dfrac{3-\sqrt{17}}{2}\\x_2=\dfrac{3+\sqrt{17}}{2}\end{matrix}\right.\)
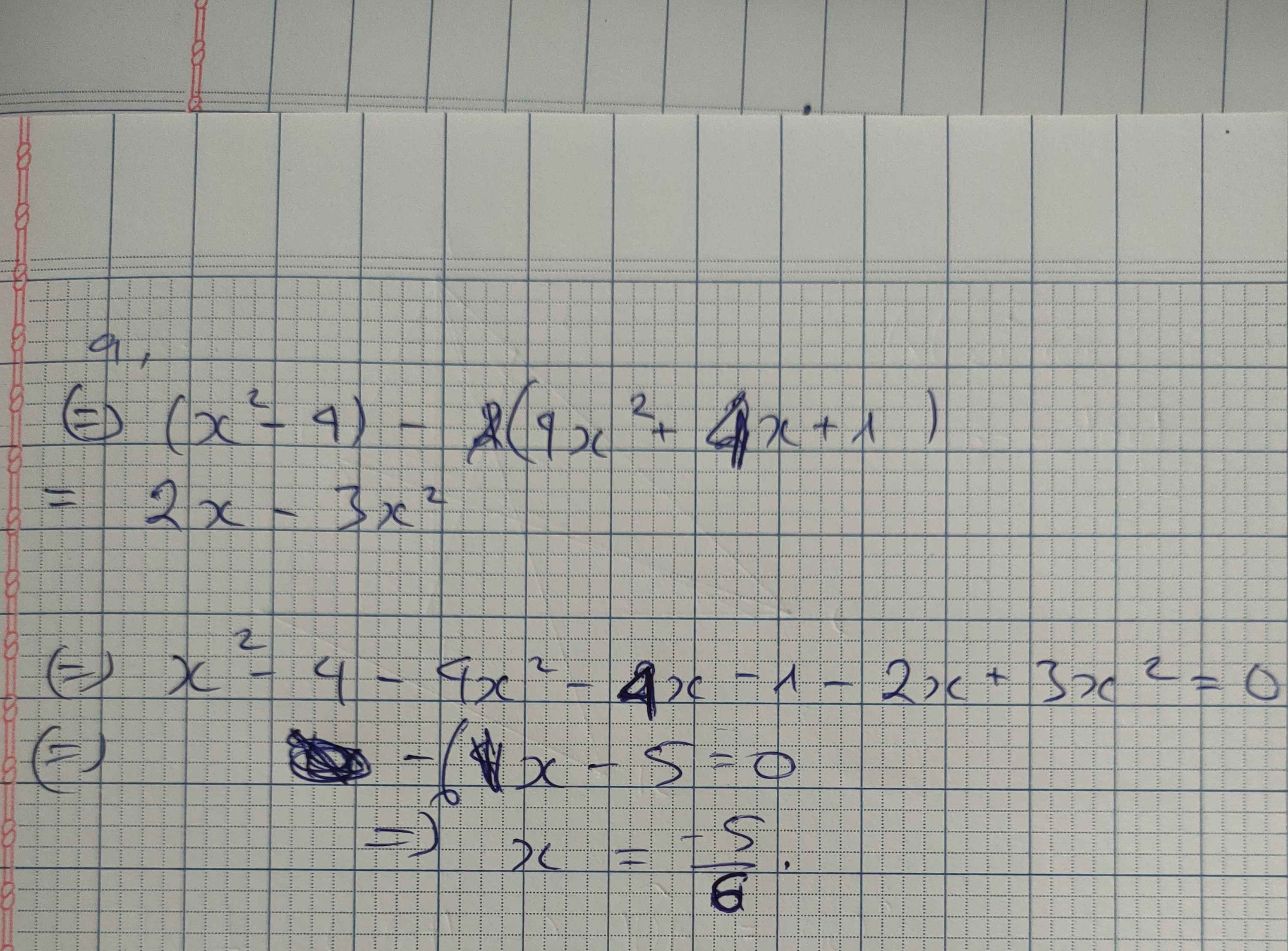
a) \(\left(x+1\right)^2\left(x+2\right)+\left(x+1\right)^2\left(x-2\right)=-24\)
\(\Leftrightarrow\left(x+1\right)^2\left(x+2+x-2\right)=0\)
\(\Leftrightarrow\left(x+1\right)^2\cdot2x=0\)
\(\Leftrightarrow\orbr{\begin{cases}\left(x+1\right)^2=0\\2x=0\end{cases}\Leftrightarrow\orbr{\begin{cases}x+1=0\\x=0\end{cases}\Leftrightarrow}\orbr{\begin{cases}x=-1\\x=0\end{cases}}}\)
b) \(2x^3+3x^2+6x+5=0\)
\(\Leftrightarrow2x^3+2x^2+x^2+x+5x+5=0\)
\(\Leftrightarrow2x^2\left(x+1\right)+x\left(x+1\right)+5\left(x+1\right)=0\)
\(\Leftrightarrow\left(x+1\right)\left(2x^2+x+5\right)=0\)
\(\Rightarrow x+1=0\left(2x^2+x+5\ne0\forall x\right)\)
<=> x=-1
Vậy x=-1