K
Khách
Hãy nhập câu hỏi của bạn vào đây, nếu là tài khoản VIP, bạn sẽ được ưu tiên trả lời.
Các câu hỏi dưới đây có thể giống với câu hỏi trên
PT
1
2 tháng 8 2021
Pt <=> 2sin\(\dfrac{3x}{2}\).cos\(\dfrac{x}{2}\) = 2cos\(\dfrac{3x}{2}\).cos\(\dfrac{x}{2}\)
⇔ cos\(\dfrac{x}{2}\) . \(\left(sin\dfrac{3x}{2}-cos\dfrac{3x}{2}\right)\) = 0
⇔ \(\sqrt{2}sin\left(\dfrac{3x}{2}-\dfrac{\pi}{4}\right).cos\dfrac{x}{2}=0\)
⇔
PT
1
CM
26 tháng 2 2018
Chọn D
Ta sẽ biến đổi phương trình thành dạng tích
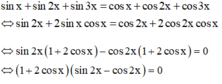
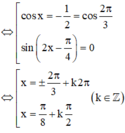
Chú ý: có thể dùng 4 đáp án thay vào phương trình để kiểm tra đâu là nghiệm
HP
24 tháng 12 2021
\(\sqrt{3}cos2x-sin2x=\sqrt{3}sinx+cosx\)
\(\Leftrightarrow\sqrt{3}cos2x-\sqrt{3}sinx-sin2x-cosx=0\)
\(\Leftrightarrow\sqrt{3}\left(1-2sin^2x-sinx\right)-2sinx.cosx-cosx=0\)
\(\Leftrightarrow-\sqrt{3}\left(sinx+1\right)\left(2sinx-1\right)-cosx\left(2sinx-1\right)=0\)
\(\Leftrightarrow\left(2sinx-1\right)\left[\sqrt{3}\left(sinx+1\right)+cosx\right]=0\)
\(\Leftrightarrow\left(2sinx-1\right)\left(\sqrt{3}sinx+cosx+\sqrt{3}\right)=0\)
\(\Leftrightarrow sinx=\dfrac{1}{2}\)
\(\Leftrightarrow\left[{}\begin{matrix}x=\dfrac{\pi}{6}+k2\pi\\x=\dfrac{5\pi}{6}+k2\pi\end{matrix}\right.\)
MA
0
TM
1
2
13 tháng 9 2023
`cos 2x+\sqrt{3}sin 2x+\sqrt{3}sin x-cos x=4`
`<=>1/2 cos 2x+\sqrt{3}/2 sin 2x+\sqrt{3}/2 sin x-1/2 cos x=2`
`<=>sin(\pi/6 +2x)+sin(x-\pi/6)=2`
Vì `-1 <= sin (\pi/6 +2x) <= 1`
`-1 <= sin (x-\pi/6) <= 1`
Dấu "`=`" xảy ra `<=>{(sin(\pi/6+2x)=1),(sin(x-\pi/6)=1):}`
`<=>{(\pi/6+2x=\pi/2+k2\pi),(x-\pi/6=\pi/2+k2\pi):}`
`<=>{(x=\pi/6+k\pi),(x=[2\pi]/3+k2\pi):}` `(k in ZZ)`
A
1
HP
15 tháng 8 2021
ĐK: \(x\ne\dfrac{\pi}{4}+k\pi;x\ne\dfrac{k\pi}{2}\)
\(\dfrac{2sin^2x+cos4x-cos2x}{\left(sinx-cosx\right)sin2x}=0\)
\(\Leftrightarrow2sin^2x+cos4x-cos2x=0\)
\(\Leftrightarrow2sin^2x-1+cos4x-cos2x+1=0\)
\(\Leftrightarrow2cos^22x-2cos2x=0\)
\(\Leftrightarrow\left[{}\begin{matrix}cos2x=0\\cos2x=1\end{matrix}\right.\)
\(\Leftrightarrow\left[{}\begin{matrix}2x=\dfrac{\pi}{2}+k\pi\\2x=k2\pi\end{matrix}\right.\)
\(\Leftrightarrow\left[{}\begin{matrix}x=\dfrac{\pi}{4}+\dfrac{k\pi}{2}\\x=k\pi\end{matrix}\right.\)
Đối chiếu điều kiện ta được \(x=-\dfrac{\pi}{4}+k\pi\)
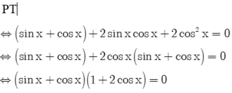
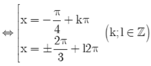
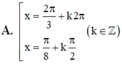
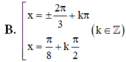
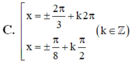
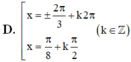
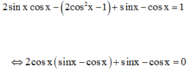
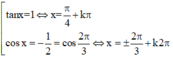
\(cos2x+cosx+1=sin2x+sinx\)
\(\Leftrightarrow cos^2x-sin^2x+cosx+cos^2x+sin^2x=2sinx.cosx+sinx\)
\(\Leftrightarrow2cos^2x+cosx=2sinx.cosx+sinx\)
\(\Leftrightarrow cosx\left(2cosx+1\right)=sinx\left(2cosx+1\right)\)
\(\Leftrightarrow\left(2cosx+1\right)\left(sinx-cosx\right)=0\)
\(\Leftrightarrow\left[{}\begin{matrix}2cosx+1=0\\sinx=cosx\end{matrix}\right.\)
\(\Rightarrow\left[{}\begin{matrix}cosx=-\dfrac{1}{2}\\tanx=1\end{matrix}\right.\)
\(\Rightarrow\left[{}\begin{matrix}x=\pm\dfrac{\pi}{3}+k2\pi\\x=\dfrac{\pi}{4}+k\pi\\\end{matrix}\right.\)