
Hãy nhập câu hỏi của bạn vào đây, nếu là tài khoản VIP, bạn sẽ được ưu tiên trả lời.


c) Ta có: \(\dfrac{5x^4+9x^3-2x^2-4x-8}{x-1}\)
\(=\dfrac{5x^4-5x^3+14x^3-14x^2+12x^2-12x+8x-8}{x-1}\)
\(=\dfrac{5x^3\left(x-1\right)+14x^2\left(x-1\right)+12x\left(x-1\right)+8\left(x-1\right)}{x-1}\)
\(=5x^3+14x^2+12x+8\)
d) Ta có: \(\dfrac{5x^3+14x^2+12x+8}{x+2}\)
\(=\dfrac{5x^3+10x^2+4x^2+8x+4x+8}{x+2}\)
\(=\dfrac{5x^2\left(x+2\right)+4x\left(x+2\right)+4\left(x+2\right)}{x+2}\)
\(=5x^2+4x+4\)

c) Ta có: \(\dfrac{5x^4+9x^3-2x^2-4x-8}{x-1}\)
\(=\dfrac{5x^4-5x^3+14x^3-14x^2+12x^2-12x+8x-8}{x-1}\)
\(=\dfrac{5x^3\left(x-1\right)+14x^2\left(x-1\right)+12x\left(x-1\right)+8\left(x-1\right)}{x-1}\)
\(=5x^3+14x^2+12x+8\)

b: \(\dfrac{\left(x^2-1\right)\left(x^2+1\right)-2x\left(x^2-1\right)}{x^2-1}\)
\(=x^2-2x+1\)
\(=\left(x-1\right)^2\)
c: \(=\dfrac{5x^4-5x^3+14x^3-14x^2+12x^2-12x+8x-8}{x-1}\)
\(=5x^3+14x^2+12x+8\)

1: Sửa đề: 3x-5
\(=\dfrac{-x^2\left(3x-5\right)-3\left(3x-5\right)}{3x-5}=-x^2-3\)
2: \(=\dfrac{5x^4-5x^3+14x^3-14x^2+12x^2-12x+8x-8}{x-1}\)
=5x^2+14x^2+12x+8
3: \(=\dfrac{5x^3+10x^2+4x^2+8x+4x+8}{x+2}=5x^2+4x+4\)
4: \(=\dfrac{\left(x^2-1\right)\left(x^2+1\right)-2x\left(x^2-1\right)}{x^2-1}=x^2+1-2x\)
5: \(=\dfrac{x^2\left(5-3x\right)+3\left(5-3x\right)}{5-3x}=x^2+3\)

Ta có: \(2x^4-21^3+34x^2+105x+50=0\)
\(\Leftrightarrow2x^4-12x^3-10x^2-9x^3+54x^2+45x-10x^2+60x+50=0\)
\(\Leftrightarrow2x^2\left(x^2-6x-5\right)-9x\left(x^2-6x-5\right)-10\left(x^2-6x-5\right)=0\)
\(\Leftrightarrow\left(x^2-6x-5\right)\left(2x^2-9x-10\right)=0\)
\(\Leftrightarrow\left[{}\begin{matrix}x^2-6x-5=0\\2x^2-9x-10=0\end{matrix}\right.\)\(\Leftrightarrow\left[{}\begin{matrix}x=3+\sqrt{14}\\x=3-\sqrt{14}\\x=\dfrac{9+\sqrt{161}}{4}\\x=\dfrac{9-\sqrt{161}}{4}\end{matrix}\right.\)

Lời giải của các bạn đều thỏa mãn yêu cầu đề bài là phân tích đa thức thành nhân tử

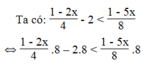
⇔ 2 – 4x – 16 < 1 – 5x
⇔ -4x + 5x < 1 – 2 + 16
⇔ x < 15
Vậy tập nghiệm của bất phương trình là: {x|x < 15}

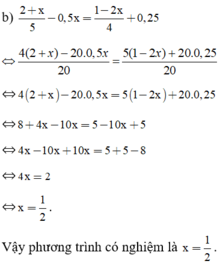
Lời giải:
$2x^4-9x^3+14x^2-9x+2=0$
$\Leftrightarrow 2x^4-2x^3-7x^3+7x^2+7x^2-7x-2x+2=0$
$\Leftrightarrow 2x^3(x-1)-7x^2(x-1)+7x(x-1)-2(x-1)=0$
$\Leftrightarrow (x-1)(2x^3-7x^2+7x-2)=0$
$\Leftrightarrow (x-1)[2(x^3-1)-7x(x-1)]=0$
$\Leftrightarrow (x-1)(x-1)(2x^2+2x+2-7x)=0$
$\Leftrightarrow (x-1)^2(2x^2-5x+2)=0$
$\Leftrightarrow (x-1)^2(2x^2-4x-x+2)=0$
$\Leftrightarrow (x-1)^2[2x(x-2)-(x-2)]=0$
$\Leftrightarrow (x-1)^2(2x-1)(x-2)=0$
\(\Rightarrow \left[\begin{matrix} x=1\\ x=\frac{1}{2}\\ x=2\end{matrix}\right.\)