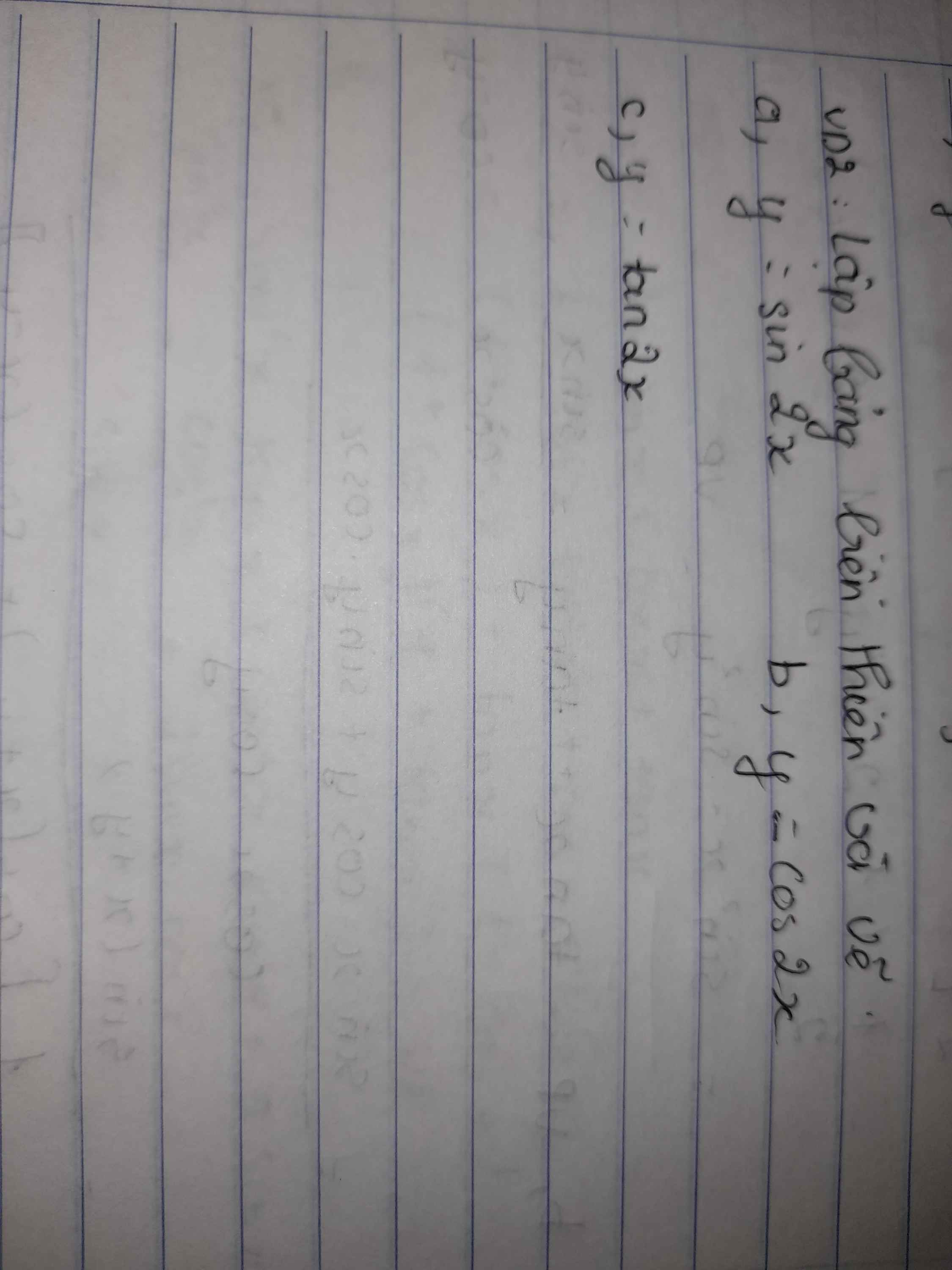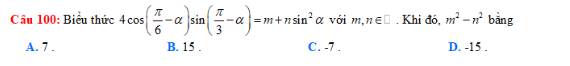K
Khách
Hãy nhập câu hỏi của bạn vào đây, nếu là tài khoản VIP, bạn sẽ được ưu tiên trả lời.
Các câu hỏi dưới đây có thể giống với câu hỏi trên
NV
Nguyễn Việt Lâm
Giáo viên
30 tháng 7 2021
a.
\(sin\left(2x-\dfrac{\pi}{4}\right)=-1\)
\(\Leftrightarrow2x-\dfrac{\pi}{4}=-\dfrac{\pi}{2}+k2\pi\)
\(\Leftrightarrow x=-\dfrac{\pi}{8}+k\pi\) (1)
\(-\dfrac{\pi}{3}\le x\le\dfrac{7\pi}{3}\Rightarrow-\dfrac{\pi}{3}\le-\dfrac{\pi}{8}+k\pi\le\dfrac{7\pi}{3}\)
\(\Rightarrow-\dfrac{5}{24}\le k\le\dfrac{59}{24}\Rightarrow k=\left\{0;1;2\right\}\)
Thế vào (1) \(\Rightarrow x=\left\{-\dfrac{\pi}{8};\dfrac{7\pi}{8};\dfrac{15\pi}{8}\right\}\)
HP
7 tháng 9 2021
5.
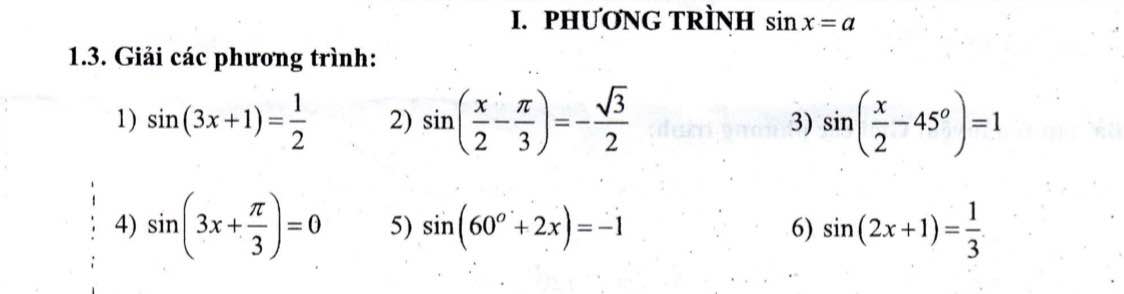
\(sin\left(60^o+2x\right)=-1\)
\(\Leftrightarrow60^o+2x=-90^o+k.360^o\)
\(\Leftrightarrow x=-75^o+k.180^o\)
6.
\(sin\left(2x+1\right)=\dfrac{1}{3}\)
\(\Leftrightarrow\left[{}\begin{matrix}2x+1=arcsin\dfrac{1}{3}+k2\pi\\2x+1=\pi-arcsin\dfrac{1}{3}+k2\pi\end{matrix}\right.\)
\(\Leftrightarrow\left[{}\begin{matrix}x=\dfrac{1}{2}arcsin\dfrac{1}{3}-\dfrac{1}{2}+k\pi\\x=\dfrac{\pi}{2}-\dfrac{1}{2}arcsin\dfrac{1}{3}-\dfrac{1}{2}+k\pi\end{matrix}\right.\)
7 tháng 9 2021
Cách làm :
sina = \(\dfrac{1}{2}\) ⇔ \(\left[{}\begin{matrix}a=\dfrac{\pi}{6}+k2\pi\\a=\dfrac{5\pi}{6}+k2\pi\end{matrix}\right.\)
sina = \(-\dfrac{\sqrt{3}}{2}\) ⇔ \(\left[{}\begin{matrix}a=-\dfrac{\pi}{3}+k2\pi\\a=\dfrac{4\pi}{3}+k2\pi\end{matrix}\right.\)
sina = 1 ⇔ \(a=\dfrac{\pi}{2}+k.2\pi\)
sina = 0 ⇔ \(a=k\pi\)
sina = -1 ⇔ \(a=-\dfrac{\pi}{2}+k.2\pi\)
sina = \(\dfrac{1}{3}\) ⇔ \(\left[{}\begin{matrix}a=arcsin\left(\dfrac{1}{3}\right)+k2\pi\\a=\pi-arcsin\left(\dfrac{1}{3}\right)+k2\pi\end{matrix}\right.\)
Với a là một đa thức xác định trên R
L
1
27 tháng 10 2023
23:
u4=10 và u7=22
=>\(\left\{{}\begin{matrix}u1+3d=10\\u1+6d=22\end{matrix}\right.\Leftrightarrow\left\{{}\begin{matrix}-3d=-12\\u1+3d=10\end{matrix}\right.\)
=>\(\left\{{}\begin{matrix}d=4\\u1=10-12=-2\end{matrix}\right.\)
=>Chọn C
Câu 22:
\(\left\{{}\begin{matrix}u1+2u5=0\\S_4=14\end{matrix}\right.\)
=>\(\left\{{}\begin{matrix}u1+2\left(u1+4d\right)=0\\4\cdot\dfrac{\left[2u1+3d\right]}{2}=14\end{matrix}\right.\)
=>\(\left\{{}\begin{matrix}3u1+8d=0\\2u1+3d=7\end{matrix}\right.\Leftrightarrow\left\{{}\begin{matrix}6u1+16d=0\\6u1+9d=21\end{matrix}\right.\)
=>\(\left\{{}\begin{matrix}7d=-21\\2u_1+3d=7\end{matrix}\right.\Leftrightarrow\left\{{}\begin{matrix}d=-3\\2u_1=7-3d=7+9=16\end{matrix}\right.\)
=>\(u_1=8;d=-3\)
=>Chọn A
21A
19B
BT
0
NV
Nguyễn Việt Lâm
Giáo viên
1 tháng 6 2021
Theo nguyên lý Dirichlet, nếu số chữ số 3 lớn hơn 5 thì luôn có ít nhất 2 chữ số 3 đứng cạnh nhau (ko thỏa mãn).
- Nếu ko có chữ số 3 nào: có đúng 1 số
- Nếu có 1 chữ số 3: xếp 9 chữ số 2 tạo ra 10 khe trống, có \(C_{10}^1\) cách đặt số 3 vào các khe trống đó \(\Rightarrow\) 10 số
- Nếu có 2 chữ số 3 (và 8 chữ số 2): xếp 8 chữ số 2 tạo thành 9 khe trống, xếp 2 chữ số 3 vào 9 khe trống đó: \(C_9^2=36\) số
- Nếu có 3 chữ số 3 và 7 chữ số 2: xếp 7 chữ số 2 tạo thành 8 khe trống, xếp 3 chữ số 3 vào 8 khe trống: \(C_8^3=...\)
Làm tương tự, nói chung kết quả sẽ là: \(C_{11}^0+C_{10}^1+C_9^2+C_8^3+C_7^4+C_6^5=...\)
DT
1
NV
Nguyễn Việt Lâm
Giáo viên
18 tháng 10 2020
a/
Nhận thấy \(cosx=0\) không phải nghiệm, chia 2 vế cho \(cos^2x\)
\(\Leftrightarrow3tan^2x+8tanx+8\sqrt{3}-9=0\)
\(\Leftrightarrow\left[{}\begin{matrix}tanx=-\sqrt{3}\\tanx=\frac{3\sqrt{3}-8}{3}\end{matrix}\right.\)
\(\Leftrightarrow\left[{}\begin{matrix}x=-\frac{\pi}{3}+k\pi\\x=arctan\left(\frac{3\sqrt{3}-8}{3}\right)+k\pi\end{matrix}\right.\)
b/
Nhận thấy \(cosx=0\) ko phải nghiệm, chia 2 vế cho \(cos^2x\)
\(tan^2x+2tanx-2=\frac{1}{2}\left(1+tan^2x\right)\)
\(\Leftrightarrow tan^2x+4tanx-5=0\Rightarrow\left[{}\begin{matrix}tanx=1\\tanx=-5\end{matrix}\right.\)
\(\Leftrightarrow\left[{}\begin{matrix}x=\frac{\pi}{4}+k\pi\\x=arctan\left(-5\right)+k\pi\end{matrix}\right.\)
c/
\(\Leftrightarrow\left(sinx+1\right)\left(1-2sin^2x-1\right)=0\)
\(\Leftrightarrow sin^2x\left(sinx+1\right)=0\Leftrightarrow\left[{}\begin{matrix}sinx=0\\sinx=-1\end{matrix}\right.\)
\(\Leftrightarrow\left[{}\begin{matrix}x=k\pi\\x=-\frac{\pi}{2}+k2\pi\end{matrix}\right.\)
BA
1
21 tháng 7 2023
4*cos(pi/6-a)*sin(pi/3-a)
=4*(cospi/6*cosa+sinpi/6*sina)*(sinpi/3*cosa-sina*cospi/3)
=4*(căn 3/2*cosa+1/2*sina)*(căn 3/2*cosa-1/2*sina)
=4*(3/4*cos^2a-1/4*sin^2a)
=3cos^2a-sin^2a
=3(1-sin^2a)-sin^2a
=3-4sin^2a
=>m=3; n=-4
m^2-n^2=-7