Giải chi tiết dùm mình với nhaCamon nhìu ạ

Khách

Hãy nhập câu hỏi của bạn vào đây, nếu là tài khoản VIP, bạn sẽ được ưu tiên trả lời.

Chọn lớp

Chọn môn

Mua vip

Quỳnh Anh

7 tháng 10 2021 - olm

Giải chi tiết dùm mình với nha

Camon nhìu ạ <3

#Toán lớp 10

Phạm Lê Chí Bảo

7 tháng 10 2021

Trung bình cộng của 8 số là 34.Tổng các số đó là

Đúng(0)

Phạm Lê Chí Bảo

7 tháng 10 2021

1+1+1+1+1+1+1+1+2+2+21++1+1+1+1

Đúng(0)

Các câu hỏi dưới đây có thể giống với câu hỏi trên

Quỳnh Anh

7 tháng 10 2021

Giải chi tiết dùm em nhaaa... Camon nhìu nhaa

#Toán lớp 10

Dương Thị Thu Hiền

29 tháng 12 2021

MN GIÚP E CÂU 5 VỚI Ạ. GIẢI CHI TIẾT CÁCH LÀM DÙM E VỚI Ạ.

#Toán lớp 10

Nguyễn Lê Phước Thịnh

29 tháng 12 2021

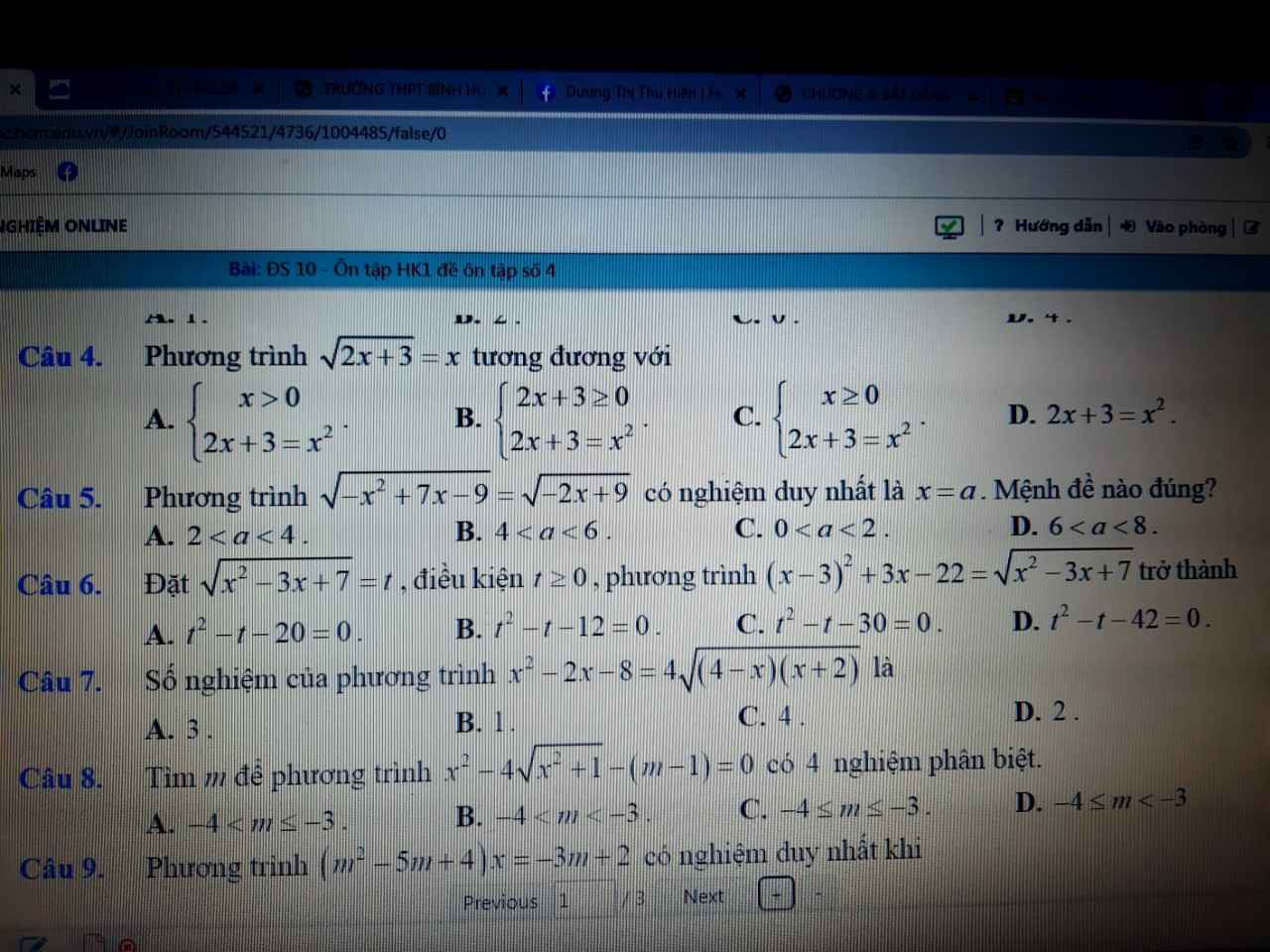
Câu 5:

\(\Leftrightarrow-x^2+7x-9+2x-9=0\)

\(\Leftrightarrow x^2-9x+18=0\)

=>x=3

=>Chọn A

Đúng(5)

Dương Thị Thu Hiền

29 tháng 12 2021

MN GIÚP E BÀI 2,7,8 VỚI Ạ. GIẢI CHI TIẾT CÁCH LÀM DÙM E.

#Toán lớp 10

Nguyễn Lê Phước Thịnh

29 tháng 12 2021

Câu 2: C

Đúng(3)

Dương Thị Thu Hiền

29 tháng 12 2021

CÁCH LÀM SAO Ạ.

Đúng(1)

Ngọc Nhã Uyên Hạ

24 tháng 8 2021

Mình chưa hiểu bài này lắm. Có thể giải chi tiết dùm được hông ạ. Đang cần gấp Cảm ơn trc nha

#Toán lớp 10

Nguyễn Thị Quỳnh Anh

16 tháng 12 2021

Giúp em bài 8 vs ạ em đang cần gấp pleaseeeeeeeee Giải chi tiết ra giùm em ạ em cảm ơn nhìu

#Toán lớp 10

Nguyễn Lê Phước Thịnh

17 tháng 12 2021

Câu 58: B

Câu 59: C

Đúng(0)

nguyễn huỳnh minh khôi

27 tháng 12 2021

giải chi tiết giúp mình với...

Đọc tiếp

#Toán lớp 10

Ami Mizuno

27 tháng 12 2021

Ta có: \(x^2-6x+m-2=0\)

\(\Rightarrow\Delta=6^2-4\left(m-2\right)\)

Để phương tình có hai nghiệm phân biệt thì \(\Delta>0\)

\(\Rightarrow36-4m+8>0\Leftrightarrow44>4m\Leftrightarrow11>m\)

Câu D

Đúng(0)

Nguyễn Nhung

23 tháng 7 2021

Giúp mình giải chi tiết với ạ

#Toán lớp 10

Ami Mizuno

23 tháng 7 2021

Câu 1: Cho t=1 ta có \(\left\{{}\begin{matrix}x=1+1\\y=2-2.1\\z=3+1\end{matrix}\right.\)\(\Leftrightarrow\left\{{}\begin{matrix}x=2\\y=0\\z=4\end{matrix}\right.\)

Vậy điểm M là M(2,0,4). Khoanh B.

Câu 2: Ta có tọa độ điểm O thuộc (d) là: O(1,2,3)

Để (d') cũng là phương trình tham số của (d) thì (d') phải đi qua điểm O(1,2,3)

Quan sát đáp án ta thấy đáp án B đúng.

Đúng(1)

Thành Danh Đỗ

23 tháng 3 2022

Giải chi tiết giúp mình với ạ

#Toán lớp 10

Nguyễn Việt Lâm

Giáo viên

23 tháng 3 2022

26.

\(\left|2x-3\right|\le x+3\Leftrightarrow\left\{{}\begin{matrix}x+3\ge0\\\left(2x-3\right)^2\le\left(x+3\right)^2\end{matrix}\right.\)

\(\Leftrightarrow\left\{{}\begin{matrix}x\ge-3\\x^2-6x\le0\end{matrix}\right.\)

\(\Leftrightarrow\left\{{}\begin{matrix}x\ge-3\\0\le x\le6\end{matrix}\right.\)

\(\Rightarrow0\le x\le6\)

\(\sqrt{x^2+x-6}\ge x+2\Leftrightarrow\left[{}\begin{matrix}\left\{{}\begin{matrix}x+2< 0\\x^2+x-6\ge0\end{matrix}\right.\\\left\{{}\begin{matrix}x+2\ge0\\x^2+x-6\ge\left(x+2\right)^2\end{matrix}\right.\end{matrix}\right.\)

\(\Leftrightarrow\left[{}\begin{matrix}\left\{{}\begin{matrix}x< -2\\\left[{}\begin{matrix}x\ge2\\x\le-3\end{matrix}\right.\end{matrix}\right.\\\left\{{}\begin{matrix}x\ge-2\\3x\le-10\end{matrix}\right.\end{matrix}\right.\) \(\Rightarrow x\le-3\)

Đúng(1)