Hãy nhập câu hỏi của bạn vào đây, nếu là tài khoản VIP, bạn sẽ được ưu tiên trả lời.

a là nghiệm nên \(\sqrt{2}a^2+a-1=0\Rightarrow\sqrt{2}a^2=1-a\)
\(\Rightarrow2a^4=\left(1-a\right)^2=a^2-2a+1\)
\(\Rightarrow2a^4-2a+3=a^2-4a+4=\left(a-2\right)^2\)
Mặt khác \(1-a=\sqrt{2}a^2>0\Rightarrow a< 1\)
\(\Rightarrow\sqrt{2\left(2a^4-2a+3\right)}+2a^2=\sqrt{2\left(a-2\right)^2}+2a^2=\sqrt{2}\left(2-a\right)+2a^2\)
\(=\sqrt{2}\left(\sqrt{2}a^2-a+2\right)=\sqrt{2}\left(1-a-a+2\right)=\sqrt{2}\left(3-2a\right)\)
\(\Rightarrow C=\dfrac{2a-3}{\sqrt{2}\left(3-2a\right)}=-\dfrac{\sqrt{2}}{2}\)

Bài 1.
Ta có:\(\left(x+\sqrt{x^2+2020}\right)\left(\sqrt{x^2+2020}-x\right)=x^2+2020-x^2=2020\)
\(\Rightarrow\left(x+\sqrt{x^2+2020}\right)\left(y+\sqrt{y^2+2020}\right)=\left(x+\sqrt{x^2+2020}\right)\left(\sqrt{x^2+2020}-x\right)\)
\(\Rightarrow y+\sqrt{y^2+2020}=\sqrt{x^2+2020}-x\)
\(\Rightarrow x+y=\sqrt{x^2+2020}-\sqrt{y^2+2020}\) (1)
Ta có:\(\left(y+\sqrt{y^2+2020}\right)\left(\sqrt{y^2+2020}-y\right)=y^2+2020-y^2=2020\)
\(\Rightarrow\left(x+\sqrt{x^2+2020}\right)\left(y+\sqrt{y^2+2020}\right)=\left(y+\sqrt{y^2+2020}\right)\left(\sqrt{y^2+2020}-y\right)\)
\(\Rightarrow x+\sqrt{x^2+2020}=\sqrt{y^2+2020}-y\)
\(\Rightarrow x+y=\sqrt{y^2+2020}-\sqrt{x^2+2020}\) (2)
Cộng vế với vế của (1) và (2) ta có:
\(2\left(x+y\right)=\sqrt{y^2+2020}-\sqrt{x^2+2020}+\sqrt{x^2+2020}-\sqrt{y^2+2020}\)
\(\Rightarrow2\left(x+y\right)=0\Rightarrow x+y=0\)
Bài 2:
Ta có: (2a+1)(2b+1)=9
nên \(2b+1=\dfrac{9}{2a+1}\)
\(\Leftrightarrow2b=\dfrac{9}{2a+1}-\dfrac{2a+1}{2a+1}=\dfrac{8-2a}{2a+1}\)
\(\Leftrightarrow b=\dfrac{8-2a}{4a+2}=\dfrac{4-a}{2a+1}\)
\(\Leftrightarrow b+2=\dfrac{4-a+4a+2}{2a+1}=\dfrac{3a+6}{2a+1}\)
Ta có: \(A=\dfrac{1}{a+2}+\dfrac{1}{b+2}\)
\(=\dfrac{1}{a+2}+\dfrac{2a+1}{3a+6}\)
\(=\dfrac{3+2a+1}{3a+6}\)
\(=\dfrac{2a+4}{3a+6}=\dfrac{2}{3}\)

2a^4=(1-a)^2=a^2-2a+1
\(A=\frac{2a-3}{\sqrt{2\left(a^2-4a+4\right)}+2a^2}=\frac{2a-3}{\sqrt{2}!\left(a-2\right)!+2a^2}\)a> 2 không thể là nghiệm=> a<2
\(A=\frac{2a-3}{\sqrt{2}\left(2-a\right)+2a^2}=\frac{2a-3}{2a^2-\sqrt{2}a+2\sqrt{2}}=\frac{2a-3}{\sqrt{2}\left(\sqrt{2}a^2-a-1+3\right)}\)
\(A=\frac{2a-3}{\sqrt{2}\left(3\right)}\)

nghiệm a si đa quá ._.
\(\sqrt{2}x^2+x-1=0\)
\(\Delta=1^2-\left(4\sqrt{2}-1\right)=\sqrt{32}+1\)
\(\Rightarrow x_{1,2}=\frac{-1\pm\sqrt{\sqrt{32}+1}}{2\sqrt{2}}\).....

Thay \(\sqrt{2}a^2=1-a\ge\)0 suy ra a <=1 tính được mẫu = \(-\sqrt{2}\left(2a-3\right)\)

Bài 1:
\(\frac{(x+1)^4}{(x^2+1)^2}+\frac{4x}{x^2+1}=6\)
\(\Leftrightarrow \frac{(x+1)^4+4x(x^2+1)}{(x^2+1)^2}=6\)
\(\Leftrightarrow \frac{x^4+8x^3+6x^2+8x+1}{(x^2+1)^2}=6\Rightarrow x^4+8x^3+6x^2+8x+1=6(x^2+1)^2\)
\(\Leftrightarrow x^4+8x^3+6x^2+8x+1=6(x^4+2x^2+1)\)
\(\Leftrightarrow 5x^4-8x^3+6x^2-8x+5=0\)
\(\Leftrightarrow 5x^3(x-1)-3x^2(x-1)+3x(x-1)-5(x-1)=0\)
\(\Leftrightarrow (x-1)(5x^3-3x^2+3x-5)=0\)
\(\Leftrightarrow (x-1)[5(x-1)(x^2+x+1)-3x(x-1)]=0\)
\(\Leftrightarrow (x-1)^2(5x^2+2x+5)=0\)
Dễ thấy \(5x^2+2x+5>0\), do đó \((x-1)^2=0\Leftrightarrow x=1\)
Bài 2: ĐK: \(x\geq 0\)
\(A=\frac{x^2-\sqrt{x}}{x+\sqrt{x}+1}-\frac{x^2+\sqrt{x}}{x-\sqrt{x}+1}+x+1\)
\(A=\frac{\sqrt{x}(\sqrt{x^3}-1)}{x+\sqrt{x}+1}-\frac{\sqrt{x}(\sqrt{x^3}+1)}{x-\sqrt{x}+1}+x+1\)
\(A=\frac{\sqrt{x}(\sqrt{x}-1)(x+\sqrt{x}+1)}{x+\sqrt{x}+1}-\frac{\sqrt{x}(\sqrt{x}+1)(x-\sqrt{x}+1)}{x-\sqrt{x}+1}+x+1\)
\(A=\sqrt{x}(\sqrt{x}-1)-\sqrt{x}(\sqrt{x}+1)+x+1\)
\(A=x-2\sqrt{x}+1=(\sqrt{x}-1)^2\)

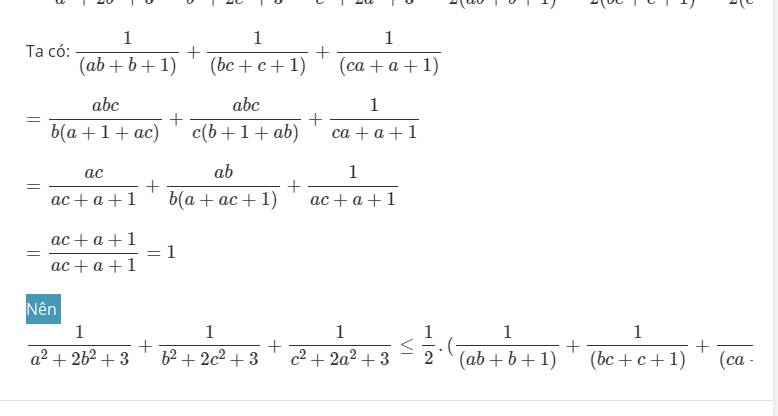


a) a là 1 nghiệm \(\Rightarrow\sqrt{2}a^2+a-1=0\Leftrightarrow2a^4=\left(1-a\right)^2=a^2-2a+1\)
\(\Rightarrow2a^4-2a+3=a^2-2a+1-2a+3=\left(a-2\right)^2\)
\(\sqrt{2\left(2a^4-2a+3\right)}+2a^2=\sqrt{2}\left(a-2\right)+2a^2\)(1)
mà \(\sqrt{2}a^2+a-1=0\Rightarrow2a^2+\sqrt{2}a-\sqrt{2}=0\)
(1)= \(2a^2+\sqrt{2}a-2\sqrt{2}=-\sqrt{2}\)
...
b) find nghiệm nguyên dương:
\(Pt\Leftrightarrow x^2+2y^2+2xy-2\left(x+2y\right)+1=0\)
\(\Leftrightarrow x^2+2x\left(y-1\right)+\left(2y^2-4y+1\right)=0\)\(\Delta'=\left(y-1\right)^2-\left(2y^2-4y+1\right)=-y^2+2y\ge0\)
\(\Leftrightarrow0\le y\le2\) kết hợp \(y\in N\)=> ....