Hãy nhập câu hỏi của bạn vào đây, nếu là tài khoản VIP, bạn sẽ được ưu tiên trả lời.

1.
\(SA\perp\left(ABC\right)\Rightarrow AC\) là hình chiếu vuông góc của SA lên (ABC)
\(\Rightarrow\widehat{SCA}\) là góc giữa SC là (ABC)
\(AC=\sqrt{AB^2+BC^2}=a\sqrt{5}\)
\(\Rightarrow tan\widehat{SCA}=\dfrac{SA}{AC}=\dfrac{\sqrt{15}}{5}\Rightarrow\widehat{SCA}\approx37^045'\)
b.
Ta có: \(\left\{{}\begin{matrix}SA\perp\left(ABC\right)\Rightarrow SA\perp BC\\AB\perp BC\end{matrix}\right.\) \(\Rightarrow BC\perp\left(SAB\right)\)
Mà \(\left\{{}\begin{matrix}BC=\left(SBC\right)\cap\left(ABC\right)\\SB=\left(SBC\right)\cap\left(SAB\right)\\AB=\left(ABC\right)\cap\left(SAB\right)\end{matrix}\right.\) \(\Rightarrow\widehat{SBA}\) là góc giữa (SBC) và (ABC)
\(tan\widehat{SBA}=\dfrac{SA}{AB}=\sqrt{3}\Rightarrow\widehat{SBA}=60^0\)
c.
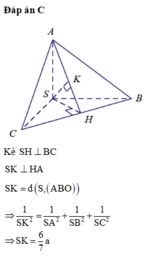
Trong mp (SAB), từ A kẻ \(AH\perp SB\)
Mà \(BC\perp\left(SAB\right)\Rightarrow BC\perp AH\)
\(\Rightarrow AH\perp\left(SBC\right)\Rightarrow AH=d\left(A;\left(SBC\right)\right)\)
Áp dụng hệ thức lượng:
\(\dfrac{1}{AH^2}=\dfrac{1}{SA^2}+\dfrac{1}{AB^2}\Rightarrow AH=\dfrac{SA.AB}{\sqrt{SA^2+AB^2}}=\dfrac{a\sqrt{3}}{2}\)
2.
\(SA\perp\left(ABCD\right)\Rightarrow AB\) là hình chiếu vuông góc của SB lên (ABCD)
\(\Rightarrow\widehat{SBA}\) là góc giữa SB là (ABCD)
\(tan\widehat{SBA}=\dfrac{SA}{AB}=3\Rightarrow\widehat{SBA}\approx71^034'\)
b.
\(\left\{{}\begin{matrix}SA\perp\left(ABCD\right)\Rightarrow SA\perp BC\\AB\perp BC\end{matrix}\right.\) \(\Rightarrow BC\perp\left(SAB\right)\)
Mà \(\left\{{}\begin{matrix}BC=\left(SBC\right)\cap\left(ABCD\right)\\SB=\left(SAB\right)\cap\left(SBC\right)\\AB=\left(SAB\right)\cap\left(ABCD\right)\end{matrix}\right.\)
\(\Rightarrow\widehat{SBA}\) là góc giữa (SBC) và (ABCD) (đã tính ở câu a)
c.
Từ A kẻ \(AH\perp SB\Rightarrow AH\perp\left(SBC\right)\)
\(\Rightarrow AH=d\left(A;\left(SBC\right)\right)\)
\(\dfrac{1}{AH^2}=\dfrac{1}{SA^2}+\dfrac{1}{AB^2}\Rightarrow AH=\dfrac{SA.AB}{\sqrt{SA^2+AB^2}}=\dfrac{3a\sqrt{10}}{10}\)

vẫn hình vẽ ấy lập luận tương tự
Gọi M là trung điểm của AB ta có:
AB _|_ SM ( tam giác SAB cân tại S ) (1)
AB _|_ CM ( tam giác ABC đều ) (2)
Từ (1),(2) suy ra AB vuông góc SCM
suy ra góc (AB,SC)=90