
Hãy nhập câu hỏi của bạn vào đây, nếu là tài khoản VIP, bạn sẽ được ưu tiên trả lời.


Ta có: \(P=\dfrac{\sqrt{x}+1}{x-1}-\dfrac{x+2}{x\sqrt{x}-1}-\dfrac{\sqrt{x}+1}{x+\sqrt{x}+1}\)
\(=\dfrac{x+\sqrt{x}+1-x-2-\left(x-1\right)}{\left(\sqrt{x}-1\right)\left(x+\sqrt{x}+1\right)}\)
\(=\dfrac{\sqrt{x}-1-x+1}{\left(\sqrt{x}-1\right)\left(x+\sqrt{x}+1\right)}\)
\(=\dfrac{-\sqrt{x}\left(\sqrt{x}-1\right)}{\left(\sqrt{x}-1\right)\left(x+\sqrt{x}+1\right)}\)
\(=\dfrac{-\sqrt{x}}{x+\sqrt{x}+1}\)

a) Ta có: \(A=\left(\dfrac{a\sqrt{a}-1}{a-\sqrt{a}}-\dfrac{a\sqrt{a}+1}{a+\sqrt{a}}\right):\dfrac{a+2}{a-2}\)
\(=\left(\dfrac{a+\sqrt{a}+1-\left(a-\sqrt{a}+1\right)}{\sqrt{a}}\right):\dfrac{a+2}{a-2}\)
\(=2\cdot\dfrac{a-2}{a+2}\)
\(=\dfrac{2a-4}{a+2}\)

`P=(1+5/(sqrtx-2)).(sqrtx-(x+2sqrtx+4)/(sqrtx+3))`
`=((sqrtx-2+5)/(sqrtx-2)).((x+3sqrtx-x-2sqrtx-4)/(sqrtx+3))`
`=(sqrtx+3)/(sqrtx-2).(sqrtx-4)/(sqrtx+3)`
`=(sqrtx-4)/(sqrtx-2)`

\(x-\sqrt{x}-2=\left(\sqrt{x}-2\right)\left(\sqrt{x}+1\right)\)

\(\sqrt{5-2\sqrt{6}}-\sqrt{5+2\sqrt{6}}\\ =\sqrt{\left(\sqrt{3}-\sqrt{2}\right)^2}-\sqrt{\left(\sqrt{3}+\sqrt{2}\right)^2}\\ =\left|\sqrt{3}-\sqrt{2}\right|-\left|\sqrt{3}+\sqrt{2}\right|\\ =\sqrt{3}-\sqrt{2}-\sqrt{3}-\sqrt{2}\\ =-2\sqrt{2}\)

gánh còng não :v
\(\left(\dfrac{\sqrt{y}}{x+\sqrt{y}}+\dfrac{\sqrt{y}}{x-\sqrt{xy}}\right):\dfrac{2\sqrt{xy}}{xy}=\left(\dfrac{\sqrt{y}}{x+\sqrt{y}}+\dfrac{\sqrt{y}}{\sqrt{x}\left(\sqrt{x}-\sqrt{y}\right)}\right):\dfrac{2}{\sqrt{xy}}=\dfrac{\sqrt{xy}\left(\sqrt{x}-\sqrt{y}\right)+\sqrt{x}\left(x+\sqrt{y}\right)}{\sqrt{x}\left(x+\sqrt{y}\right)\left(\sqrt{x}-\sqrt{y}\right)}:\dfrac{2}{\sqrt{xy}}=\dfrac{x\sqrt{y}-y\sqrt{x}+x\sqrt{x}+\sqrt{xy}}{\sqrt{x}\left(x+\sqrt{y}\right)\left(\sqrt{x}-\sqrt{y}\right)}:\dfrac{2}{\sqrt{xy}}=\dfrac{\sqrt{x}\left(\sqrt{xy}-y+x+\sqrt{y}\right)}{\sqrt{x}\left(x+\sqrt{y}\right)\left(\sqrt{x}-\sqrt{y}\right)}:\dfrac{2}{\sqrt{xy}}=\dfrac{\sqrt{y}\left(\sqrt{x}-\sqrt{y}\right)+\left(x+\sqrt{y}\right)}{\left(x +\sqrt{y}\right)\left(\sqrt{x}-\sqrt{y}\right)}:\dfrac{2}{\sqrt{xy}}\) mình làm đc đó thôi ( mỏi tay :v )

\(X=\dfrac{3}{\left(\sqrt{x}-2\right)\left(\sqrt{x}+1\right)}+\dfrac{2}{\sqrt{x}+1}+\dfrac{1}{2-\sqrt{x}}\left(đk:x\ge0;x\ne4\right)\)
\(X=\dfrac{3}{\left(\sqrt{x}-2\right)\left(\sqrt{x}+1\right)}+\dfrac{2}{\sqrt{x}+1}-\dfrac{1}{\sqrt{x}-2}\)
\(X=\dfrac{3}{\left(\sqrt{x}-2\right)\left(\sqrt{x}+1\right)}+\dfrac{2\left(\sqrt{x}-2\right)}{\left(\sqrt{x}-2\right)\left(\sqrt{x}+1\right)}-\dfrac{\sqrt{x}+1}{\left(\sqrt{x}-2\right)\left(\sqrt{x}+1\right)}\)
\(X=\dfrac{3+2\sqrt{x}-4-\sqrt{x}-1}{\left(\sqrt{x}-2\right)\left(\sqrt{x}+1\right)}\)
\(X=\dfrac{\sqrt{x}-2}{\left(\sqrt{x}-2\right)\left(\sqrt{x}+1\right)}\)
\(X=\dfrac{1}{\sqrt{x}+1}\)
\(S=\left(\dfrac{1}{x+2\sqrt{x}}+\dfrac{1}{\sqrt{x}-2}\right):\left(\dfrac{1-\sqrt{x}}{x+4\sqrt{x}+4}\right)\left(đk:x\ge0;x\ne1\right)\)
\(S=\left(\dfrac{\sqrt{x}-2}{\sqrt{x}\left(\sqrt{x}+2\right)\left(\sqrt{x}-2\right)}+\dfrac{\sqrt{x}\left(\sqrt{x}+2\right)}{\sqrt{x}\left(\sqrt{x}+2\right)\left(\sqrt{x}-2\right)}\right):\left(\dfrac{1-\sqrt{x}}{x+4\sqrt{x}+4}\right)\)
\(S=\dfrac{\sqrt{x}-2+x+2\sqrt{x}}{\sqrt{x}\left(\sqrt{x}+2\right)\left(\sqrt{x}-2\right)}.\dfrac{x+4\sqrt{x}+4}{1-\sqrt{x}}\)
\(S=\dfrac{x+3\sqrt{x}-2}{\sqrt{x}\left(\sqrt{x}+2\right)\left(\sqrt{x}-2\right)}.\dfrac{\left(\sqrt{x}+2\right)^2}{1-\sqrt{x}}\)
\(S=\dfrac{\left(x+3\sqrt{x}-2\right)\left(\sqrt{x}+2\right)}{\sqrt{x}\left(\sqrt{x}-2\right)\left(1-\sqrt{x}\right)}\)
(đến đoạn này thì trong ngoặc ko tách ra đc nữa nên mik nghĩ là đến đây là xong, nếu sai thì bn nói mik)

\(A=\left(\dfrac{\sqrt{x}-2}{x-1}-\dfrac{\sqrt{x}+2}{x+2\sqrt{x}+1}\right)\cdot\dfrac{\left(x-1\right)^2}{2}\)
\(=\dfrac{x-\sqrt{x}-2-\sqrt{x}-\sqrt{x}+2}{\left(\sqrt{x}-1\right)^2\cdot\left(\sqrt{x}+1\right)}\cdot\dfrac{\left(x-1\right)^2}{2}\)
\(=-\sqrt{x}\cdot\left(\sqrt{x}+1\right)\)
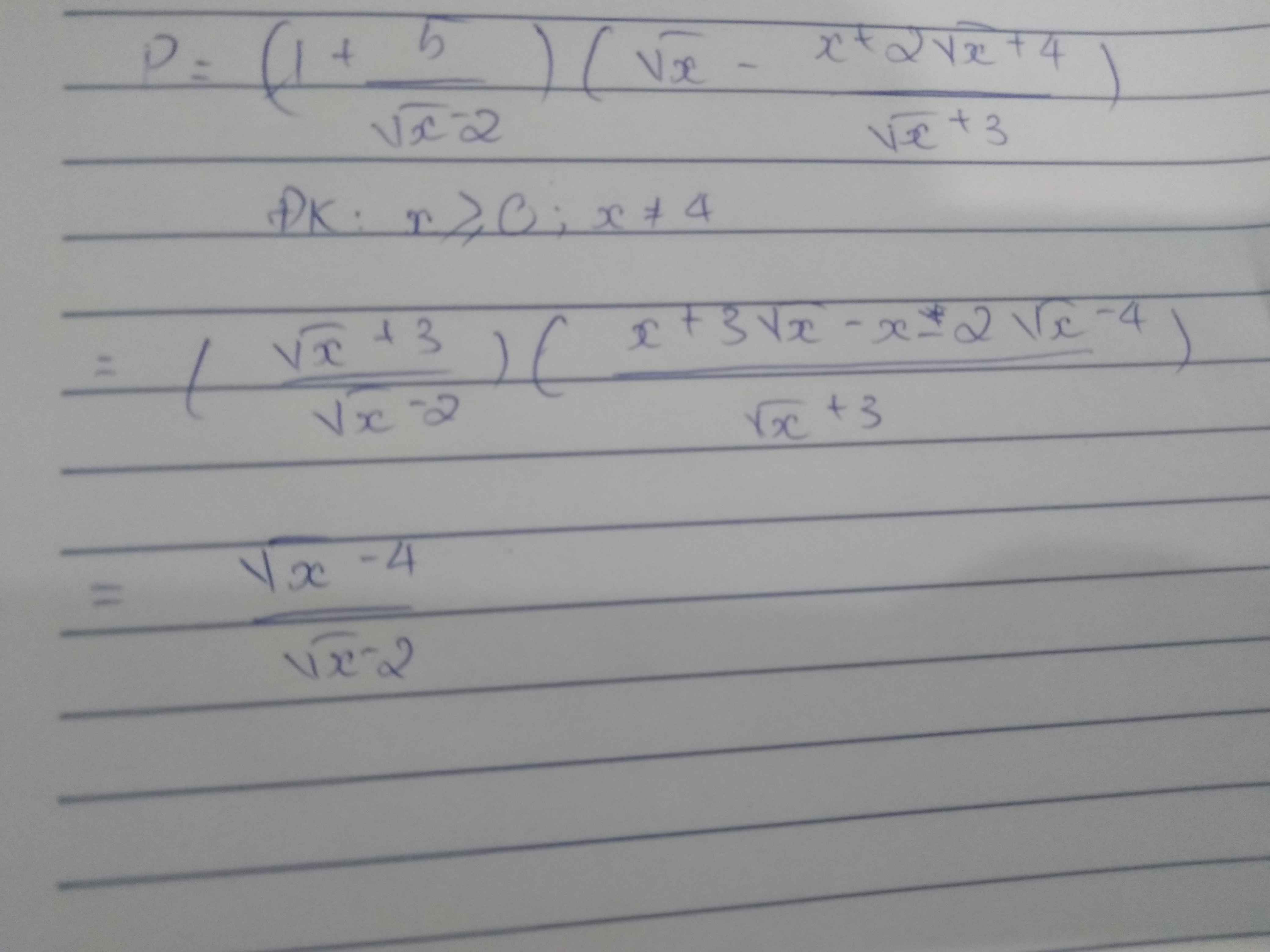

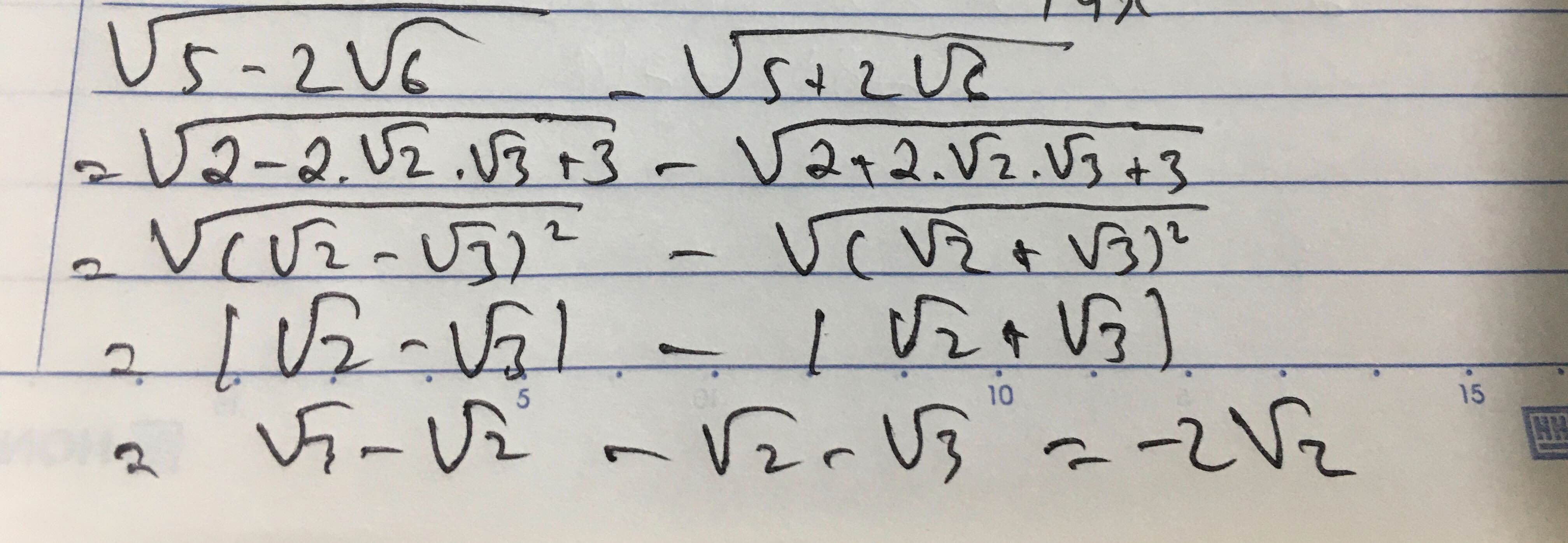
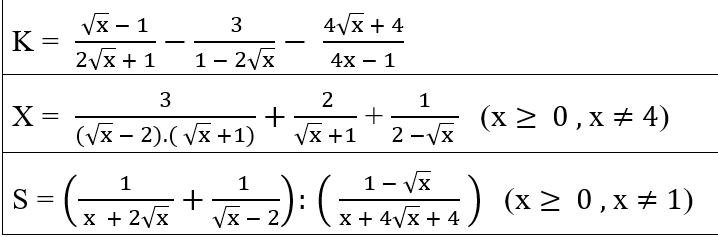
Ta có: \(P=1:\left(\dfrac{x+2}{x\sqrt{x}-1}+\dfrac{\sqrt{x}+1}{x+\sqrt{x}+1}-\dfrac{\sqrt{x}+1}{x-1}\right)\)
\(=1:\left(\dfrac{x+2}{\left(\sqrt{x}-1\right)\left(x+\sqrt{x}+1\right)}+\dfrac{x-1}{\left(\sqrt{x}-1\right)\left(x+\sqrt{x}+1\right)}-\dfrac{x+\sqrt{x}+1}{\left(\sqrt{x}-1\right)\left(x+\sqrt{x}+1\right)}\right)\)
\(=1:\dfrac{x+2+x-1-x-\sqrt{x}-1}{\left(\sqrt{x}-1\right)\left(x+\sqrt{x}+1\right)}\)
\(=1:\dfrac{x-\sqrt{x}}{\left(\sqrt{x}-1\right)\left(x+\sqrt{x}+1\right)}\)
\(=\dfrac{\left(\sqrt{x}-1\right)\left(x+\sqrt{x}+1\right)}{\sqrt{x}\left(\sqrt{x}-1\right)}\)
\(=\dfrac{x+\sqrt{x}+1}{\sqrt{x}}\)