Hãy nhập câu hỏi của bạn vào đây, nếu là tài khoản VIP, bạn sẽ được ưu tiên trả lời.

Bài 1:
a) Ta có: \(P\left(x\right)=3x^4+2x^2-3x^4-2x^2+2x-5\)
\(=\left(3x^4-3x^4\right)+\left(2x^2-2x^2\right)+2x-5\)
\(=2x-5\)
Bài 1:
b)
\(P\left(-1\right)=2\cdot\left(-1\right)-5=-2-5=-7\)
\(P\left(3\right)=2\cdot3-5=6-5=1\)

\(f\left(x\right)=x^3-2x^2+3x+2\)
\(g\left(x\right)=-x^3-3x^2+2\)

`a,`
`F(x)=4x^4-2+2x^3+2x^4-5x+4x^3-9`
`F(x)=(2x^4+4x^4)+(2x^3+4x^3)-5x+(-2-9)`
`F(x)=6x^4+6x^3-5x-11`
`b,`
`K(x)=F(x)+G(x)`
`K(x)=(6x^4+6x^3-5x-11)+(6x^4+6x^3-x^2-5x-27)`
`K(x)=6x^4+6x^3-5x-11+6x^4+6x^3-x^2-5x-27`
`K(x)=(6x^4+6x^4)+(6x^3+6x^3)-x^2+(-5x-5x)+(-11-27)`
`K(x)=12x^4+12x^3-x^2-10x-38`
`c,`
`H(x)=F(x)-G(x)`
`H(x)=(6x^4+6x^3-5x-11)-(6x^4+6x^3-x^2-5x-27)`
`H(x)=6x^4+6x^3-5x-11-6x^4-6x^3+x^2+5x+27`
`H(x)=(6x^4-6x^4)+(6x^3-6x^3)+x^2+(-5x+5x)+(-11+27)`
`H(x)=x^2+16`
Đặt `x^2+16=0`
Ta có: \(x^2\ge0\text{ }\forall\text{ }x\)
`->`\(x^2+16\ge16>0\text{ }\forall\text{ }x\)
`->` Đa thức `H(x)` vô nghiệm.
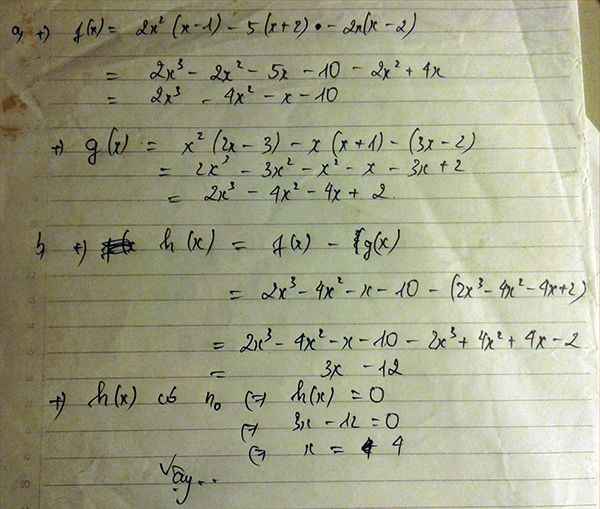
a) Ta có:+) f(x) = 2x2(x - 1) - 5(x - 2) - 2x(x - 2)
f(x) = 2x3 - 2x2 - 5x + 10 - 2x2 + 2x
f(x) = 2x3 - 4x2 - 3x + 10
f(x) = 2x3 - 2x2 - 5x + 10
+) g(x) = x2(2x - 3) - x(x + 1) - (3x - 2)
g(x) = 2x3 - 3x2 - x2 - x - 3x + 2
g(x) = 2x3 - 4x2 - 4x + 2
b) f(2) = 2.23 - 4. 22 - 3.2 + 10 = 16 - 16 - 6 + 10 = 4
g(-2) = 2.(-2)3 - 4.(-2)2 - 4.(-2) + 2 = 2 . 8 - 4.4 + 8 + 2 = 10
c) H(x) = f(x) - g(x) = (2x3 - 4x2 - 3x + 10) - (2x3 - 4x2 - 4x + 2)
H(x) = 2x3 - 4x2 - 3x + 10 - 2x3 + 4x2 + 4x - 2
H(x) = (2x3 - 2x3) - (4x2 - 4x2) - (3x - 4x) + (10 - 2)
H(x) = x + 8
=> f(x) - g(x) = A(x) = -x - 8
d) Ta có: H(x) = 0
=> x + 8 = 0
=> x = -8
deo tra loi