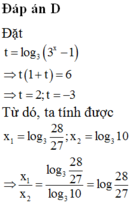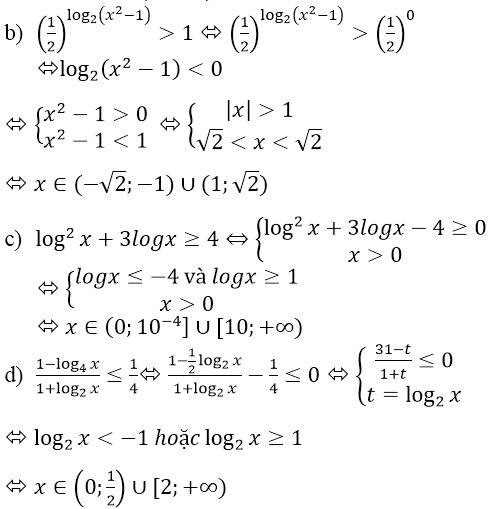Hãy nhập câu hỏi của bạn vào đây, nếu là tài khoản VIP, bạn sẽ được ưu tiên trả lời.

Câu 1:
\(y=x^3-3x^2-2\Rightarrow y'=3x^2-6x\)
Gọi hoành độ của M là \(x_M\)
Hệ số góc của tiếp tuyến của đồ thị (C) tại M bằng 9 tương đương với:
\(f'(x_M)=3x_M^2-6x_M=9\)
\(\Leftrightarrow x_M=3\) hoặc $x_M=-1$
\(\Rightarrow y_M=-2\) hoặc \(y_M=-6\)
Vậy tiếp điểm có tọa độ (3;-2) hoặc (-1;-6)
Đáp án B
Câu 2:
Gọi hoành độ tiếp điểm là $x_0$
Hệ số góc của tiếp tuyến tại tiếp điểm là:
\(f'(x_0)=x_0^2-4x_0+3\)
Vì tt song song với \(y=3x-\frac{20}{3}\Rightarrow f'(x_0)=3\)
\(\Leftrightarrow x_0^2-4x_0+3=3\Leftrightarrow x_0=0; 4\)
Khi đó: PTTT là:
\(\left[{}\begin{matrix}y=3\left(x-0\right)+f\left(0\right)=3x+4\\y=3\left(x-4\right)+f\left(4\right)=3x-\dfrac{20}{3}\end{matrix}\right.\) (đt 2 loại vì trùng )
Do đó \(y=3x+4\Rightarrow \) đáp án A
Câu 3:
PT hoành độ giao điểm:
\(\frac{2x+1}{x-1}-(-x+m)=0\)
\(\Leftrightarrow x^2+(1-m)x+(m+1)=0\) (1)
Để 2 ĐTHS cắt nhau tại hai điểm pb thì (1) phải có hai nghiệm phân biệt
\(\Leftrightarrow \Delta=(1-m)^2-4(m+1)> 0\)
\(\Leftrightarrow m^2-6m-3> 0\)
\(\Leftrightarrow\left[{}\begin{matrix}m< 3-2\sqrt{3}\\m>3+2\sqrt{3}\end{matrix}\right.\)
Kết hợp với m nguyên và \(m\in (0;10)\Rightarrow m=7;8;9\)
Có 3 giá trị m thỏa mãn.

a)
Có:
\(log_2^{\left(2^x+1\right)}.log_2^{\left(2^{x+1}+2\right)}=2\)
\(\Leftrightarrow log_2^{\left(2^x+1\right)}.\left[1+log_2^{\left(2^{x+1}\right)}\right]=2\)
Đặt \(t=log_2^{\left(2^x+1\right)}\), ta có phương trình \(t\left(1+t\right)=2\Leftrightarrow t^2+t-2=0\)
\(\Leftrightarrow\left[{}\begin{matrix}t=1\\t=-2\end{matrix}\right.\Leftrightarrow\left[{}\begin{matrix}log_2^{\left(2^x+1\right)}=1\\log_2^{\left(2x+1\right)}=-2\end{matrix}\right.\)
\(\Leftrightarrow\left[{}\begin{matrix}2^x+1=2\\2^x+1=\dfrac{1}{4}\end{matrix}\right.\Leftrightarrow\left[{}\begin{matrix}2^x=1\\2^x=-\dfrac{3}{4}\left(không-t.m\right)\end{matrix}\right.\Leftrightarrow x=0\)
b)
Với điều kiện \(x>0\), ta có:
\(log.\left(x^{log9}\right)=log9.logx\) và \(log\left(9^{logx}=logx.log9\right)\)
nên \(log\left(x^{log9}\right)=log\left(9^{logx}\right)\)
\(\Rightarrow x^{log9}=9^{logx}\)
Đặt \(t=x^{log9}\), ta được phương trình \(2t=6\Leftrightarrow t=3\Leftrightarrow x^{log9}=3\)
\(\Leftrightarrow log\left(x^{log9}\right)=log3\Leftrightarrow log9.logx=log3\)
\(\Leftrightarrow logx=\dfrac{log3}{log9}\Leftrightarrow x=\dfrac{1}{2}\)
\(\Leftrightarrow x=\sqrt{10}\) (thỏa mãn điều kiện \(x>0\)).
c)
Với điều kiện \(x>0\), lấy lôgarit thập phân hai vế của phương trình đã cho, ta được:
\(\left(3log^3x-\dfrac{2}{3}logx\right).logx=\dfrac{7}{3}\)
Đặt \(t=logx\), ta được phương trình:
\(3t^4-\dfrac{2}{3}t^2-\dfrac{7}{3}=0\)
\(\Leftrightarrow9t^4-2t^2-7=0\Leftrightarrow\left[{}\begin{matrix}t^2=1\\t^2=-\dfrac{7}{9}\left(không-t.m\right)\end{matrix}\right.\Leftrightarrow\left[{}\begin{matrix}t=1\\t=-1\end{matrix}\right.\)
\(\Leftrightarrow\left[{}\begin{matrix}logx=1\\logx=-1\end{matrix}\right.\Leftrightarrow\left[{}\begin{matrix}x=10\\x=\dfrac{1}{10}\end{matrix}\right.\)
d)
Đặt \(t=log_5^{\left(x+2\right)}\) với điều kiện \(x+2>0\), \(x+2\ne1\), ta có:
\(1+\dfrac{2}{t}=t\Leftrightarrow t^2-t-2=0,t\ne0\)
\(\Leftrightarrow\left[{}\begin{matrix}t=-1\\t=2\end{matrix}\right.\Leftrightarrow\left[{}\begin{matrix}log_5^{\left(x+2\right)}=-1\\log_5^{\left(x+2\right)}=2\end{matrix}\right.\Leftrightarrow\left[{}\begin{matrix}x+2=\dfrac{1}{5}\\x+2=25\end{matrix}\right.\Leftrightarrow\left[{}\begin{matrix}x=-\dfrac{9}{5}\\x=23\end{matrix}\right.\)