
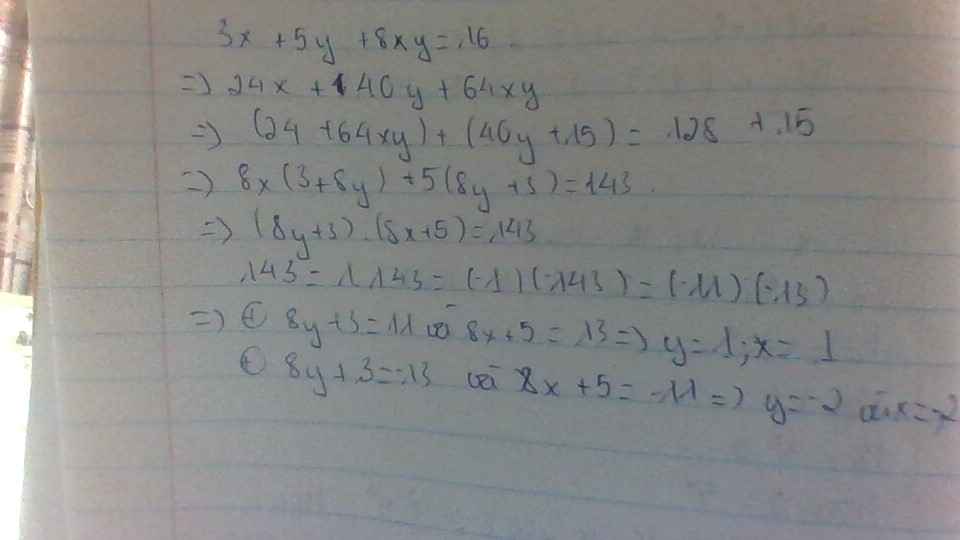
Hãy nhập câu hỏi của bạn vào đây, nếu là tài khoản VIP, bạn sẽ được ưu tiên trả lời.

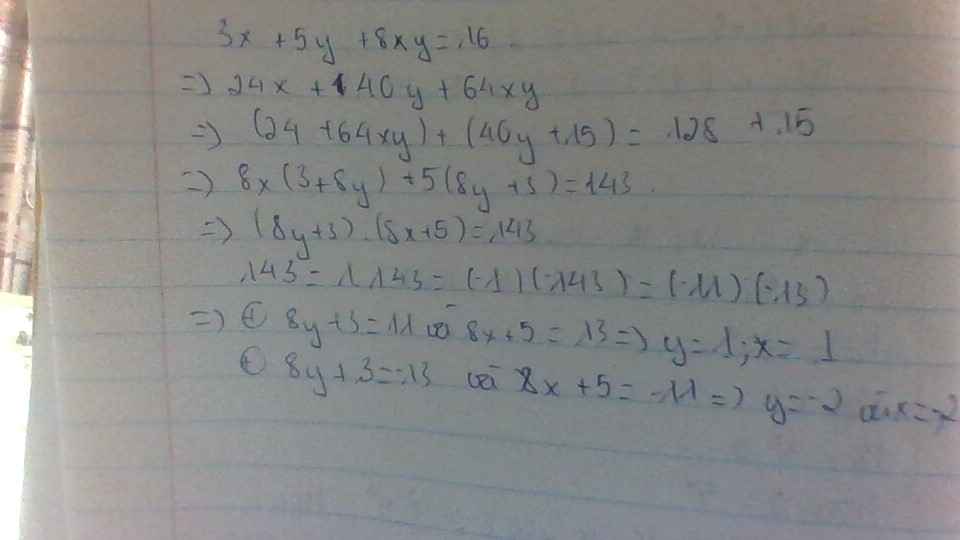

a, 3x ( y+1) + y + 1 = 7
(y+1)(3x +1) =7
th1 : \(\left\{{}\begin{matrix}y+1=1\\3x+1=7\end{matrix}\right.\) => \(\left\{{}\begin{matrix}y=0\\x=2\end{matrix}\right.\)
th2: \(\left\{{}\begin{matrix}y+1=-1\\3x+1=-7\end{matrix}\right.\)=> x = -8/3 (loại)
th3: \(\left\{{}\begin{matrix}y+1=7\\3x+1=1\end{matrix}\right.\)=> \(\left\{{}\begin{matrix}y=6\\x=0\end{matrix}\right.\)
th 4 : \(\left\{{}\begin{matrix}y+1=-7\\3x+1=-1\end{matrix}\right.\)=> x=-2/3 (loại)
Vậy (x,y)= (2 ;0); (0; 6)
b, xy - x + 3y - 3 = 5
(x( y-1) + 3( y-1) = 5
(y-1)(x+3) = 5
th1: \(\left\{{}\begin{matrix}y-1=1\\x+3=5\end{matrix}\right.\) => \(\left\{{}\begin{matrix}y=2\\x=8\end{matrix}\right.\)
th2: \(\left\{{}\begin{matrix}y-1=-1\\x+3=-5\end{matrix}\right.\) => \(\left\{{}\begin{matrix}y=0\\x=-8\end{matrix}\right.\)
th3: \(\left\{{}\begin{matrix}y-1=5\\x+3=1\end{matrix}\right.\) => \(\left\{{}\begin{matrix}y=6\\x=-2\end{matrix}\right.\)
th4: \(\left\{{}\begin{matrix}y-1=-5\\x+3=-1\end{matrix}\right.\) => \(\left\{{}\begin{matrix}y=-4\\x=-4\end{matrix}\right.\)
vậy (x, y) = ( 8; 2); ( -8; 0); (-2; 6); (-4; -4)
c, 2xy + x + y = 7 => y = \(\dfrac{7-x}{2x+1}\) ; y ϵ Z ⇔ 7-x ⋮ 2x+1
⇔ 14 - 2x ⋮ 2x + 1 ⇔ 15 - 2x - 1 ⋮ 2x + 1
th1 : 2x + 1 = -1=> x = -1; y = \(\dfrac{7-(-1)}{-1.2+1}\) = -8
th2: 2x+ 1 = 1=> x =0; y = 7
th3: 2x+1 = -3 => x = x=-2 => y = \(\dfrac{7-(-2)}{-2.2+1}\) = -3
th4: 2x+ 1 = 3 => x = 1 => y = \(\dfrac{7+1}{2.1+1}\) = 2
th5: 2x + 1 = -5 => x = -3=> y = \(\dfrac{7-(-3)}{-3.2+1}\) = -2
th6: 2x + 1 = 5 => x = 2; ; y = \(\dfrac{7-2}{2.2+1}\) =1
th7 : 2x + 1 = -15 => x = -8; y = \(\dfrac{7-(-8)}{-8.2+1}\) = -1
th8 : 2x+1 = 15 => x = 7; y = \(\dfrac{7-7}{2.7+1}\) = 0
kết luận
(x,y) = (-1; -8); (0 ;7); ( -2; -3) ; ( 1; 2); ( -3; -2); (2;1); (-8;-1);(7;0)
3xy−2x+5y=293xy−2x+5y=29
9xy−6x+15y=879xy−6x+15y=87
(9xy−6x)+(15y−10)=77(9xy−6x)+(15y−10)=77
3x(3y−2)+5(3y−2)=773x(3y−2)+5(3y−2)=77
(3y−2)(3x+5)=77(3y−2)(3x+5)=77
⇒(3y−2)⇒(3y−2) và (3x+5)(3x+5) là Ư(77)=±1,±7,±11,±77Ư(77)=±1,±7,±11,±77
Ta có bảng giá trị sau:
Do x,y∈Zx,y∈Z nên (x,y)∈{(−4;−3),(−2;−25),(2;3),(24;1)}

a: =>x-xy+y=0
=>x(1-y)+1-y-1=0
=>(x+1)(1-y)=1
=>(x+1)(y-1)=-1
=>\(\left(x+1;y-1\right)\in\left\{\left(-1;1\right);\left(1;-1\right)\right\}\)
=>\(\left(x,y\right)\in\left\{\left(-2;2\right);\left(0;0\right)\right\}\)
b: 2x-xy-2y=3
=>x(2-y)-2y+4=7
=>x(2-y)+2(2-y)=7
=>(x+2)(y-2)=-7
=>\(\left(x+2;y-2\right)\in\left\{\left(1;-7\right);\left(-7;1\right);\left(-1;7\right);\left(7;-1\right)\right\}\)
=>\(\left(x;y\right)\in\left\{\left(-1;-5\right);\left(-9;3\right);\left(-3;9\right);\left(5;1\right)\right\}\)
c: =>x(4-y)+5y-20=-3
=>x(4-y)-5(4-y)=-3
=>(4-y)(x-5)=-3
=>(x-5)(y-4)=3
=>\(\left(x-5;y-4\right)\in\left\{\left(1;3\right);\left(3;1\right);\left(-1;-3\right);\left(-3;-1\right)\right\}\)
=>\(\left(x,y\right)\in\left\{\left(6;9\right);\left(8;5\right);\left(4;1\right);\left(2;3\right)\right\}\)

Bài 1: Tìm x, y nguyên biết :
a) 4x + 2xy + y = 7
=> 2.x(y-2)+(y-2)=5
=> ( y-2)(2x+1)= 5
Ta có bảng sau:
| 2x+1 | -5 | -1 | 1 | 5 |
| y-2 | -1 | -5 | 5 | 1 |
| x | -3 | -1 | 0 | 2 |
| y | 1 | -3 | 7 | 3 |
Điều kiện: t/m
Vậy:....
phần b và c tương tự

\(13x=13\Leftrightarrow x=1\)
\(\left(x-1\right)\left(y+3\right)=-5\)
\(TH1\hept{\begin{cases}x-1=-5\\y+3=1\end{cases}\Rightarrow\hept{\begin{cases}x=-4\\y=-2\end{cases}}}\)
\(TH2\hept{\begin{cases}x-1=5\\y+3=-1\end{cases}\Rightarrow\hept{\begin{cases}x=6\\y=2\end{cases}}}\)
\(2n+1⋮n-3\)
\(2n-6+7⋮n-3\)
\(7⋮n-3\)
\(\Rightarrow n-3\inƯ\left(7\right)=\left\{\pm1;\pm7\right\}\)
Tự lập bảng ....
Tương tự bài tiếp theo nhen
Mấy bài kia chắc c lm đc r nhỉ
2. a) \(2n+1⋮n-3\)
\(\Leftrightarrow2.\left(n-3\right)+7⋮n-3\)
\(\Leftrightarrow7⋮n-3\)
\(\Leftrightarrow n-3\in\left\{-7;-1;1;7\right\}\)
\(\Leftrightarrow n\in\left\{-4;2;4;10\right\}\) ( thỏa mãn n nguyên )
Vậy \(n\in\left\{-4;2;4;10\right\}\)
b) \(3n+8⋮n+1\)
\(\Leftrightarrow3.\left(n+1\right)+5⋮n+1\)
\(\Leftrightarrow5⋮n+1\)
\(\Leftrightarrow n+1\in\left\{-5;-1;1;5\right\}\)
\(\Leftrightarrow n\in\left\{-6;-2;0;4\right\}\) ( thỏa mãn n nguyên )
Vậy \(n\in\left\{-6;-2;0;4\right\}\)
~~~~~~~~~~ Học tốt nha ~~~~~~~~~~~~~~~~~

a) \(\frac{-2}{x}=\frac{y}{5}\Leftrightarrow xy=-10\)
\(\Rightarrow\left[{}\begin{matrix}\left\{{}\begin{matrix}x=-1\\y=10\end{matrix}\right.\\\left\{{}\begin{matrix}x=-2\\y=5\end{matrix}\right.\end{matrix}\right.\) hoặc \(\left[{}\begin{matrix}\left\{{}\begin{matrix}x=-10\\y=1\end{matrix}\right.\\\left\{{}\begin{matrix}x=-5\\y=2\end{matrix}\right.\end{matrix}\right.\)
Vậy : ...
b) \(\frac{3+x}{7+y}=\frac{3}{7}\)
\(\Leftrightarrow21+7x=21+3y\)
\(\Leftrightarrow7x=3y\)
\(\Leftrightarrow\frac{x}{3}=\frac{y}{7}=\frac{x+y}{3+7}=\frac{20}{10}=2\) ( Tính chất dãy tỉ số bằng nhau )
\(\Rightarrow\left\{{}\begin{matrix}x=6\\y=14\end{matrix}\right.\)
Vậy : ....
c) \(3x=5y\Rightarrow\frac{x}{5}=\frac{y}{3}=\frac{x+y}{5+3}=\frac{16}{8}=2\) ( Tính chất dãy tỉ số bằng nhau )
\(\Rightarrow\left\{{}\begin{matrix}x=10\\y=6\end{matrix}\right.\)
Vậy : ...

=>\(\int^{3x-2=1}_{5y-4=1}\Leftrightarrow\int^{x=1}_{y=1}\)
Hoặc\(\int^{3x-2=-1}_{5y-4=-1}\Leftrightarrow\int^{x=\frac{1}{3}}_{y=\frac{3}{5}}\)( loại vì x,y thuộc N)
Vậy x =1 ; y =1
(3x-2)(5y-4)=1
suy ra 3x-2 và 5y-4 là Ư(1)={1,-1}
3x-2=1 và 5y-4=1
3x=3. 5y=5
=> x=1,y=1
3x-2=-1 và 5y-4=-1
3x=1. 5y=3
=> x=1/3,y=3/5
