Hãy nhập câu hỏi của bạn vào đây, nếu là tài khoản VIP, bạn sẽ được ưu tiên trả lời.

\(a,d//d_1\Leftrightarrow\left\{{}\begin{matrix}m+2=-2\\m\ne3\end{matrix}\right.\Leftrightarrow m=-4\\ b,d\perp d_2\Leftrightarrow\dfrac{1}{3}\left(m+2\right)=-1\Leftrightarrow m+2=-3\Leftrightarrow m=-5\\ c,d.qua.N\left(1;3\right)\Leftrightarrow x=1;y=3\Leftrightarrow3=m+2+m\\ \Leftrightarrow2m=1\Leftrightarrow m=\dfrac{1}{2}\)

a: Thay x=2 và y=-3 vào (d), ta được:
\(2\left(2m-1\right)-2m+5=-3\)
=>\(4m-2-2m+5=-3\)
=>2m+3=-3
=>2m=-6
=>\(m=-\dfrac{6}{2}=-3\)
b: Để (d)//(d') thì \(\left\{{}\begin{matrix}2m-1=2\\-2m+5\ne1\end{matrix}\right.\)
=>\(\left\{{}\begin{matrix}2m=3\\-2m\ne-4\end{matrix}\right.\Leftrightarrow\left\{{}\begin{matrix}m=\dfrac{3}{2}\\m\ne2\end{matrix}\right.\)
=>m=3/2
Thay m=3/2 vào (d), ta được:
\(y=\left(2\cdot\dfrac{3}{2}-1\right)x-2\cdot\dfrac{3}{2}+5=2x+2\)
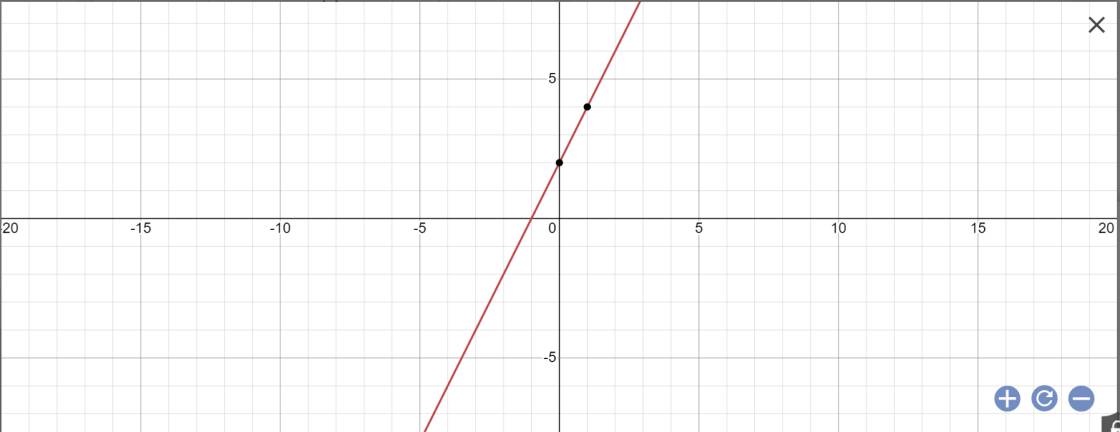
y=2x+2 nên a=2
Gọi \(\alpha\) là góc tạo bởi (d) với trục Ox
\(tan\alpha=2\)
=>\(\alpha\simeq63^026'\)

1.
\(a,\Leftrightarrow2m-1+m-2=6\Leftrightarrow3m=9\Leftrightarrow m=3\\ b,2x+3y-5=0\Leftrightarrow3y=-2x+5\Leftrightarrow y=-\dfrac{2}{3}x+\dfrac{5}{3}\)
Để \(\left(d\right)\text{//}y=-\dfrac{2}{3}x+\dfrac{5}{3}\Leftrightarrow\left\{{}\begin{matrix}2m-1=-\dfrac{2}{3}\\m-2\ne\dfrac{5}{3}\end{matrix}\right.\Leftrightarrow\left\{{}\begin{matrix}m=\dfrac{1}{6}\\m\ne\dfrac{11}{3}\end{matrix}\right.\Leftrightarrow m=\dfrac{1}{6}\)
\(c,x+2y+1=0\Leftrightarrow2y=-x-1\Leftrightarrow y=-\dfrac{1}{2}x-\dfrac{1}{2}\\ \left(d\right)\bot y=-\dfrac{1}{2}x-\dfrac{1}{2}\Leftrightarrow\left(-\dfrac{1}{2}\right)\left(2m-1\right)=-1\\ \Leftrightarrow\dfrac{1}{2}\left(2m-1\right)=1\Leftrightarrow m-\dfrac{1}{2}=1\Leftrightarrow m=\dfrac{3}{2}\)
2.
Gọi điểm cố định đó là \(A\left(x_0;y_0\right)\)
\(\Leftrightarrow y_0=\left(2m-1\right)x_0+m-2\\ \Leftrightarrow2mx_0+m-x_0-2-y_0=0\\ \Leftrightarrow m\left(2x_0+1\right)-\left(x_0+y_0+2\right)=0\\ \Leftrightarrow\left\{{}\begin{matrix}2x_0=-1\\x_0+y_0+2=0\end{matrix}\right.\Leftrightarrow\left\{{}\begin{matrix}x_0=-\dfrac{1}{2}\\y_0=-\dfrac{3}{2}\end{matrix}\right.\)

a: Để (d)//(d1) thì \(\left\{{}\begin{matrix}m^2+2m=-1\\m+1\ne-2023\end{matrix}\right.\)
=>\(\left\{{}\begin{matrix}m^2+2m+1=0\\m\ne-2024\end{matrix}\right.\)
=>\(\left\{{}\begin{matrix}\left(m+1\right)^2=0\\m\ne-2024\end{matrix}\right.\)
=>(m+1)2=0
=>m+1=0
=>m=-1
b: Thay x=0 và y=2024 vào (d), ta được:
\(0\left(m^2+2m\right)+m+1=2024\)
=>m+1=2024
=>m=2023
c: Tọa độ giao điểm của (d2) và (d3) là:
\(\left\{{}\begin{matrix}x-2=-4x+3\\y=x-2\end{matrix}\right.\)
=>\(\left\{{}\begin{matrix}5x=5\\y=x-2\end{matrix}\right.\Leftrightarrow\left\{{}\begin{matrix}x=1\\y=1-2=-1\end{matrix}\right.\)
Thay x=1 và y=-1 vào (d), ta được:
\(1\left(m^2+2m\right)+m+1=-1\)
=>\(m^2+3m+2=0\)
=>(m+2)(m+1)=0
=>\(\left[{}\begin{matrix}m+2=0\\m+1=0\end{matrix}\right.\Leftrightarrow\left[{}\begin{matrix}m=-2\\m=-1\end{matrix}\right.\)

gọi (d): y=(m-1)x+2n ; (d'): y=x-2
điều kiện để (d) là hsbn: m khác 1
điều kiện để (d) // (d'): {\(\hept{\begin{cases}m-1=1==>m=2\\2nkhác-2==>nkhác-1\end{cases}}\)
thay m=2 vào (d) ta có y=x+2n
do (d) đi qua (1;4)=> 4=1+2n => n=3/2
vậy với m=2, n=3/2 thì thỏa mãn đề bài

a: Thay x=1 và y=2 vào (d), ta được:
2m+1=2
hay m=1/2
b: Để (d)//(d') thì m+3=4
hay m=1