
Hãy nhập câu hỏi của bạn vào đây, nếu là tài khoản VIP, bạn sẽ được ưu tiên trả lời.


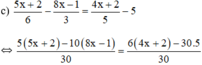
⇔ 5(5x + 2) – 10(8x – 1) = 6(4x + 2) – 150
⇔ 25x + 10 – 80x + 10 = 24x + 12 – 150
⇔ 20 – 55x = 24x – 138
⇔ -55x – 24x = -138 – 20
⇔ -79x = -158
⇔ x = 2.
Vậy phương trình có tập nghiệm S = {2}.

a) 6x - 3 = 8x + 9
6x = 8x + 9 + 3
6x = 8x + 12
6x - 6x = 8x - 6x + 12
0 = 2x + 12
0 - 12 = 2x
-12 = 2x
2x = -12
x = -12 : 2
x = -6
b) 7x - 5 = 13 - 5x
7x = 13 - 5x + 5
7x = 13 + 5 - 5x
7x = 18 - 5x
7x + 5x = 18 - 5x + 5x
12x = 18 - (5x - 5x)
12x = 18
12x = 18
x = 18 : 12
x = \(\frac{3}{2}\)(hoặc = 1,5)
c) 2 - 3x = 5x + 10
3x = 2 - (5x + 10)
3x = 2 - 5x - 10
3x = 2 - 10 - 5x
3x = -8 - 5x
3x - 3x = -8 - 5x + 3x
0 = -8 - (5x + 3x)
0 = -8 - 8x
-8 - 8x = 0
8x = -8 - 0
8x = -8
x = -8 : 8
x = -1

\(5x^2+8x-13=0\)
\(\Rightarrow5x\left(x-1\right)+13\left(x-1\right)=0\)
\(\Rightarrow\left(5x+13\right)\left(x-1\right)=0\)
\(\Rightarrow\left[{}\begin{matrix}5x+13=0\\x-1=0\end{matrix}\right.\Rightarrow\left[{}\begin{matrix}x=-\dfrac{13}{5}\\x=1\end{matrix}\right.\).
\(5x^2+8x-13=0\Leftrightarrow5x^2-5x+13x-13=0\Leftrightarrow\left(5x^2-5x\right)+\left(13x-13\right)=0\Leftrightarrow5x\left(x-1\right)+13\left(x-1\right)=0\Leftrightarrow\left(x-1\right)\left(5x+13\right)=0\Leftrightarrow\left\{{}\begin{matrix}x-1=0\\5x+13=0\end{matrix}\right.\Leftrightarrow\left\{{}\begin{matrix}x=1\\x=\dfrac{-13}{5}\end{matrix}\right.\)


\(B=5x^2+y^2-4xy-6x+13\)
\(=\left(4x^2-4xy+y^2\right)+\left(x^2-6x+9\right)+4\)
\(=\left(2x-y\right)^2+\left(x-3\right)^2+4\ge4\)
Dấu "=" xảy ra \(\Leftrightarrow\left\{{}\begin{matrix}2x-y=0\\x-3=0\end{matrix}\right.\Leftrightarrow\left\{{}\begin{matrix}x=3\\y=6\end{matrix}\right.\)
Vậy \(B_{min}=4\Leftrightarrow\left\{{}\begin{matrix}x=3\\y=6\end{matrix}\right.\)
\(C=9x^2+y^2-2xy-8x+10\)
\(=\left(x^2-2xy+y^2\right)+\left(4x^2-4x+1\right)+\left(4x^2-4x+1\right)+8\)
\(=\left(x-y\right)^2+\left(2x-1\right)^2+\left(2x-1\right)^2+8\ge8\)
Dấu "=" xảy ra \(\Leftrightarrow\left\{{}\begin{matrix}x-y=0\\2x-1=0\end{matrix}\right.\) \(\Leftrightarrow x=y=\frac{1}{2}\)
Vậy \(C_{max}=8\Leftrightarrow x=y=\frac{1}{2}\)



a) Ta có: \(x\left(x-3xy\right)-\frac{3}{5}y\left(4y-5x^2\right)\)
\(=x^2-3x^2y-\frac{12}{5}y^2+3x^2y\)
\(=x^2-\frac{12}{5}y^2\)(1)
Thay x=-2 và \(y=-\frac{1}{2}\) vào biểu thức (1), ta được:
\(\left(-2\right)^2-\frac{12}{5}\cdot\left(-\frac{1}{2}\right)^2\)
\(=4-\frac{12}{5}\cdot\frac{1}{4}\)
\(=4-\frac{3}{5}=\frac{17}{5}\)
Vậy: Giá trị của biểu thức \(x\left(x-3xy\right)-\frac{3}{5}y\left(4y-5x^2\right)\) tại x=-2 và \(y=-\frac{1}{2}\) là \(\frac{17}{5}\)
b) Ta có: x=7
nên 8=x+1
Thay 8=x+1 vào biểu thức \(x^{15}-8x^{14}+8x^{13}-8x^{12}+...-8x^2+8x-5\), ta được:
\(x^{15}-x^{14}\cdot\left(x+1\right)+x^{13}\cdot\left(x+1\right)-x^{12}\cdot\left(x+1\right)+...-x^2\cdot\left(x+1\right)+x\left(x+1\right)-5\)
\(=x^{15}-x^{15}-x^{14}+x^{14}+x^{13}-x^{13}-x^{12}+x^{12}-...-x^3-x^2+x^2+x-5\)
\(=x-5=7-5=2\)
Vậy: Giá trị của biểu thức \(x^{15}-8x^{14}+8x^{13}-8x^{12}+...-8x^2+8x-5\) tại x=7 là 2

a) \(2\left(x+1\right)-1=3-\left(1-2x\right)\)
\(\Leftrightarrow2x+2-1=3-1+2x\)
\(\Leftrightarrow2x-2x=-2+1+3-1\)
\(\Leftrightarrow0x=1\)(vô lí)
Vậy tập nghiệm của phương trình trên bằng \(S=\varnothing\)
b)\(\left(5x-1\right)^2-x^2-8x-16=0\)
\(\Leftrightarrow\left(5x-1\right)^2-\left(x^2+8x+16\right)=0\)
\(\Leftrightarrow\left(5x-1\right)^2-\left(x+4\right)^2=0\)
\(\Leftrightarrow\left(5x-1-x-4\right)\left(5x-1+x+4\right)=0\)
\(\Leftrightarrow\left(4x-5\right)\left(6x+3\right)=0\)
\(\Leftrightarrow\hept{\begin{cases}4x-5=0\\6x+3=0\end{cases}\Leftrightarrow\hept{\begin{cases}x=\frac{5}{4}\\x=-\frac{1}{2}\end{cases}}}\)
Vậy tập nghiệm của phương trình trên bằng\(S=\left\{\frac{5}{4};-\frac{1}{2}\right\}\)
#hoktot<3#
x=2
\(5x+13=8x+7\)
\(\Leftrightarrow5x-8x=7-13\)
\(\Leftrightarrow-3x=-6\)
\(\Leftrightarrow x=2\)