Hãy nhập câu hỏi của bạn vào đây, nếu là tài khoản VIP, bạn sẽ được ưu tiên trả lời.

b: Phương trình hoành độ giao điểm là:
x+1=-x+3
\(\Leftrightarrow2x=2\)
\(\Leftrightarrow x=1\)
hay y=2

a, Phương trình hoành độ giao điểm là \(\dfrac{3}{2}x=3x-3\Leftrightarrow\dfrac{3}{2}x=3\Leftrightarrow x=2\Leftrightarrow y=3\Leftrightarrow A\left(2;3\right)\)
Vậy \(A\left(2;3\right)\) là giao điểm của 2 đt
b, Gọi \(\left(d_3\right):y=ax+b\left(a\ne0\right)\) là đt cần tìm
\(\left(d_3\right)//\left(d_1\right)\Leftrightarrow\left\{{}\begin{matrix}a=\dfrac{3}{2}\\b\ne0\end{matrix}\right.\)
PT giao của d3 với Ox tại hoành độ -6 là \(-6a+b=0\Leftrightarrow b=6\cdot\dfrac{3}{2}=9\)
Vậy \(\left(d_3\right):y=\dfrac{3}{2}x+9\)

\(b,\text{PT hoành độ giao điểm: }-2x+5=x-1\Leftrightarrow x=2\Leftrightarrow y=1\Leftrightarrow A\left(2;1\right)\\ \text{Vậy }A\left(2;1\right)\text{ là giao điểm }\left(d_1\right)\text{ và }\left(d_2\right)\\ c,\text{Gọi }\left(d_3\right):y=ax+b\left(a\ne0\right)\text{ là đt cần tìm}\\ \left(d_3\right)\text{//}\left(d_1\right)\text{ và }M\left(-2;1\right)\in\left(d_3\right)\Leftrightarrow\left\{{}\begin{matrix}a=-2\\b\ne5\\-2a+b=1\end{matrix}\right.\Leftrightarrow\left\{{}\begin{matrix}a=-2\\b=-1\end{matrix}\right.\\ \Leftrightarrow\left(d_3\right):y=-2x-1\)

c: Gọi (d): y=ax+b(a<>0) là phương trình đường thẳng cần tìm
Vì (d)//(d1) nên \(\left\{{}\begin{matrix}a=\dfrac{1}{2}\\b\ne0\end{matrix}\right.\)
Vậy: (d): \(y=\dfrac{1}{2}x\)+b
Thay x=4 và y=5 vào (d), ta được:
\(b+\dfrac{1}{2}\cdot4=5\)
=>b+2=5
=>b=3
Vậy: (d): \(y=\dfrac{1}{2}x+3\)

b:
Goi a1,a2 lần lượt là số đo góc tạo bởi (d1), (d2) với trục Ox
tan a1=1
=>a1=45 độ
tan a2=-1
=>a2=135 độ
c: Tọa độ C là:
x+1=-x+3 và y=x+1
=>x=1 và y=2
d: Thay x=1 và y=2 vào y=mx+m-1, ta được:
m+m-1=2
=>2m-1=2
=>2m=3
=>m=3/2

a: 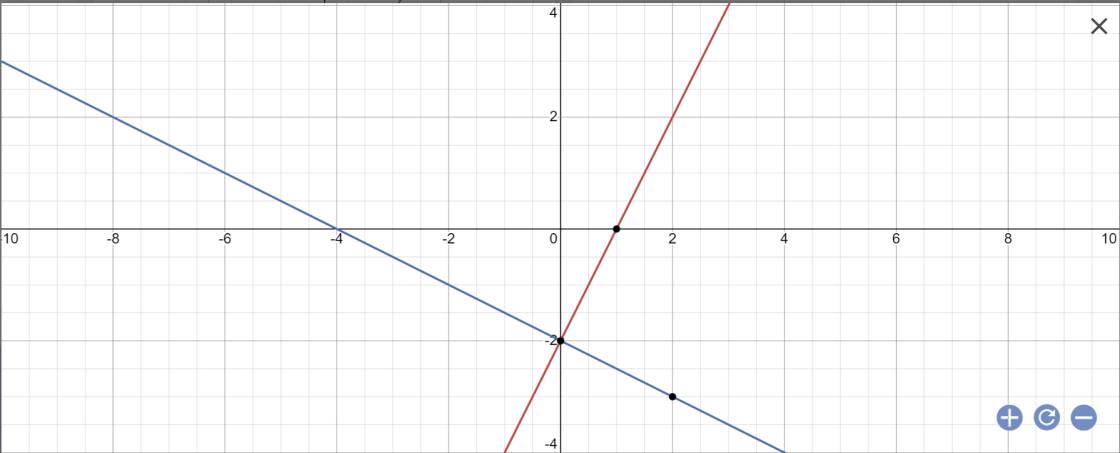
b: Tọa độ A là:
2x-2=-1/2x-2 và y=2x-2
=>x=0 và y=-2
Tọa độ B là:
y=0 và 2x-2=0
=>x=1 và y=0
Tọa độ C là:
y=0 và -1/2x-2=0
=>x=-4; y=0
i: A(0;-2); B(1;0); C(-4;0)
\(\overrightarrow{AB}=\left(1;2\right);\overrightarrow{AC}=\left(-4;2\right)\)
Vì 1*(-4)+2*2=0
nên ΔABC vuông tại A
ii: \(AB=\sqrt{1^2+2^2}=\sqrt{5}\)
\(AC=\sqrt{\left(-4\right)^2+2^2}=2\sqrt{5}\)
\(BC=\sqrt{5+20}=5\left(cm\right)\)
\(C_{ABC}=AB+AC+BC=5+3\sqrt{5}\left(cm\right)\)
\(S_{ABC}=\dfrac{1}{2}\cdot2\sqrt{5}\cdot\sqrt{5}=5\left(cm^2\right)\)
b: Thay x=1 vào (d1), ta được:
y=1-3=-2