Giup mik voi
Hãy nhập câu hỏi của bạn vào đây, nếu là tài khoản VIP, bạn sẽ được ưu tiên trả lời.


a)
\(\left(3^2-2^3\right)x+3^2\cdot2^2=4^2\cdot3\\ \left(9-8\right)x+36=48\\ x=48-36\\ x=12\)
b)
\(\dfrac{x-2}{-4}=\dfrac{-9}{x-2}\left(x\ne2\right)\\\left(x-2\right)^2 =-4\cdot-9\\ \left(x-2\right)^2=36\\ \left(x-2\right)^2=6^2 \)
TH1: x - 2 = 6
x = 8
TH2: x - 2 = -6
x = -4
c)
\(\left(x-1\right)^2+\left(-3\right)^2=5^2\cdot\left(-1\right)^{100}\\ \left(x-1\right)^2+9=25\cdot1\\ \left(x-1\right)^2=25-9=16\\ \left(x-1\right)^2=4^2\)
TH1: x - 1 = 4
x = 5
TH2: x - 1 = -4
x = -3
d) x5-x3=0
⇔ x3(x2-1)=0
⇔ x3(x-1)(x+1)=0
TH1: x=0
TH2: x-1=0 ⇔ x =1
TH3: x + 1=0 ⇔ x =-1
c) x-5 / 3 = -12 /5-x
⇔ x-5 / 3 = 12 / x-5
⇔ (x-5)2= 36
⇔ (x-5)2= 62
TH1: x -5 =6
⇔ x = 11
TH2: x- 5 = -6
⇔ x = -1
f) ⇔ (2x-1)(2x-2)=0
TH1: 2x-1 = 0
⇔ x = 1/2
TH2: 2x-2 = 0
⇔ x=1
g) bài toán có quy luật
⇔ \(\dfrac{2}{6}+\dfrac{2}{12}+\dfrac{2}{20}+...+\dfrac{2}{x\left(x+1\right)}=\dfrac{2023}{2024}\) (nhân 2 cả tử và mẫu)
⇔\(\dfrac{1}{6}+\dfrac{1}{12}+\dfrac{1}{20}+...+\dfrac{1}{x\left(x+1\right)}=\dfrac{2023}{4048}\) ( chia hai vế cho 2 )
⇔\(\dfrac{1}{2.3}+\dfrac{1}{3.4}+\dfrac{1}{4.5}+...+\dfrac{1}{x\left(x+1\right)}=\dfrac{2023}{4048}\)
⇔\(\dfrac{3-2}{2.3}+\dfrac{4-3}{3.4}+\dfrac{5-4}{4.5}+...+\dfrac{\left(x+1\right)-x}{x\left(x+1\right)}=\dfrac{2023}{4048}\)
⇔\(\dfrac{1}{2}-\dfrac{1}{3}+\dfrac{1}{3}-\dfrac{1}{4}+\dfrac{1}{4}-\dfrac{1}{5}+...+\dfrac{1}{x}-\dfrac{1}{x+1}=\dfrac{2023}{4048}\)
⇔\(\dfrac{1}{2}-\dfrac{1}{x+1}=\dfrac{2023}{4048}\) ( rút gọn ta đc)
⇔\(\dfrac{\left(x+1\right)-2}{2.\left(x+1\right)}=\dfrac{2023}{4048}\) ( quy đồng)
⇔ \(\dfrac{x-1}{x+1}=\dfrac{2023}{2024}\) ( nhân 2 vế cho 2 )
⇔ 2024.(x-1)= 2023. ( x+1)
⇔ 2024x -2024 = 2023x + 2023
⇔ 2024x - 2023x= 2023+2024
⇔ x = 4047
tick cho tui nha!

Quãng đường Nam đi được trong 3 giờ đầu là:
\(12\cdot3=36\left(km\right)\)
Quãng đường Nam đi được trong 2 giờ sau là:
\(11\cdot2=22\left(km\right)\)
Quãng đường Nam đi được trong 5 giờ là:
\(36+22=58\left(km\right)\)
Vậy...
Tổng quãng đường mà bạn Nam đi được trong 3 giờ đầu là:
\(3\times12=36\left(km\right)\)
Tổng quãng đường mà bạn Nam đi được trong 2 giờ sau là:
\(2\times11=22\left(km\right)\)
Tổng quãng đường mà bạn Nam đi được trong 5 giờ là:
\(36+22=58\left(km\right)\)
Đáp số : \(58km\)


\(\left(\dfrac{2}{3}\right)^8:\left(\dfrac{4}{9}\right)^3\\ =\left[\left(\dfrac{2}{3}\right)^2\right]^4:\left(\dfrac{4}{9}\right)^3\\ =\left(\dfrac{4}{9}\right)^4:\left(\dfrac{4}{9}\right)^3\\ =\dfrac{4}{9}\)
\(27^3:3^2\\ =\left(3^3\right)^3:3^2\\ =3^9:3^2\\ =3^7\\ =2187\)
\(\left(-\dfrac{3}{5}\right)^4:\left(\dfrac{5}{2}\right)^4\\ =\left(-\dfrac{3}{5}:\dfrac{5}{2}\right)^4\\ =\left(-\dfrac{6}{25}\right)^4\)
\(\left(\dfrac{3}{5}\right)^{12}:\left(\dfrac{9}{25}\right)^5\\ =\left(\dfrac{3}{5}\right)^{12}:\left[\left(\dfrac{3}{5}\right)^2\right]^5\\ =\left(\dfrac{3}{5}\right)^{12}:\left(\dfrac{3}{5}\right)^{10}\\ =\left(\dfrac{3}{5}\right)^2\\ =\dfrac{9}{25}\)
\(\left(\dfrac{2}{3}\right)^8:\left(\dfrac{4}{9}\right)^3=\left(\dfrac{4}{9}\right)^4:\left(\dfrac{4}{9}\right)^3=\dfrac{4}{9}\)
\(27^3:3^2=3^9:3^2=3^7=2187\)

a) Ta có:
\(64^8=\left(2^6\right)^8=2^{6\cdot8}=2^{48}\)
\(16^{12}=\left(2^4\right)^{12}=2^{4\cdot12}=2^{48}\)
\(\Rightarrow64^8=16^{12}\)
b) Ta có:
\(\left(\dfrac{1}{16}\right)^{10}=\left[\left(\dfrac{1}{2}\right)^4\right]^{10}=\left(\dfrac{1}{2}\right)^{4\cdot10}=\left(\dfrac{1}{2}\right)^{40}\)
Mà: 50 > 40 => `(1/2)^50<(1/2)^40`
c) Ta có:
\(\left(\dfrac{9}{16}\right)^{100}=\left[\left(\dfrac{3}{4}\right)^2\right]^{100}=\left(\dfrac{3}{4}\right)^{200}\)
Mà: `3/4>2/3=>(3/4)^200>(2/3)^200`
\(^{^{ }}\)a,64^8=16^12
b,(1/16)^10<(1/2)^50
c,(2/3)^200>(9/16)^100
CỦA BẠN ĐÂY NẾU SAI THÌ CHO MÌNH XIN LỖI NHÉ

a; 25 x 53 x \(\dfrac{1}{625}\) x 52
= 52 x 53 x \(\dfrac{1}{5^4}\) x 52
= 55 x \(\dfrac{1}{5^4}\) x 52
= 5 x 52
= 53
a)
\(25\cdot5^3\cdot\dfrac{1}{625}\cdot5^2\\ =\left(5^2\cdot5^3\cdot5^2\right)\cdot\dfrac{1}{625}\\ =5^7\cdot\dfrac{1}{5^4}\\ =5^3\)
b)
\(5^2\cdot3^5\cdot\left(\dfrac{3}{5}\right)^2\\ =5^2\cdot3^5\cdot\dfrac{3^2}{5^2}\\ =3^5\cdot3^2\\ =3^7\)
c)
\(\left(-\dfrac{1}{7}\right)^4\cdot49^2\\ =\dfrac{\left(-1\right)^4}{7^4}\cdot\left(7^2\right)^2\\ =\dfrac{1}{7^4}\cdot7^4\\ =1\)
d)
\(\left(\dfrac{1}{16}\right)^2:\left(\dfrac{1}{2}\right)^4\cdot\left(-\dfrac{1}{8}\right)^3\\ =\left[\left(\dfrac{1}{2}\right)^4\right]^2:\left(\dfrac{1}{2}\right)^4\cdot\left[\left(-\dfrac{1}{2}\right)^3\right]^3\\ =\left(\dfrac{1}{2}\right)^8:\left(\dfrac{1}{2}\right)^4\cdot\left(-\dfrac{1}{2}\right)^9\\ =\left(\dfrac{1}{2}\right)^4\cdot\left(-\dfrac{1}{2}\right)^9\\ =\left(\dfrac{1}{2}\right)^4\cdot-\left(\dfrac{1}{2}\right)^9\\ =-\left(\dfrac{1}{2}\right)^{13}\)

a; \(x\) : (- \(\dfrac{1}{3}\))3 = \(\dfrac{1}{9}\)
\(x\) : (\(-\dfrac{1}{27}\)) = \(\dfrac{1}{9}\)
\(x\) = \(\dfrac{1}{9}\) x (- \(\dfrac{1}{27}\))
\(x\) = - \(\dfrac{1}{243}\)
Vậy \(x\) = - \(\dfrac{1}{243}\)
b; (\(\dfrac{4}{5}\))5 x \(x\) = (\(\dfrac{4}{5}\))7
\(x\) = (\(\dfrac{4}{5}\))7 : (\(\dfrac{4}{5}\))5
\(x\) = \(\dfrac{4^7}{5^7}\) : \(\dfrac{4^5}{5^5}\)
\(x\) = \(\dfrac{4^7}{5^7}\) x \(\dfrac{5^5}{4^5}\)
\(x\) = \(\dfrac{4^2}{5^2}\)
\(x\) = \(\dfrac{16}{25}\)
Vậy \(x\) = \(\dfrac{16}{25}\)
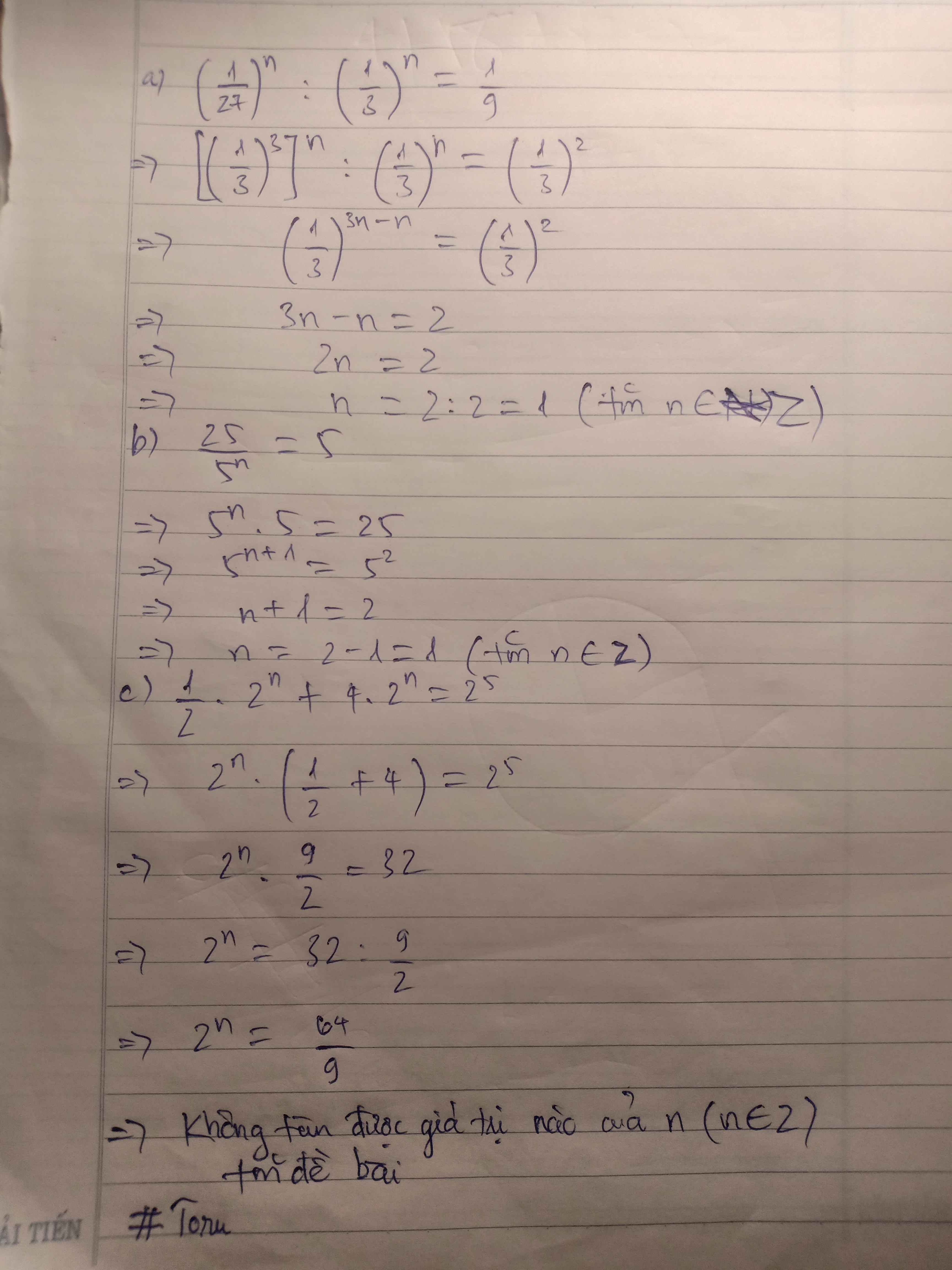
Bài 1:
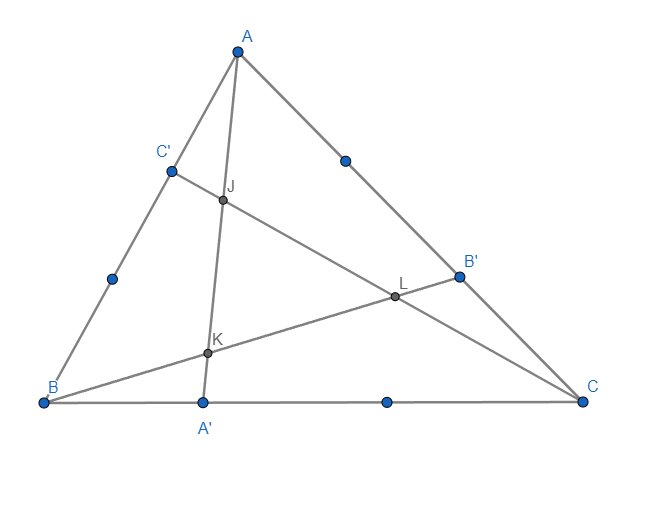
1; Ta có Oy là tia chung của hai góc xOy và yOz
Mặt khác: \(\widehat{xOy}\) + \(\widehat{yOz}\) = 400 + 200 = 600 = \(\widehat{xOz}\)
Vậy tia Oy nằm giữa hai tia Ox và Oz (đúng)
2; Tam giác PQR có các cạnh lần lượt là: PQ; QR; PR
Vậy tam giác PQR là hình gồm ba đoạn PQ; QR; RP đúng
3; Nếu tia Ox nằm giữa hai tia Oy và Oz thì
\(\widehat{yOz}\) = \(\widehat{yOx}\) + \(\widehat{xOz}\)
Vậy \(\widehat{xOy}\) + \(\widehat{zOy}\) = \(\widehat{xOz}\) (sai)
4; Hai góc kề bù là hai góc có một cạnh chung và hai cạnh còn lại là hai tia đối nhau.
Vậy hai góc kề bù là hai góc có hai cạnh là hai tia đối nhau là sai
Bài 2
1; Số nghich đảo của 0,25 là:
1 : 0,25 = 4
Chọn B.4
2; 60% của 55 là: 55 x 60 : 100 = 33
Chọn A.33