Tích phân của (-x²+x+2) cận từ -1 đến 2. Mọi người giải chi tiết giúp em với ạ. Em cảm ơn
Hãy nhập câu hỏi của bạn vào đây, nếu là tài khoản VIP, bạn sẽ được ưu tiên trả lời.


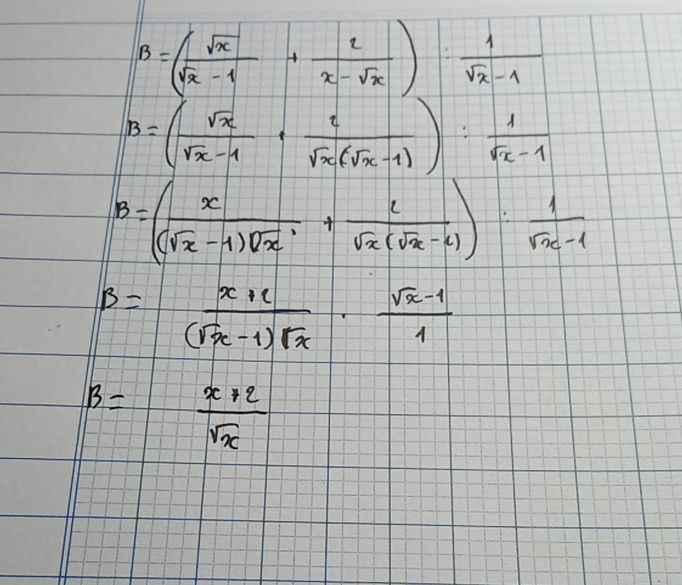
\(=\dfrac{\sqrt{x}+1}{\sqrt{x}\left(\sqrt{x}-1\right)}\cdot\dfrac{\left(\sqrt{x}-1\right)^2}{\sqrt{x}+1}=\dfrac{\sqrt{x}-1}{\sqrt{x}}\)

Tham khảo:https://hoc247.net/hoi-dap/toan-8/phan-tich-da-thuc-x-7-x-2-1-thanh-nhan-tu-faq417522.html
\(=x^7+x^6-x^6+x^5-x^5+x^4-x^4+x^3-x^3+x^2+x^2-x^2+x-x+1\\ =\left(x^7+x^6+x^5\right)-\left(x^6+x^5+x^4\right)+\left(x^4+x^3+x^2\right)-\left(x^3+x^2+x\right)+\left(x^2+x+1\right)\\ =\left(x^2+x+1\right)\left(x^5-x^4+x^2-x+1\right)\)

\(\dfrac{x-3}{3}=4-\dfrac{1-2x}{5}\)
=>5(x-3)=60-3(1-2x)
=>5x-15=60-3+6x
=>5x-15=6x+57
=>6x+57=5x-15
hay x=-72(nhận)

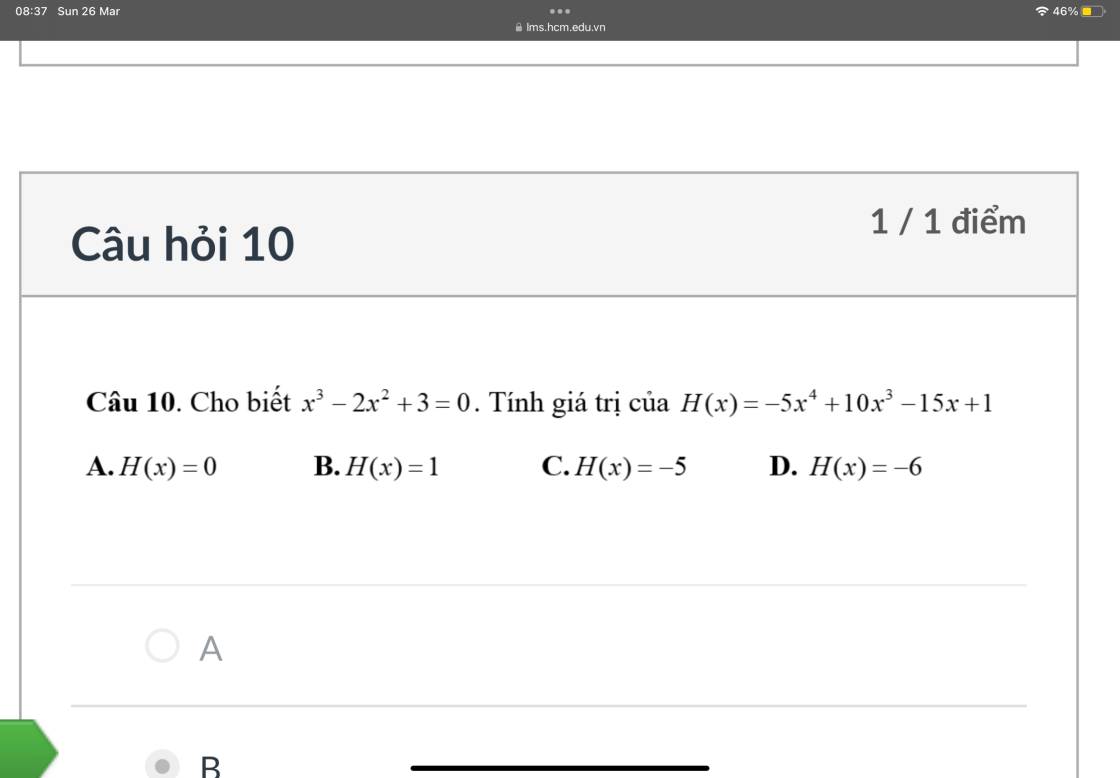
10.
\(H\left(x\right)=-5x^4+10x^3-15x+1\)
\(=-5x\left(x^3-2x^2+3\right)+1\)
\(=-5x.0+1\)
\(=1\)
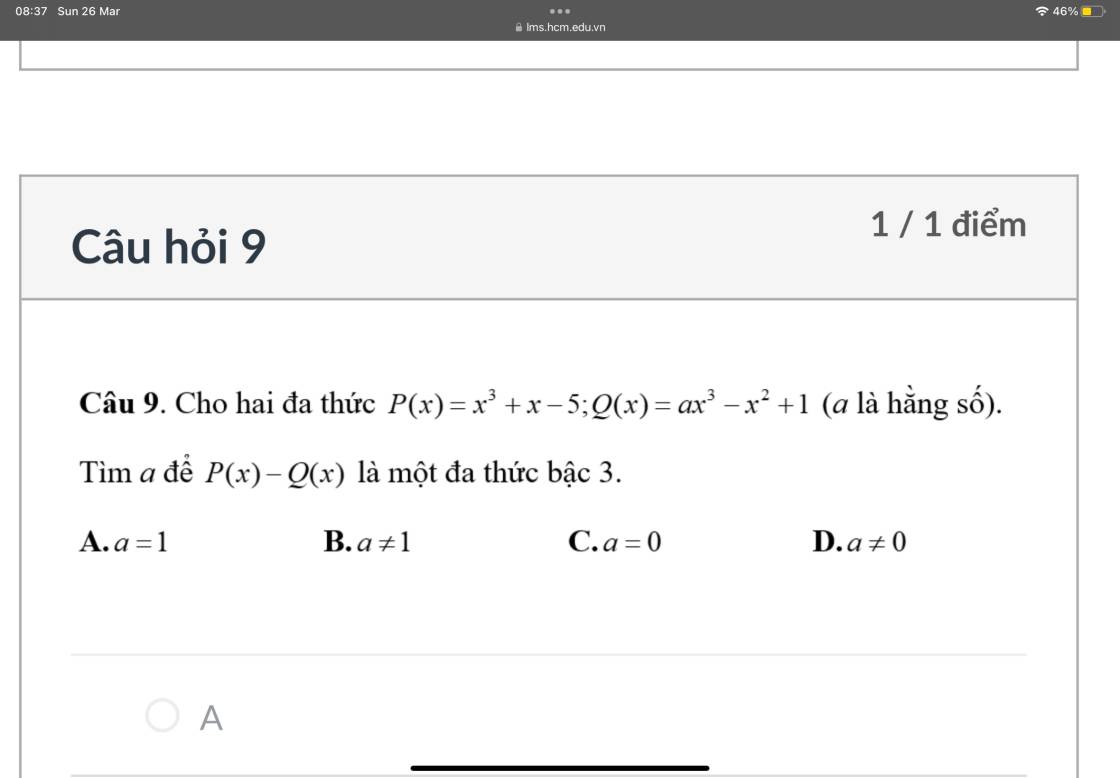
9.
\(P\left(x\right)-Q\left(x\right)=\left(1-a\right)x^3+x^2+x-6\)
\(P\left(x\right)-Q\left(x\right)\) là đa thức bậc 3 khi và chỉ khi \(1-a\ne0\)
\(\Rightarrow a\ne1\)

\(\dfrac{x}{\dfrac{4}{2}}=\dfrac{4}{\dfrac{x}{2}}\)
\(\Rightarrow\dfrac{x}{2}=\dfrac{8}{x}\)
\(\Rightarrow x^2=8\cdot2\)
\(\Rightarrow x^2=16\)
\(\Rightarrow x^2=4^2\)
\(\Rightarrow\left[{}\begin{matrix}x=4\\x=-4\end{matrix}\right.\)
= -2³/3 + 2²/2 + 2.2 - [-(-1)³/3 + (-1)²/2 + 2.(-1)]
= -8/3 + 2 + 4 - 1/3 - 1/2 + 2
= 8 - 3 - 1/2
= 9/2
\(\int\limits^2_{-1}\left(-x^2+x+2\right)dx=\left(-\dfrac{x^3}{3}+\dfrac{x^2}{2}+2x\right)|^2_{-1}=\dfrac{9}{2}\)